Unit 3 Scarcity, work, and choice
Themes and capstone units
How individuals do the best they can, and how they resolve the trade-off between earnings and free time
- Decision making under scarcity is a common problem because we usually have limited means available to meet our objectives.
- Economists model these situations, first by defining all of the feasible actions, then evaluating which of these actions is best, given the objectives.
- Opportunity costs describe the unavoidable trade-offs in the presence of scarcity: satisfying one objective more means satisfying other objectives less.
- A model of decision making under scarcity can be applied to the question of how much time to spend working, when facing a trade-off between more free time and more income.
- This model also helps to explain differences in the hours that people work in different countries, and the changes in our hours of work throughout history.
Imagine that you are working in New York, in a job that is paying you $15 an hour for a 40-hour working week, which gives you earnings of $600 per week. There are 168 hours in a week, so after 40 hours of work, you are left with 128 hours of free time for all your non-work activities, including leisure and sleep.
Suppose, by some happy stroke of luck, you are offered a job at a much higher wage—six times higher. Your new hourly wage is $90. Not only that, your prospective employer lets you choose how many hours you work each week.
Will you carry on working 40 hours per week? If you do, your weekly pay will be six times higher than before: $3,600. Or will you decide that you are satisfied with the goods you can buy with your weekly earnings of $600? You can now earn this by cutting your weekly hours to just 6 hours and 40 minutes (a six-day weekend!), and enjoy about 26% more free time than before. Or would you use this higher hourly wage rate to raise both your weekly earnings and your free time by some intermediate amount?
The idea of suddenly receiving a six-fold increase in your hourly wage and being able to choose your own hours of work might not seem very realistic. But we know from Unit 2 that technological progress since the Industrial Revolution has been accompanied by a dramatic rise in wages. In fact, the average real hourly earnings of American workers did increase more than six-fold during the twentieth century. And while employees ordinarily cannot just tell their employer how many hours they want to work, over long time periods the typical hours that we work do change. In part, this is a response to how much we prefer to work. As individuals, we can choose part-time work, although this may restrict our job options. Political parties also respond to the preferences of voters, so changes in typical working hours have occurred in many countries as a result of legislation that imposes maximum working hours.
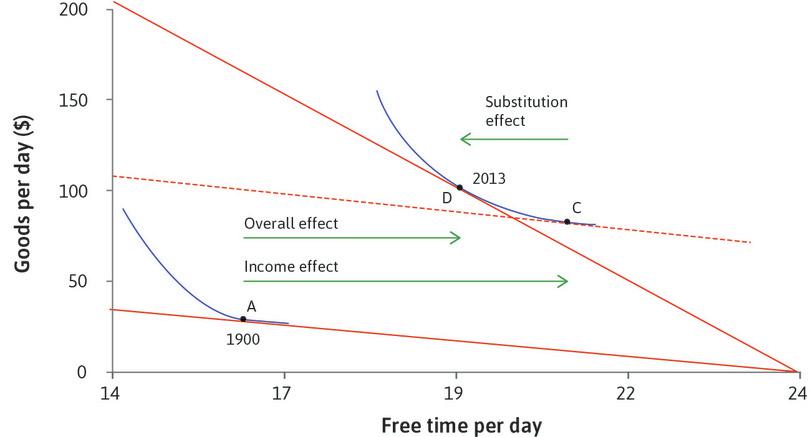
So have people used economic progress as a way to consume more goods, enjoy more free time, or both? The answer is both, but in different proportions in different countries. While hourly earnings increased by more than six-fold for twentieth century Americans, their average annual work time fell by a little more than one-third. So people at the end of this century enjoyed a four-fold increase in annual earnings with which they could buy goods and services, but a much smaller increase of slightly less than one-fifth in their free time. (The percentage increase in free time would be higher if you did not count time spent asleep as free time, but it is still small relative to the increase in earnings.) How does this compare with the choice you made when our hypothetical employer offered you a six-fold increase in your wage?
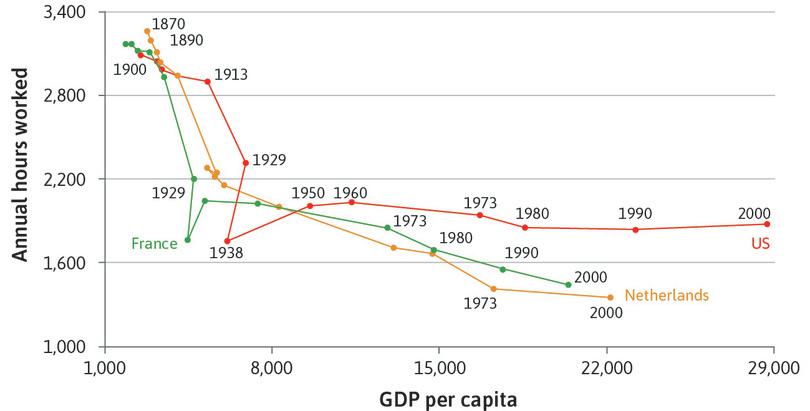
Figure 3.1 shows trends in income and working hours since 1870 in three countries.
As in Unit 1, income is measured as per-capita GDP in US dollars. This is not the same as average earnings, but gives us a useful indication of average income for the purposes of comparison across countries and through time. In the late nineteenth and early twentieth century, average income approximately trebled, and hours of work fell substantially. During the rest of the twentieth century, income per head rose four-fold.
Hours of work continued to fall in the Netherlands and France (albeit more slowly) but levelled off in the US, where there has been little change since 1960.
Annual hours of work and income (1870–2000).
Annual hours of work and income (1870–2000).
Figure 3.1 Annual hours of work and income (1870–2000).
Maddison Project. 2013. 2013 edition. Michael Huberman and Chris Minns. 2007. ‘The times they are not changin’: Days and hours of work in Old and New Worlds, 1870–2000’. Explorations in Economic History 44 (4): pp. 538–567. GDP is measured at PPP in 1990 international Geary-Khamis dollars.
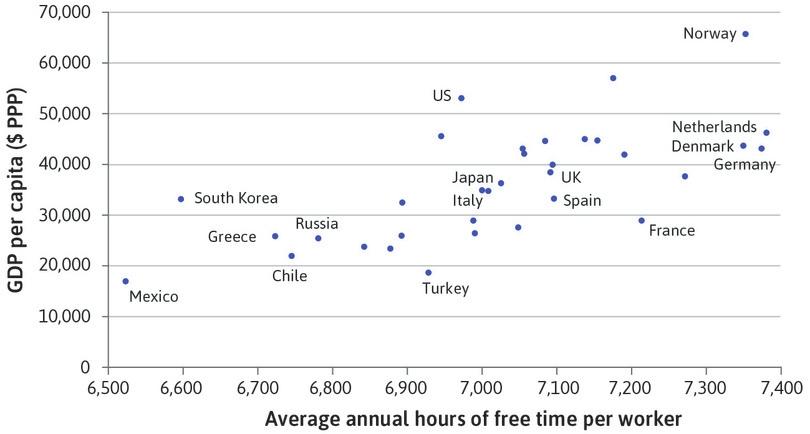
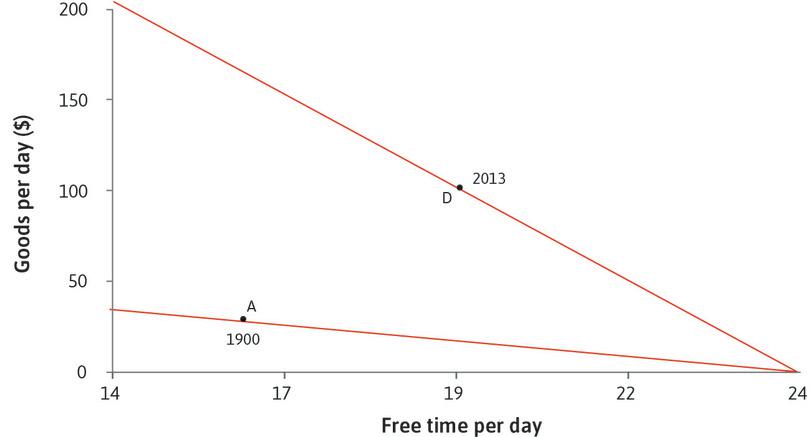
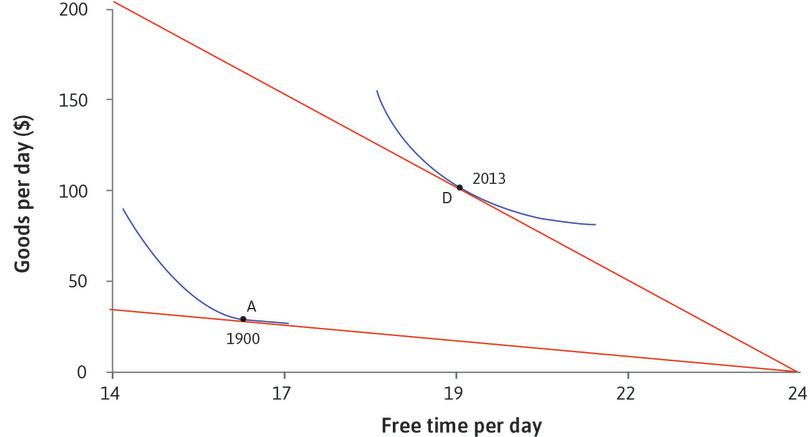
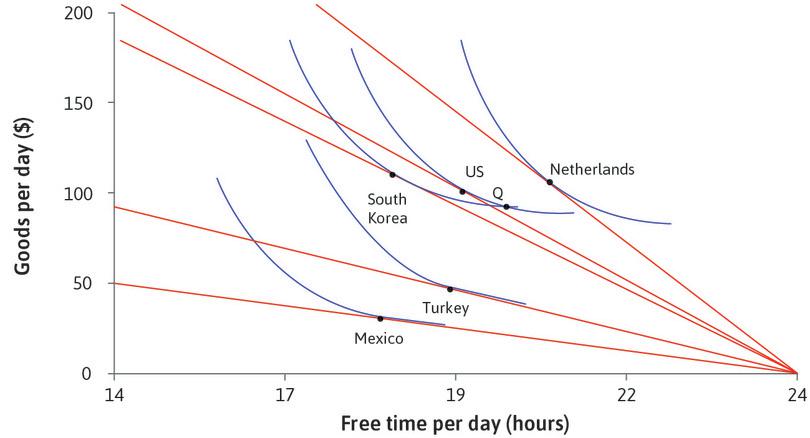
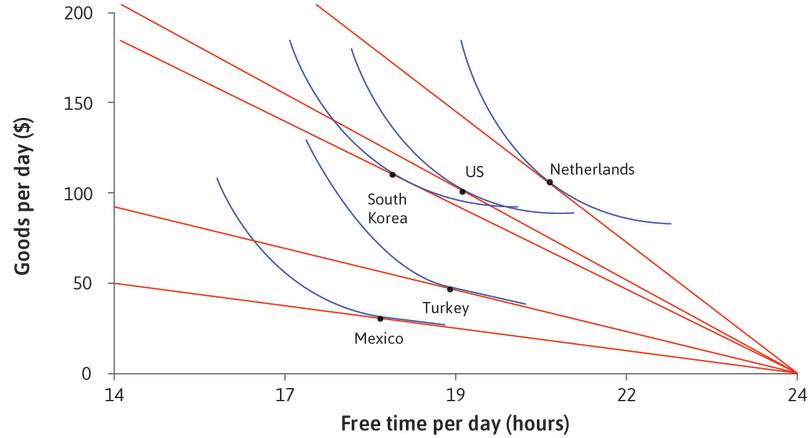
While many countries have experienced similar trends, there are still differences in outcomes. Figure 3.2 illustrates the wide disparities in free time and income between countries in 2013. Here we have calculated free time by subtracting average annual working hours from the number of hours in a year. You can see that the higher-income countries seem to have lower working hours and more free time, but there are also some striking differences between them. For example, the Netherlands and the US have similar levels of income, but Dutch workers have much more free time. And the US and Turkey have similar amounts of free time but a large difference in income.
Annual hours of free time per worker and income (2013).
Annual hours of free time per worker and income (2013).
Figure 3.2 Annual hours of free time per worker and income (2013).
OECD. Average annual hours actually worked per worker. OECD. Level of GDP per capita and productivity. Accessed June 2016. Data for South Korea refers to 2012.
In many countries there has been a huge increase in living standards since 1870. But in some places people have carried on working just as hard as before but consumed more, while in other countries people now have much more free time. Why has this happened? We will provide some answers to this question by studying a basic problem of economics—scarcity—and how we make choices when we cannot have all of everything that we want, such as goods and free time.
Study the model of decision making that we use carefully! It will be used repeatedly throughout the course, because it provides insight into a wide range of economic problems.
Question 3.1 Choose the correct answer(s)
Currently you work for 40 hours per week at the wage rate of £20 an hour. Your free hours are defined as the number of hours not spent in work per week, which in this case is 24 hours × 7 days − 40 hours = 128 hours per week. Suppose now that your wage rate has increased by 25%. If you are happy to keep your total weekly income constant, then:
- The new wage rate is £20 × 1.25 = £25 per hour. Your original weekly income is £20 × 40 hours = £800. Therefore, your new total number of working hours is £800/£25 per hour = 32 hours. This represents a change of (32 – 40)/40 = -20%.
- The new wage rate is £20 × 1.25 = £25 per hour. Your original weekly income is £20 × 40 hours = £800. Therefore, your new total number of working hours is £800/£25 per hour = 32 hours.
- The new wage rate is £20 × 1.25 = £25 per hour. Your original weekly income is £20 × 40 hours = £800. Therefore, your new total number of working hours is £800/£25 per hour = 32 hours. Then your free time is now 24 hours per day × 7 days per week – 32 = 136 hours per week, an increase of (136 – 128)/128 = 6.25% ? 25%.
- The new wage rate is £20 × 1.25 = £25 per hour. Your original weekly income is £20 × 40 hours = £800. Therefore, your new total number of working hours is £800/£25 per hour = 32 hours. Then your free time is now 24 × 7 – 32 = 136 hours per week, an increase of (136 – 128)/128 = 6.25%.
Question 3.2 Choose the correct answer(s)
Look again at Figure 3.1, which depicts the annual number of hours worked against GDP per capita in the US, France and the Netherlands, between 1870 and 2000. Which of the following is true?
- The negative relationship between the number of hours worked and GDP per capita does not necessarily imply that one causes the other.
- The lower GDP per capita in the Netherlands may be due to a number of factors, including the possibility that Dutch people may prefer less income but more leisure time for cultural or other reasons.
- The GDP per capita of France increased from roughly to $2,000 to $20,000 (ten-fold) while annual hours worked fell from over 3,000 to under 1,500.
- That would be nice. However past performance does not necessarily mean that the trend will continue in the future.
3.1 Labour and production
In Unit 2 we saw that labour can be thought of as an input in the production of goods and services. Labour is work; for example the welding, assembling, and testing required to make a car. Work activity is often difficult to measure, which is an important point in later units because employers find it difficult to determine the exact amount of work that their employees are doing. We also cannot measure the effort required by different activities in a comparable way (for example, baking a cake versus building a car), so economists often measure labour simply as the number of hours worked by individuals engaged in production, and assume that as the number of hours worked increases, the amount of goods produced also increases.
As a student, you make a choice every day: how many hours to spend studying. There may be many factors influencing your choice: how much you enjoy your work, how difficult you find it, how much work your friends do, and so on. Perhaps part of the motivation to devote time to studying comes from your belief that the more time you spend studying, the higher the grade you will be able to obtain at the end of the course. In this unit, we will construct a simple model of a student’s choice of how many hours to work, based on the assumption that the more time spent working, the better the final grade will be.
We assume a positive relationship between hours worked and final grade, but is there any evidence to back this up? A group of educational psychologists looked at the study behaviour of 84 students at Florida State University to identify the factors that affected their performance.1
At first sight there seems to be only a weak relationship between the average number of hours per week the students spent studying and their Grade Point Average (GPA) at the end of the semester. This is in Figure 3.3.
The 84 students have been split into two groups according to their hours of study. The average GPA for those with high study time is 3.43—only just above the GPA of those with low study time.
| High study time (42 students) | Low study time (42 students) | |
|---|---|---|
| Average GPA | 3.43 | 3.36 |
Study time and grades
42 students with high study time achieved GPAs of 3.43; 42 students with low study time achieved GPAs of 3.36
Figure 3.3 Study time and grades.
Elizabeth Ashby Plant, Karl Anders Ericsson, Len Hill, and Kia Asberg. 2005. ‘Why study time does not predict grade point average across college students: Implications of deliberate practice for academic performance.’ Contemporary Educational Psychology 30 (1): pp. 96–116. Additional calculations were conducted by Ashby Plant, Florida State University, in June 2015.
Looking more closely, we discover this study is an interesting illustration of why we should be careful when we make ceteris paribus assumptions (remember from Unit 2 that this means ‘holding other things constant’). Within each group of 42 students there are many potentially important differences. The conditions in which they study would be an obvious difference to consider: an hour working in a busy, noisy room may not be as useful as an hour spent in the library.
In Figure 3.4, we see that students studying in poor environments are more likely to study longer hours. Of these 42 students, 31 of them have high study time, compared with only 11 of the students with good environments. Perhaps they are distracted by other people around them, so it takes them longer to complete their assignments than students who work in the library.
| High study time | Low study time | |
|---|---|---|
| Good environment | 3.63 (11 students) | 3.43 (31 students) |
| Poor environment | 3.36 (31 students) | 3.17 (11 students) |
Figure 3.4 Average GPA in good and poor study environments.
Plant et al. ‘Why study time does not predict grade point average across college students’, ibid.
Now look at the average GPAs in the top row: if the environment is good, students who study longer do better—and you can see in the bottom row that high study time pays off for those who work in poor environments too. This relationship was not as clear when we didn’t consider the effect of the study environment.
So, after taking into account environment and other relevant factors (including the students’ past GPAs, and the hours they spent in paid work or partying), the psychologists estimated that an additional hour of study time per week raised a student’s GPA at the end of the semester by 0.24 points on average. If we take two students who are the same in all respects except for study time, we predict that the one who studies for longer will have a GPA that is 0.24 points higher for each extra hour: study time raises GPA by 0.24 per hour, ceteris paribus.
Exercise 3.1 Ceteris paribus assumptions
You have been asked to conduct a research study at your university, just like the one at Florida State University.
- In addition to study environment, which factors do you think should ideally be held constant in a model of the relationship between study hours and final grade?
- What information about the students would you want to collect beyond GPA, hours of study, and study environment?
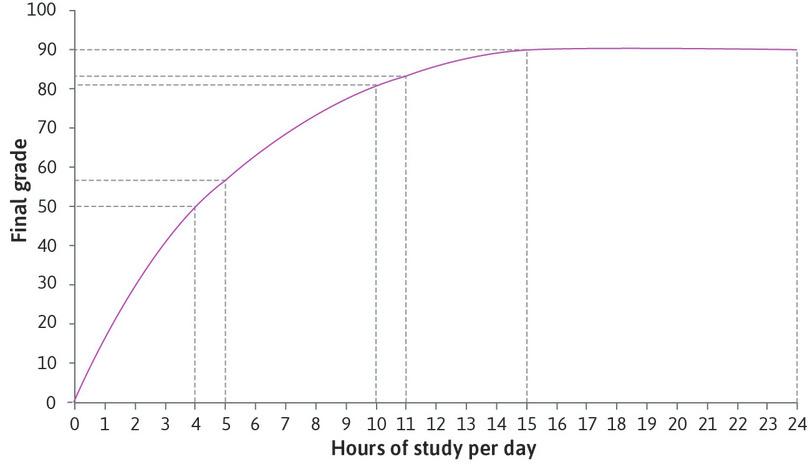
Now imagine a student, whom we will call Alexei. He can vary the number of hours he spends studying. We will assume that, as in the Florida study, the hours he spends studying over the semester will increase the percentage grade that he will receive at the end, ceteris paribus. This relationship between study time and final grade is represented in the table in Figure 3.5. In this model, study time refers to all of the time that Alexei spends learning, whether in class or individually, measured per day (not per week, as for the Florida students). The table shows how his grade will vary if he changes his study hours, if all other factors—his social life, for example—are held constant.
- production function
- A graphical or mathematical expression describing the amount of output that can be produced by any given amount or combination of input(s). The function describes differing technologies capable of producing the same thing.
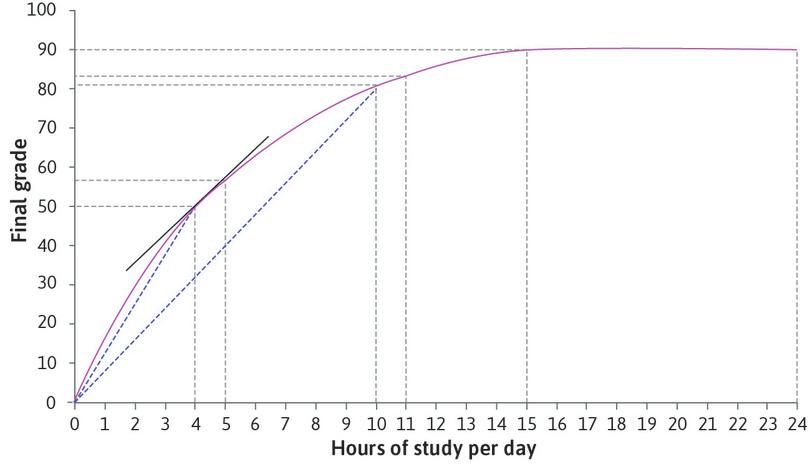
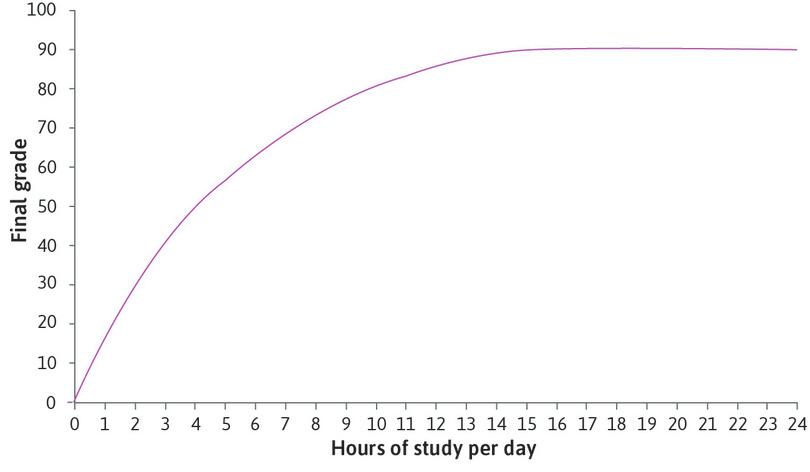
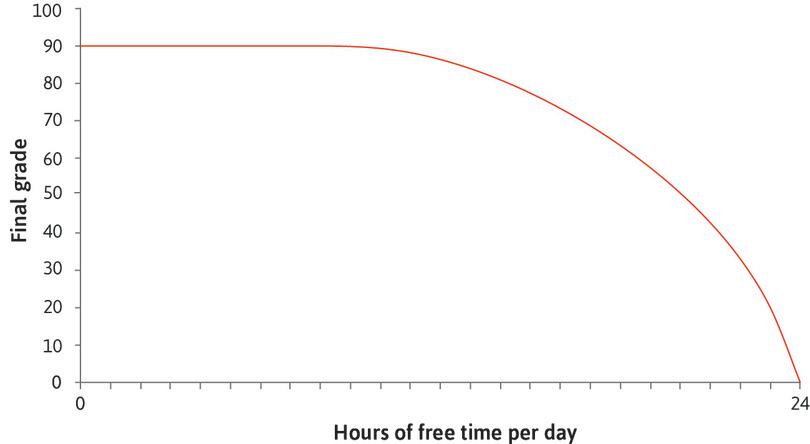
This is Alexei’s production function: it translates the number of hours per day spent studying (his input of labour) into a percentage grade (his output). In reality, the final grade might also be affected by unpredictable events (in everyday life, we normally lump the effect of these things together and call it ‘luck’). You can think of the production function as telling us what Alexei will get under normal conditions (if he is neither lucky nor unlucky).
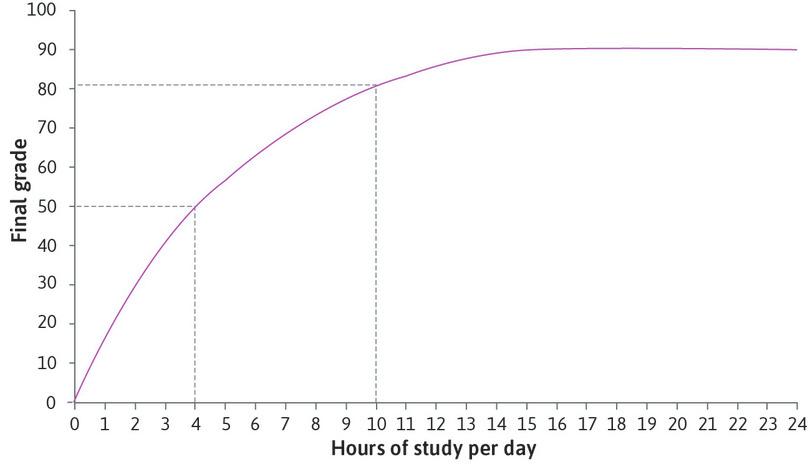
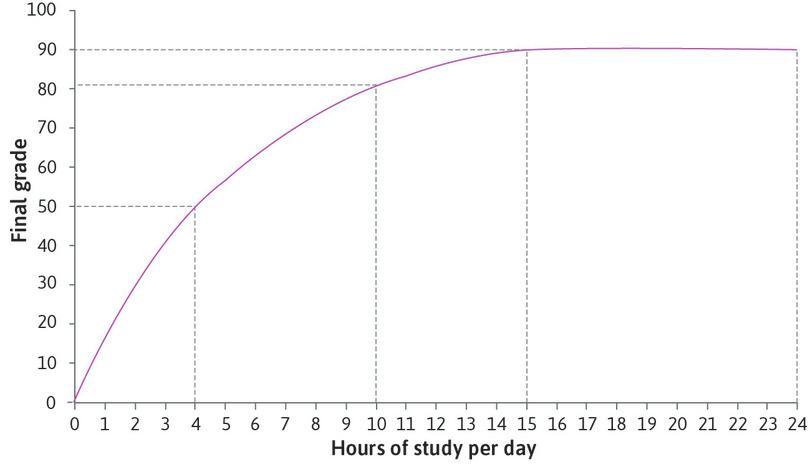
If we plot this relationship on a graph, we get the curve in Figure 3.5. Alexei can achieve a higher grade by studying more, so the curve slopes upward. At 15 hours of work per day he gets the highest grade he is capable of, which is 90%. Any time spent studying beyond that does not affect his exam result (he will be so tired that studying more each day will not achieve anything), and the curve becomes flat.
- average product
- Total output divided by a particular input, for example per worker (divided by the number of workers) or per worker per hour (total output divided by the total number of hours of labour put in).
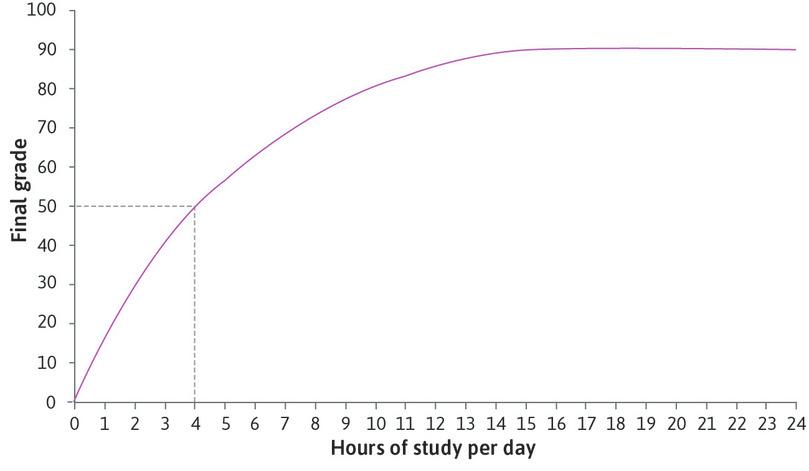
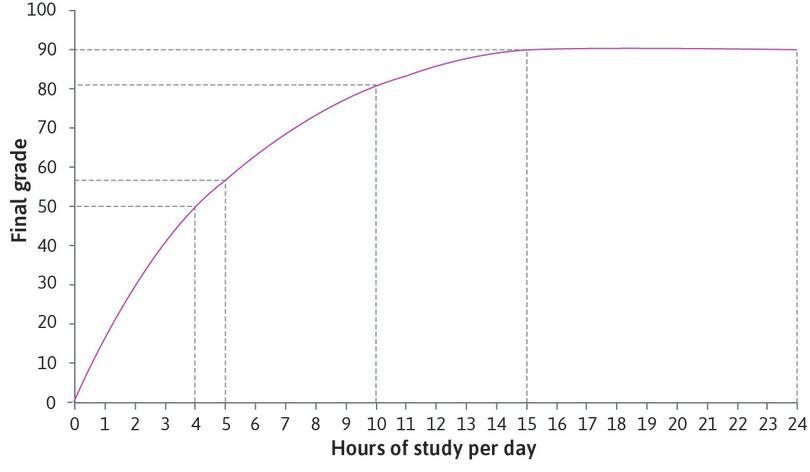
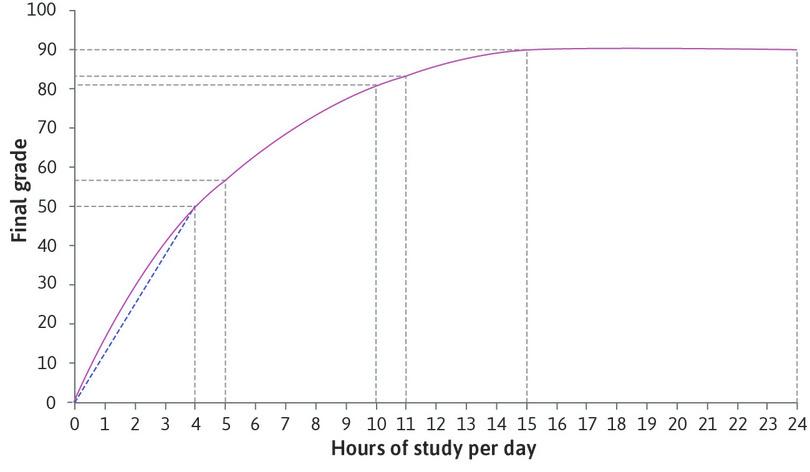
We can calculate Alexei’s average product of labour, as we did for the farmers in Unit 2. If he works for 4 hours per day, he achieves a grade of 50. The average product—the average number of percentage points per hour of study—is 50 / 4 = 12.5. In Figure 3.5 it is the slope of a ray from the origin to the curve at 4 hours per day:
- marginal product
- The additional amount of output that is produced if a particular input was increased by one unit, while holding all other inputs constant.
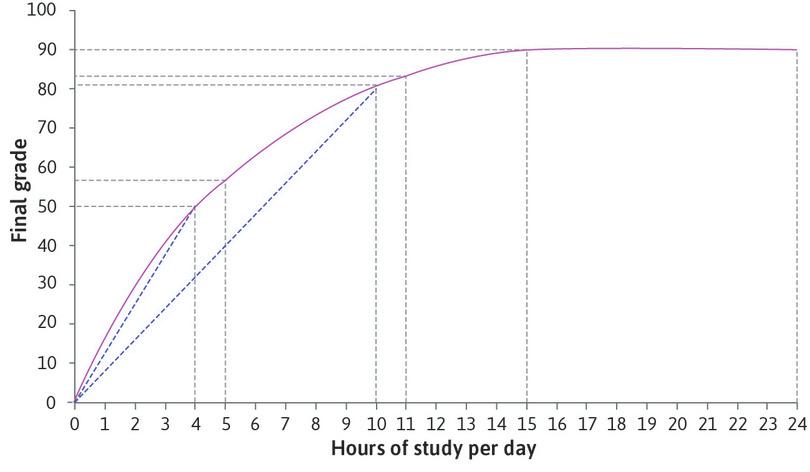
Alexei’s marginal product is the increase in his grade from increasing study time by one hour. Follow the steps in Figure 3.5 to see how to calculate the marginal product, and compare it with the average product.
At each point on the production function, the marginal product is the increase in the grade from studying one more hour. The marginal product corresponds to the slope of the production function.
Leibniz: Average and marginal productivity
- diminishing returns
- A situation in which the use of an additional unit of a factor of production results in a smaller increase in output than the previous increase. Also known as: diminishing marginal returns in production
Alexei’s production function in Figure 3.5 gets flatter the more hours he studies, so the marginal product of an additional hour falls as we move along the curve. The marginal product is diminishing. The model captures the idea that an extra hour of study helps a lot if you are not studying much, but if you are already studying a lot, then studying even more does not help very much.
Leibniz: Diminishing marginal productivity
- concave function
- A function of two variables for which the line segment between any two points on the function lies entirely below the curve representing the function (the function is convex when the line segment lies above the function).
In Figure 3.5, output increases as the input increases, but the marginal product falls—the function becomes gradually flatter. A production function with this shape is described as concave.
Leibniz: Concave and convex functions
If we compare the marginal and average products at any point on Alexei’s production function, we find that the marginal product is below the average product. For example, when he works for four hours his average product is 50/4 = 12.5 points per hour, but an extra hour’s work raises his grade from 50 to 57, so the marginal product is 7. This happens because the marginal product is diminishing: each hour is less productive than the ones that came before. And it implies that the average product is also diminishing: each additional hour of study per day lowers the average product of all his study time, taken as a whole.
This is another example of the diminishing average product of labour that we saw in Unit 2. In that case, the average product of labour in food production (the food produced per worker) fell as more workers cultivated a fixed area of land.
Lastly, notice that if Alexei was already studying for 15 hours a day, the marginal product of an additional hour would be zero. Studying more would not improve his grade. As you might know from experience, a lack of either sleep or time to relax could even lower Alexei’s grade if he worked more than 15 hours a day. If this were the case, then his production function would start to slope downward, and Alexei’s marginal product would become negative.
- tangency
- When two curves share one point in common but do not cross. The tangent to a curve at a given point is a straight line that touches the curve at that point but does not cross it.
Marginal change is an important and common concept in economics. You will often see it marked as a slope on a diagram. With a production function like the one in Figure 3.5, the slope changes continuously as we move along the curve. We have said that when Alexei studies for 4 hours a day the marginal product is 7, the increase in the grade from one more hour of study. Because the slope of the curve changes between 4 and 5 hours on the horizontal axis, this is only an approximation to the actual marginal product. More precisely, the marginal product is the rate at which the grade increases, per hour of additional study. In Figure 3.5 the true marginal product is the slope of the tangent to the curve at 4 hours. In this unit, we will use approximations so that we can work in whole numbers, but you may notice that sometimes these numbers are not quite the same as the slopes.
Exercise 3.2 Production functions
- Draw a graph to show a production function that, unlike Alexei’s, becomes steeper as the input increases.
- Can you think of an example of a production process that might have this shape? Why would the slope get steeper?
- What can you say about the marginal and average products in this case?
Marginal product
The marginal product is the rate of change of the grade at 4 hours of study. Suppose Alexei has been studying for 4 hours a day, and studies for 1 minute longer each day (a total of 4.016667 hours). Then, according to the graph, his grade will rise by a very small amount—about 0.124. A more precise estimate of the marginal product (the rate of change) would be:
If we looked at smaller changes in study time even further (the rise in grade for each additional second of study per day, for example) we would get closer to the true marginal product, which is the slope of the tangent to the curve at 4 hours of study.
Question 3.3 Choose the correct answer(s)
Figure 3.5 shows Alexei’s production function, with the final grade (the output) related to the number of hours spent studying (the input).
Which of the following is true?
- Because there are no previous hours to consider, the average product for the initial hour is just the improvement produced by a single hour, which in turn approximates to the marginal product from 0 to 1 hours (the precise marginal product changes over this interval, reflected in the decreasing slope of the production function).
- The marginal product is constant beyond 15 hours, but the average product continues to diminish. This is because the marginal product (zero) is less than the average product, which remains positive but is decreasing (more hours with no additional improvement reduces the average).
- If studying for more than 15 hours had a negative effect on Alexei’s grade, then the marginal product would be negative, implying a downward-sloping curve beyond 15 hours.
- The average product at 20 hours is 90 grade points/20 hours = 4.5 points per hour. The marginal product, however, is zero – as indicated by the production function being flat beyond 15 hours.
3.2 Preferences
- preferences
- A description of the benefit or cost we associate with each possible outcome.
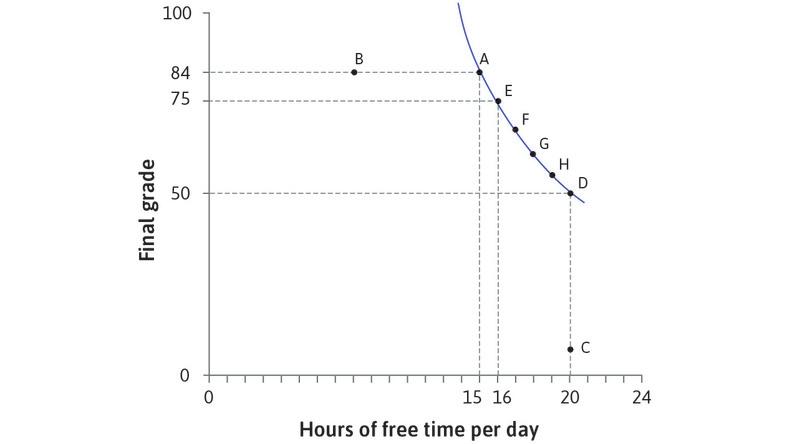
If Alexei has the production function shown in Figure 3.5, how many hours per day will he choose to study? The decision depends on his preferences—the things that he cares about. If he cared only about grades, he should study for 15 hours a day. But, like other people, Alexei also cares about his free time—he likes to sleep, go out or watch TV. So he faces a trade-off: how many percentage points is he willing to give up in order to spend time on things other than study?
We illustrate his preferences using Figure 3.6, with free time on the horizontal axis and final grade on the vertical axis. Free time is defined as all the time that he does not spend studying. Every point in the diagram represents a different combination of free time and final grade. Given his production function, not every combination that Alexei would want will be possible, but for the moment we will only consider the combinations that he would prefer.
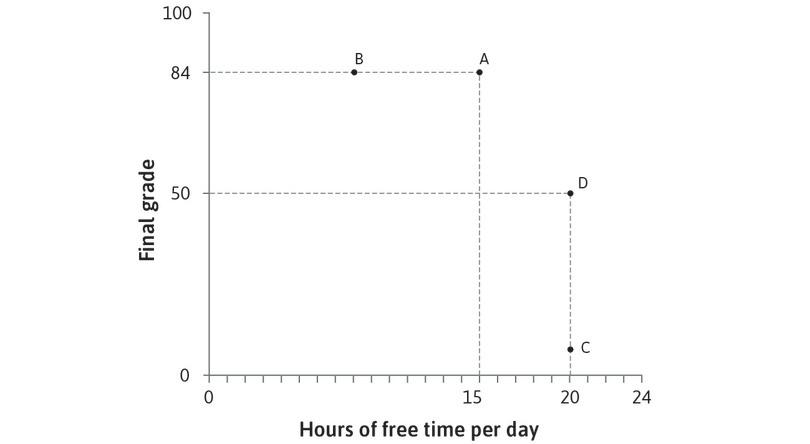
We can assume:
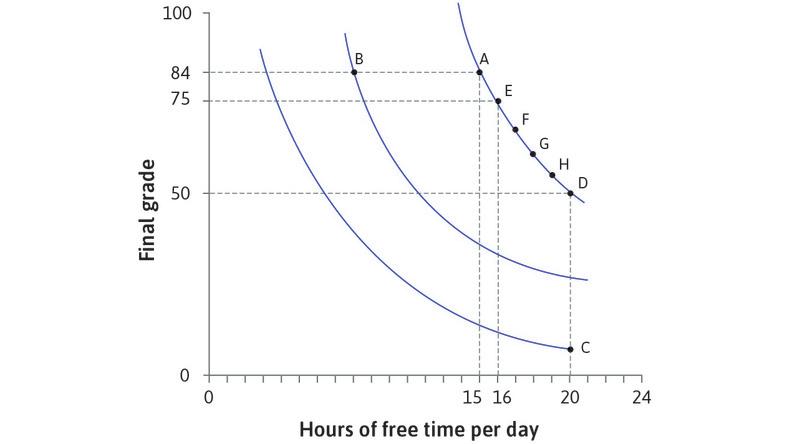
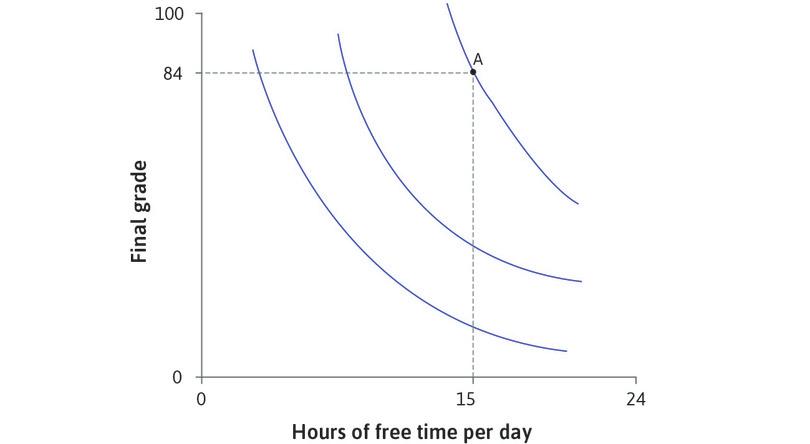
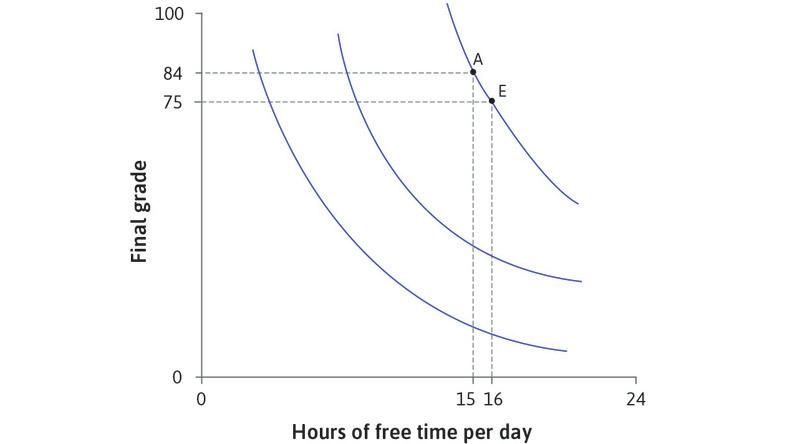
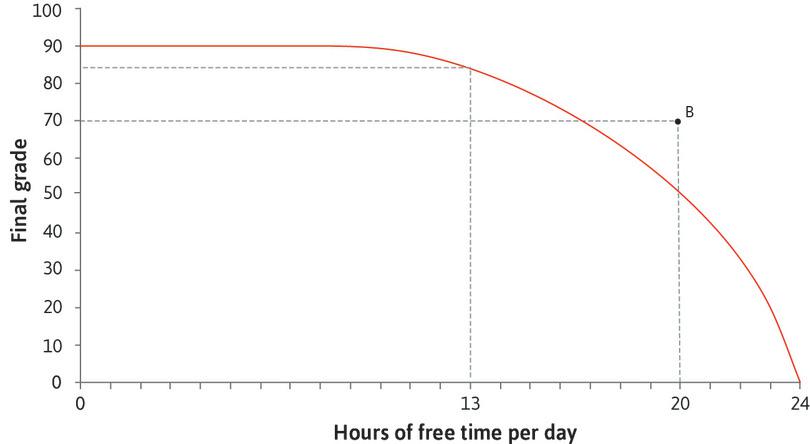
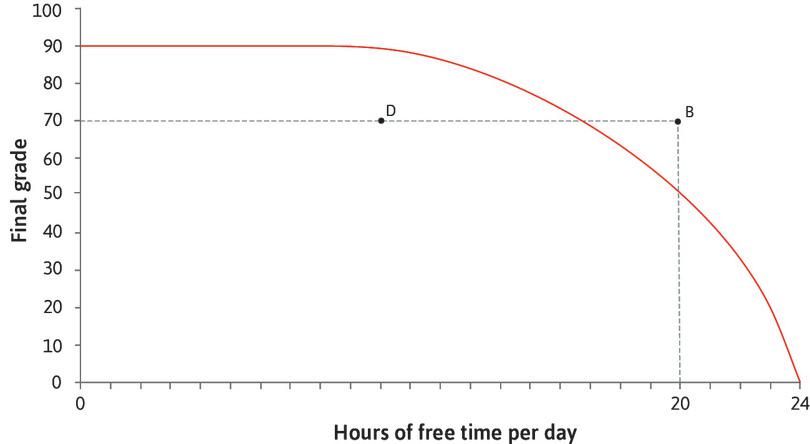
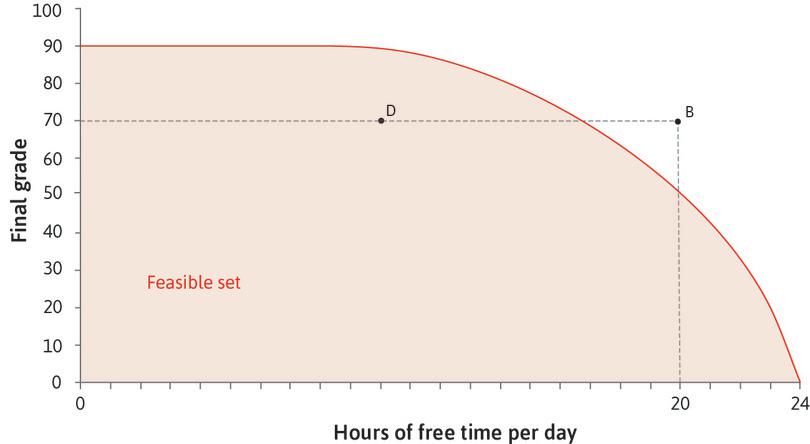
- For a given grade, he prefers a combination with more free time to one with less free time. Therefore, even though both A and B in Figure 3.6 correspond to a grade of 84, Alexei prefers A because it gives him more free time.
- Similarly, if two combinations both have 20 hours of free time, he prefers the one with a higher grade.
- But compare points A and D in the table. Would Alexei prefer D (low grade, plenty of time) or A (higher grade, less time)? One way to find out would be to ask him.
- utility
- A numerical indicator of the value that one places on an outcome, such that higher valued outcomes will be chosen over lower valued ones when both are feasible.
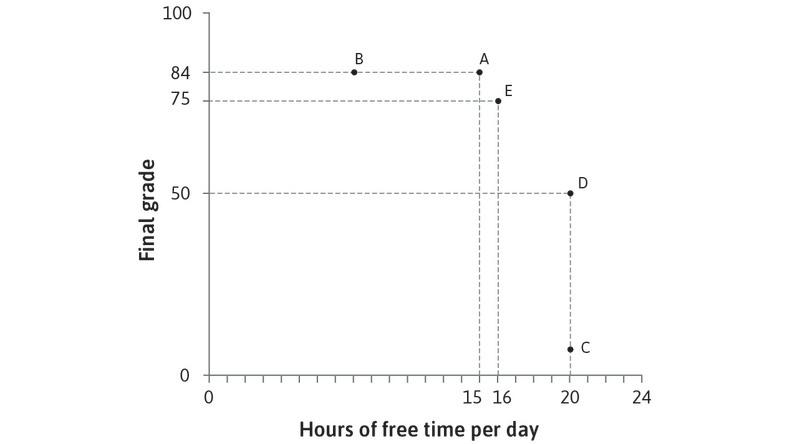
Suppose he says he is indifferent between A and D, meaning he would feel equally satisfied with either outcome. We say that these two outcomes would give Alexei the same utility. And we know that he prefers A to B, so B provides lower utility than A or D.
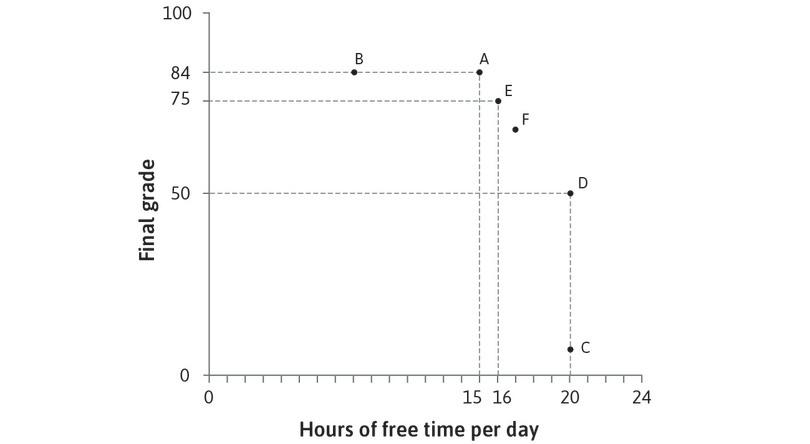
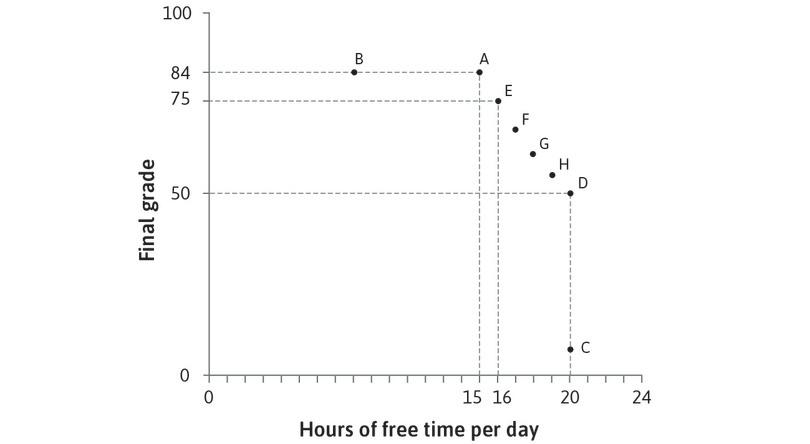
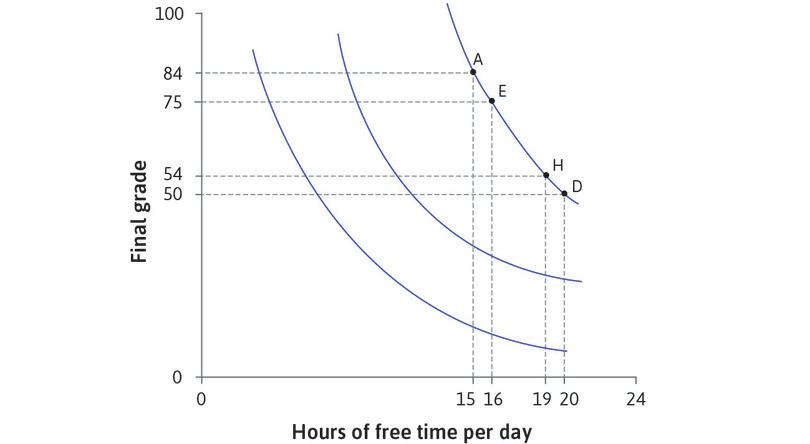
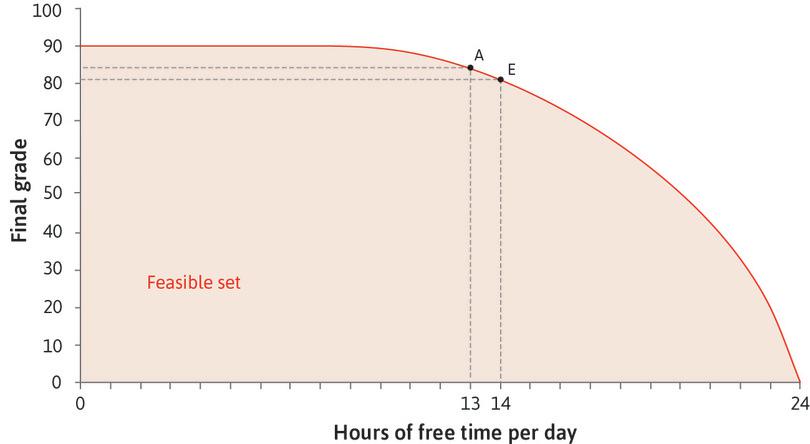
A systematic way to graph Alexei’s preferences would be to start by looking for all of the combinations that give him the same utility as A and D. We could ask Alexei another question: ‘Imagine that you could have the combination at A (15 hours of free time, 84 points). How many points would you be willing to sacrifice for an extra hour of free time?’ Suppose that after due consideration, he answers ‘nine’. Then we know that he is indifferent between A and E (16 hours, 75 points). Then we could ask the same question about combination E, and so on until point D. Eventually we could draw up a table like the one in Figure 3.6. Alexei is indifferent between A and E, between E and F, and so on, which means he is indifferent between all of the combinations from A to D.
- indifference curve
- A curve of the points which indicate the combinations of goods that provide a given level of utility to the individual.
The combinations in the table are plotted in Figure 3.6, and joined together to form a downward-sloping curve, called an indifference curve, which joins together all of the combinations that provide equal utility or ‘satisfaction’.
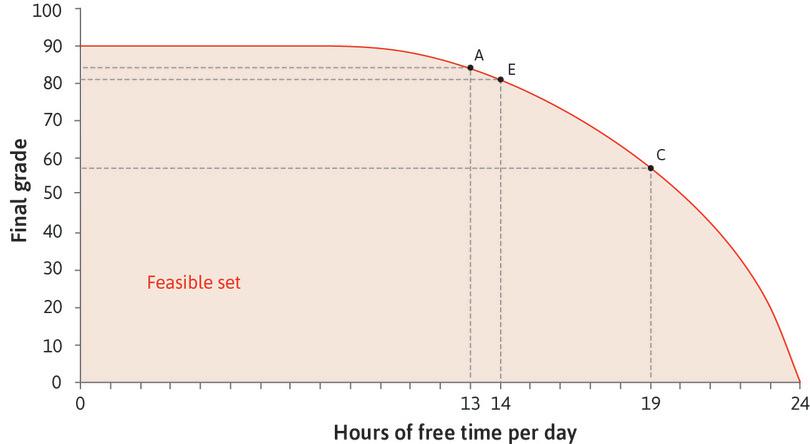
If you look at the three curves drawn in Figure 3.6, you can see that the one through A gives higher utility than the one through B. The curve through C gives the lowest utility of the three. To describe preferences we don’t need to know the exact utility of each option; we only need to know which combinations provide more or less utility than others.
- consumption good
- A good or service that satisfies the needs of consumers over a short period.
The curves we have drawn capture our typical assumptions about people’s preferences between two goods. In other models, these will often be consumption goods such as food or clothing, and we refer to the person as a consumer. In our model of a student’s preferences, the goods are ‘final grade’ and ‘free time’. Notice that:
- Indifference curves slope downward due to trade-offs: If you are indifferent between two combinations, the combination that has more of one good must have less of the other good.
- Higher indifference curves correspond to higher utility levels: As we move up and to the right in the diagram, further away from the origin, we move to combinations with more of both goods.
- Indifference curves are usually smooth: Small changes in the amounts of goods don’t cause big jumps in utility.
- Indifference curves do not cross: Why? See Exercise 3.3.
- As you move to the right along an indifference curve, it becomes flatter.
- marginal rate of substitution (MRS)
- The trade-off that a person is willing to make between two goods. At any point, this is the slope of the indifference curve. See also: marginal rate of transformation.
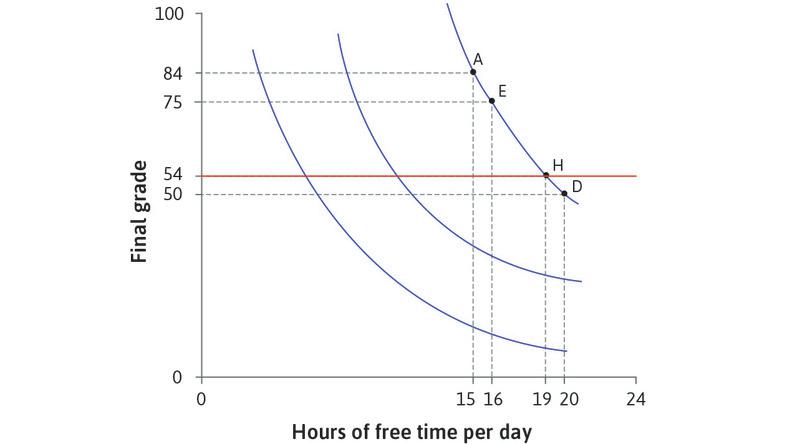
To understand the last property in the list, look at Alexei’s indifference curves, which are plotted again in Figure 3.7. If he is at A, with 15 hours of free time and a grade of 84, he would be willing to sacrifice 9 percentage points for an extra hour of free time, taking him to E (remember that he is indifferent between A and E). We say that his marginal rate of substitution (MRS) between grade points and free time at A is nine; it is the reduction in his grade that would keep Alexei’s utility constant following a one-hour increase of free time.
We have drawn the indifference curves as becoming gradually flatter because it seems reasonable to assume that the more free time and the lower the grade he has, the less willing he will be to sacrifice further percentage points in return for free time, so his MRS will be lower. In Figure 3.7 we have calculated the MRS at each combination along the indifference curve. You can see that, when Alexei has more free time and a lower grade, the MRS—the number of percentage points he would give up to get an extra hour of free time—gradually falls.
The MRS is just the slope of the indifference curve, and it falls as we move to the right along the curve. If you think about moving from one point to another in Figure 3.7, you can see that the indifference curves get flatter if you increase the amount of free time, and steeper if you increase the grade. When free time is scarce relative to grade points, Alexei is less willing to sacrifice an hour for a higher grade: his MRS is high and his indifference curve is steep.
As the analysis in Figure 3.7 shows, if you move up the vertical line through 15 hours, the indifference curves get steeper: the MRS increases. For a given amount of free time, Alexei is willing to give up more grade points for an additional hour when he has a lot of points compared to when he has few (for example, if he was in danger of failing the course). By the time you reach A, where his grade is 84, the MRS is high; grade points are so plentiful here that he is willing to give up 9 percentage points for an extra hour of free time.
Leibniz: Indifference curves and the marginal rate of substitution
You can see the same effect if you fix the grade and vary the amount of free time. If you move to the right along the horizontal line for a grade of 54, the MRS becomes lower at each indifference curve. As free time becomes more plentiful, Alexei becomes less and less willing to give up grade points for more time.
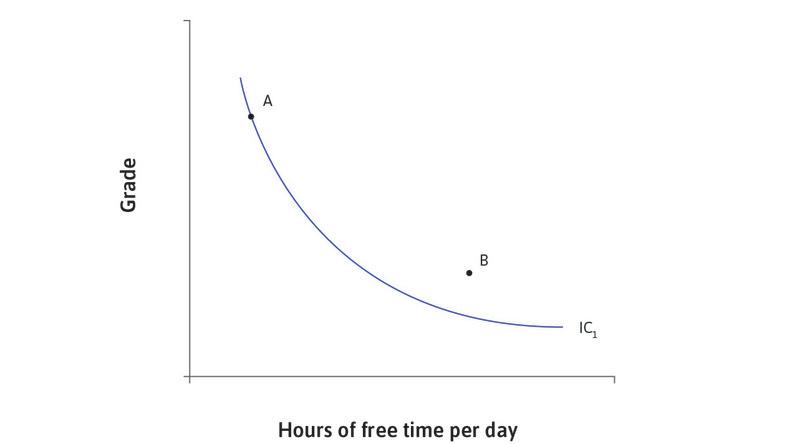
Exercise 3.3 Why indifference curves never cross
In the diagram below, IC1 is an indifference curve joining all the combinations that give the same level of utility as A. Combination B is not on IC1.
- Does combination B give higher or lower utility than combination A? How do you know?
- Draw a sketch of the diagram, and add another indifference curve, IC2, that goes through B and crosses IC1. Label the point at which they cross as C.
- Combinations B and C are both on IC2. What does that imply about their levels of utility?
- Combinations C and A are both on IC1. What does that imply about their levels of utility?
- According to your answers to (3) and (4), how do the levels of utility at combinations A and B compare?
- Now compare your answers to (1) and (5), and explain how you know that indifference curves can never cross.
Exercise 3.4 Your marginal rate of substitution
Imagine that you are offered a job at the end of your university course with a salary per hour (after taxes) of £12.50. Your future employer then says that you will work for 40 hours per week leaving you with 128 hours of free time per week. You tell a friend: ‘at that wage, 40 hours is exactly what I would like.’
- Draw a diagram with free time on the horizontal axis and weekly pay on the vertical axis, and plot the combination of hours and the wage corresponding to your job offer, calling it A. Assume you need about 10 hours a day for sleeping and eating, so you may want to draw the horizontal axis with 70 hours at the origin.
- Now draw an indifference curve so that A represents the hours you would have chosen yourself.
- Now imagine you were offered another job requiring 45 hours of work per week. Use the indifference curve you have drawn to estimate the level of weekly pay that would make you indifferent between this and the original offer.
- Do the same for another job requiring 35 hours of work per week. What level of weekly pay would make you indifferent between this and the original offer?
- Use your diagram to estimate your marginal rate of substitution between pay and free time at A.
Question 3.4 Choose the correct answer(s)
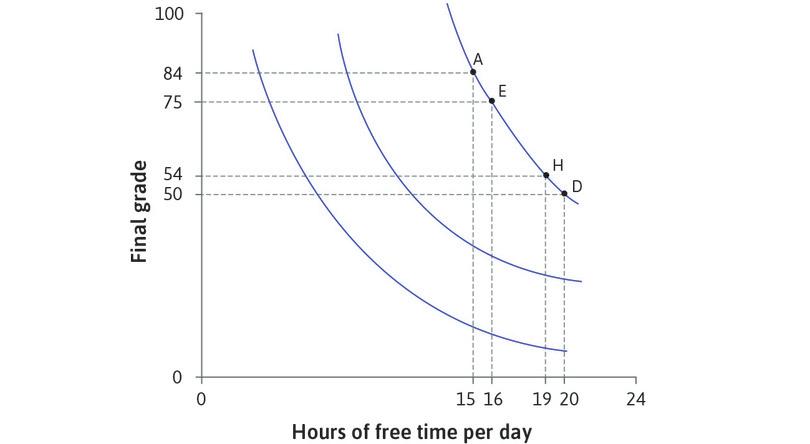
Figure 3.6 shows Alexei’s indifference curves for free time and final grade. Which of the following is true?
- The indifference curve through C is lower than that through B. Hence Alexei prefers B to C.
- A, where Alexei has the grade of 84 and 15 hours of free time, and D, where Alexei has the grade of 50 with 20 hours of free time, are on the same indifference curve.
- At D Alexei has the same amount of free time but a higher grade.
- The opposite trade-off is true: going from G to D, Alexei is willing to give up 10 grade points for 2 extra hours of free time. Going from G to E, he is willing to give up 2 hours of free time for 15 extra grade points.
Question 3.5 Choose the correct answer(s)
What is the marginal rate of substitution (MRS)?
- The marginal rate of substitution represents the ratio of the trade-off at the margin, in other words, how much of one good the consumer is willing to sacrifice for one extra unit of the other.
- This is the definition of the marginal rate of substitution.
- The MRS is the amount of one good that can be substituted for one unit of the other while keeping utility constant.
- The slope of the indifference curve represents the marginal rate of substitution: the trade-off between two goods that keeps utility constant.
3.3 Opportunity costs
- opportunity cost
- When taking an action implies forgoing the next best alternative action, this is the net benefit of the foregone alternative.
Alexei faces a dilemma: we know from looking at his preferences that he wants both his grade and his free time to be as high as possible. But given his production function, he cannot increase his free time without getting a lower grade in the exam. Another way of expressing this is to say that free time has an opportunity cost: to get more free time, Alexei has to forgo the opportunity of getting a higher grade.
In economics, opportunity costs are relevant whenever we study individuals choosing between alternative and mutually exclusive courses of action. When we consider the cost of taking action A we include the fact that if we do A, we cannot do B. So ‘not doing B’ becomes part of the cost of doing A. This is called an opportunity cost because doing A means forgoing the opportunity to do B.
Imagine that an accountant and an economist have been asked to report the cost of going to a concert, A, in a theatre, which has a $25 admission cost. In a nearby park there is concert B, which is free but happens at the same time.
- Accountant
- The cost of concert A is your ‘out-of-pocket’ cost: you paid $25 for a ticket, so the cost is $25.
- Economist
- But what do you have to give up to go to concert A? You give up $25, plus the enjoyment of the free concert in the park. So the cost of concert A for you is the out-of-pocket cost plus the opportunity cost.
Suppose that the most you would have been willing to pay to attend the free concert in the park (if it wasn’t free) was $15. The benefit of your next best alternative to concert A would be $15 of enjoyment in the park. This is the opportunity cost of going to concert A.
- economic cost
- The out-of-pocket cost of an action, plus the opportunity cost.
So the total economic cost of concert A is $25 + $15 = $40. If the pleasure you anticipate from being at concert A is greater than the economic cost, say $50, then you will forego concert B and buy a ticket to the theatre. On the other hand, if you anticipate $35 worth of pleasure from concert A, then the economic cost of $40 means you will not choose to go to the theatre. In simple terms, given that you have to pay $25 for the ticket, you will instead opt for concert B, pocketing the $25 to spend on other things and enjoying $15 worth of benefit from the free park concert.
Why don’t accountants think this way? Because it is not their job. Accountants are paid to keep track of money, not to provide decision rules on how to choose among alternatives, some of which do not have a stated price. But making sensible decisions and predicting how sensible people will make decisions involve more than keeping track of money. An accountant might argue that the park concert is irrelevant:
- Accountant
- Whether or not there is a free park concert does not affect the cost of going to the concert A. The cost to you is always $25.
- Economist
- But whether or not there is a free park concert can affect whether you go to concert A or not, because it changes your available options. If your enjoyment from A is $35 and your next best alternative is staying at home, with enjoyment of $0, you will choose concert A. However, if concert B is available, you will choose it over A.
- economic rent
- A payment or other benefit received above and beyond what the individual would have received in his or her next best alternative (or reservation option). See also: reservation option.
In Unit 2, we said that if an action brings greater net benefits than the next best alternative, it yields an economic rent and you will do it. Another way of saying this is that you receive an economic rent from taking an action when it results in a benefit greater than its economic cost (the sum of out-of-pocket and opportunity costs).
The table in Figure 3.8 summarizes the example of your choice of which concert to attend.
| A high value on the theatre choice (A) | A low value on the theatre choice (A) | |
|---|---|---|
| Out-of-pocket cost (price of ticket for A) | $25 | $25 |
| Opportunity cost (foregone pleasure of B, park concert) | $15 | $15 |
| Economic cost (sum of out-of-pocket and opportunity cost) | $40 | $40 |
| Enjoyment of theatre concert (A) | $50 | $35 |
| Economic rent (enjoyment minus economic cost) | $10 | −$5 |
| Decision | A: Go to the theatre concert. | B: Go to the park concert. |
Opportunity costs and economic rent: Which concert will you choose?
Opportunity costs and economic rent relating to a choice between concerts in park or theatre.
Figure 3.8 Opportunity costs and economic rent: Which concert will you choose?
Question 3.6 Choose the correct answer(s)
You are a taxi driver in Melbourne who earns A$50 for a day’s work. You have been offered a one-day ticket to the Australian Open for A$40. As a tennis fan, you value the experience at A$100. With this information, what can we say?
- By going to the Open you are foregoing the opportunity of earning A$50 from taxi driving. This is your opportunity cost.
- The economic cost is the sum of the actual price you pay plus the opportunity cost, which in this case is A$40 + A$50 = A$90.
- The economic rent of an action is its benefit minus its economic cost (out-of-pocket plus opportunity costs). Therefore, the economic rent is A$100 – A$40 – A$50 = A$10.
- The maximum price you would have paid for the ticket is the price at which your economic rent would be zero, which in this case is A$50.
Exercise 3.5 Opportunity costs
The British government introduced legislation in 2012 that gave universities the option to raise their tuition fees. Most chose to increase annual tuition fees for students from £3,000 to £9,000.
Does this mean that the cost of going to university has tripled? (Think about how an accountant and an economist might answer this question. To simplify, assume that the tuition fee is an ‘out of pocket’ cost. Ignore student loans.)
3.4 The feasible set
Now we return to Alexei’s problem of how to choose between grades and free time. Free time has an opportunity cost in the form of lost percentage points in his grade (equivalently, we might say that percentage points have an opportunity cost in the form of the free time Alexei has to give up to obtain them). But before we can describe how Alexei resolves his dilemma, we need to work out precisely which alternatives are available to him.
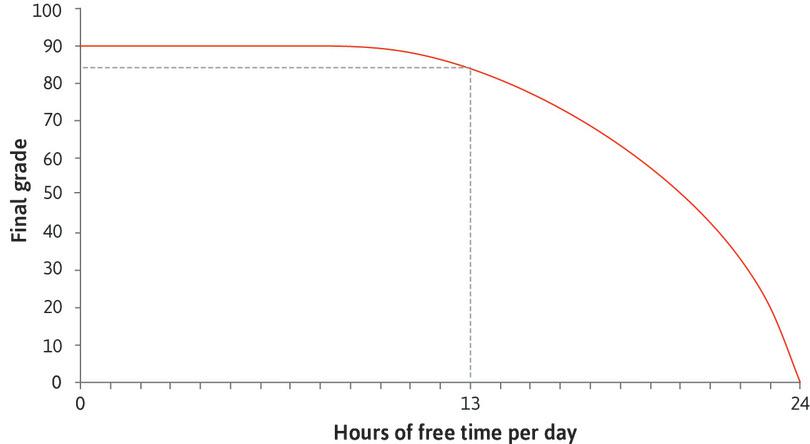
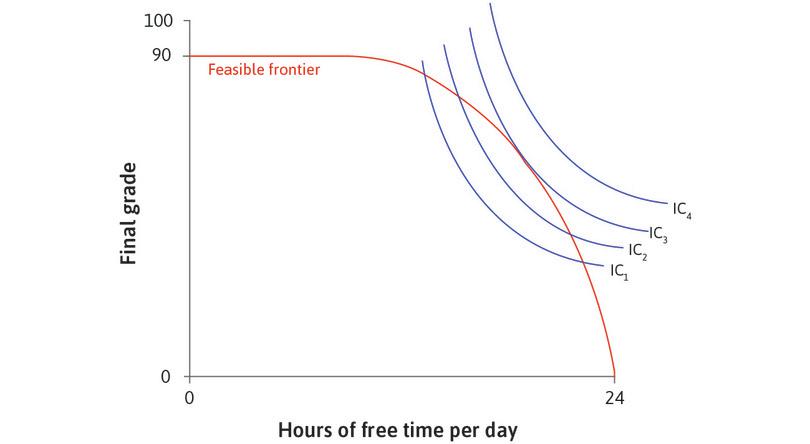
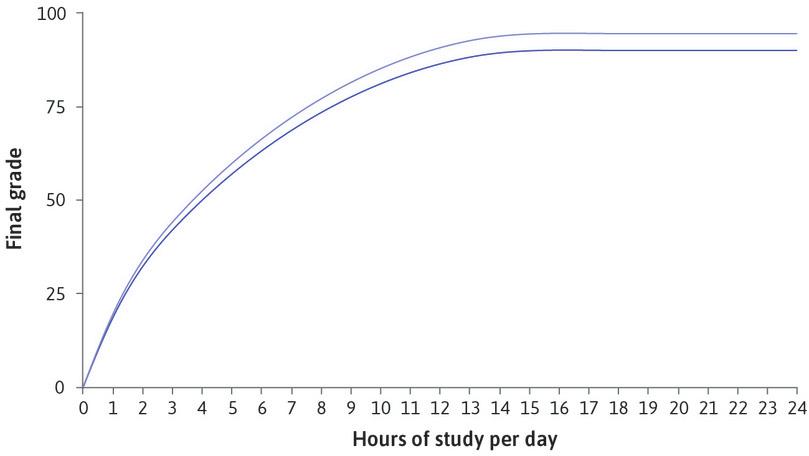
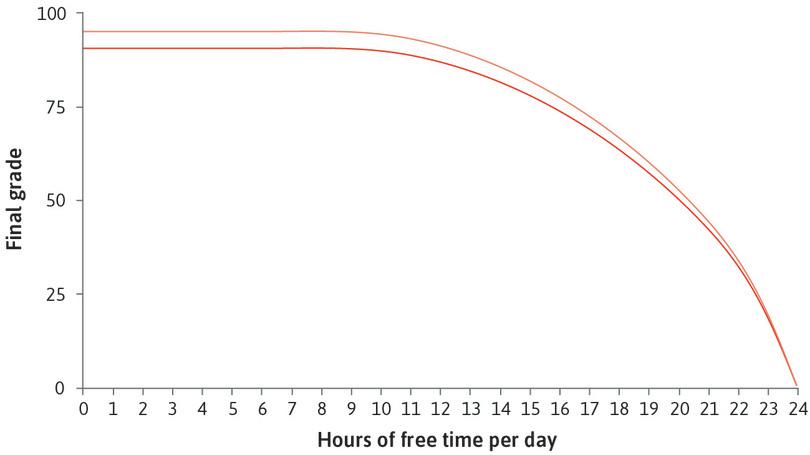
To answer this question, we look again at the production function. This time we will show how the final grade depends on the amount of free time, rather than study time. There are 24 hours in a day. Alexei must divide this time between studying (all the hours devoted to learning) and free time (all the rest of his time). Figure 3.9 shows the relationship between his final grade and hours of free time per day—the mirror image of Figure 3.5. If Alexei studies solidly for 24 hours, that means zero hours of free time and a final grade of 90. If he chooses 24 hours of free time per day, we assume he will get a grade of zero.
- feasible frontier
- The curve made of points that defines the maximum feasible quantity of one good for a given quantity of the other. See also: feasible set.
In Figure 3.9, the axes are final grade and free time, the two goods that give Alexei utility. If we think of him choosing to consume a combination of these two goods, the curved line in Figure 3.9 shows what is feasible. It represents his feasible frontier: the highest grade he can achieve given the amount of free time he takes. Follow the steps in Figure 3.9 to see which combinations of grade and free time are feasible, and which are not, and how the slope of the frontier represents the opportunity cost of free time.
- feasible set
- All of the combinations of the things under consideration that a decision-maker could choose given the economic, physical or other constraints that he faces. See also: feasible frontier.
Any combination of free time and final grade that is on or inside the frontier is feasible. Combinations outside the feasible frontier are said to be infeasible given Alexei’s abilities and conditions of study. On the other hand, even though a combination lying inside the frontier is feasible, choosing it would imply Alexei has effectively thrown away something that he values. If he studied for 14 hours a day, then according to our model, he could guarantee himself a grade of 89. But he could obtain a lower grade (70, say), if he just stopped writing before the end of the exam. It would be foolish to throw away points like this for no reason, but it would be possible. Another way to obtain a combination inside the frontier might be to sit in the library doing nothing—Alexei would be taking less free time than is available to him, which again makes no sense.
By choosing a combination inside the frontier, Alexei would be giving up something that is freely available—something that has no opportunity cost. He could obtain a higher grade without sacrificing any free time, or have more time without reducing his grade.
The feasible frontier is a constraint on Alexei’s choices. It represents the trade-off he must make between grade and free time. At any point on the frontier, taking more free time has an opportunity cost in terms of grade points foregone, corresponding to the slope of the frontier.
- marginal rate of transformation (MRT)
- The quantity of some good that must be sacrificed to acquire one additional unit of another good. At any point, it is the slope of the feasible frontier. See also: marginal rate of substitution.
Another way to express the same idea is to say that the feasible frontier shows the marginal rate of transformation: the rate at which Alexei can transform free time into grade points. Look at the slope of the frontier between points A and E in Figure 3.9.
- The slope of AE (vertical distance divided by horizontal distance) is −3.
- At point A, Alexei could get one more unit of free time by giving up 3 grade points. The opportunity cost of a unit of free time is 3.
- At point E, Alexei could transform one unit of time into 3 grade points. The marginal rate at which he can transform free time into grade points is 3.
Note that the slope of AE is only an approximation to the slope of the frontier. More precisely, the slope at any point is the slope of the tangent, and this represents both the MRT and the opportunity cost at that point.
Note that we have now identified two trade-offs:
Leibniz: Marginal rates of transformation and substitution
- The marginal rate of substitution (MRS): In the previous section, we saw that it measures the trade-off that Alexei is willing to make between final grade and free time.
- The marginal rate of transformation (MRT): In contrast, this measures the trade-off that Alexei is constrained to make by the feasible frontier.
As we shall see in the next section, the choice Alexei makes between his grade and his free time will strike a balance between these two trade-offs.
Question 3.7 Choose the correct answer(s)
Look at Figure 3.5 which shows Alexei’s production function: how the final grade (the output) depends on the number of hours spent studying (the input).
Free time per day is given by 24 hours minus the hours of study per day. Consider Alexei’s feasible set of combinations of final grade and hours of free time per day. What can we conclude?
- The hours of free time per day is already given as 24 hours minus the hours of study per day. Therefore, the number of hours spent sleeping is included in the hours of free time.
- Given that the production function is just the feasible frontier except that it takes negative free time (hours of study) as its input, the former is simply the latter mirrored across the horizontal axis and shifted horizontally.
- The production function is horizontal after 15 hours of study per day. Therefore, the feasible frontier is horizontal only up to 9 hours of free time per day.
- 10 hours of study is equivalent to 14 hours of free time given a 24-hour day, and the marginal product of labour (additional output per labour hour) is the same as the marginal rate of transformation (trade-off between extra output and labour), so these two values are equal.
3.5 Decision making and scarcity
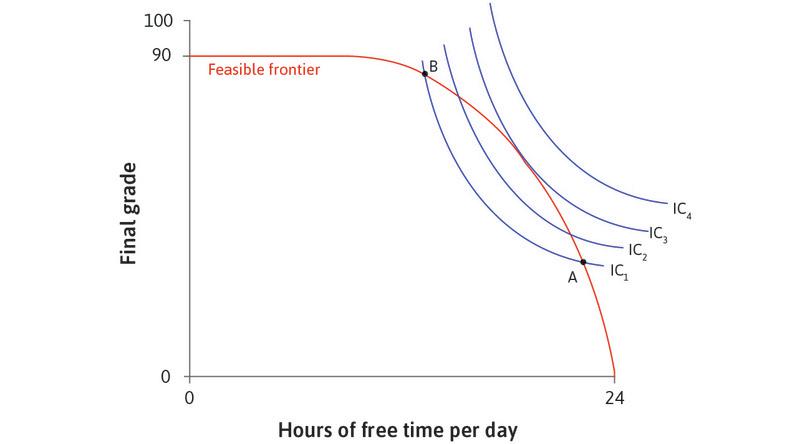
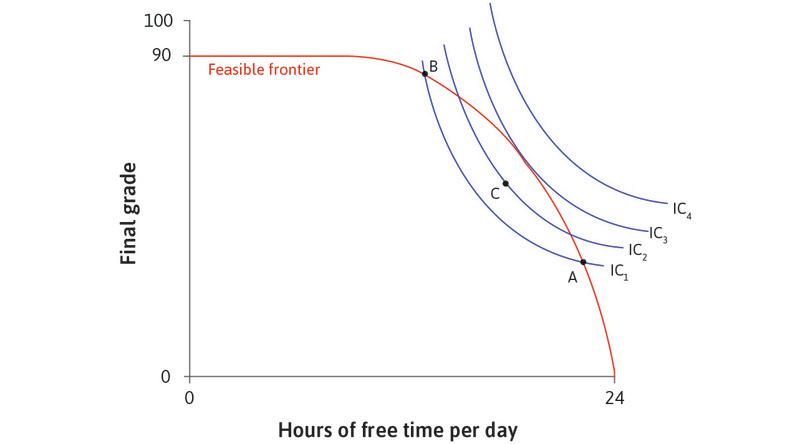
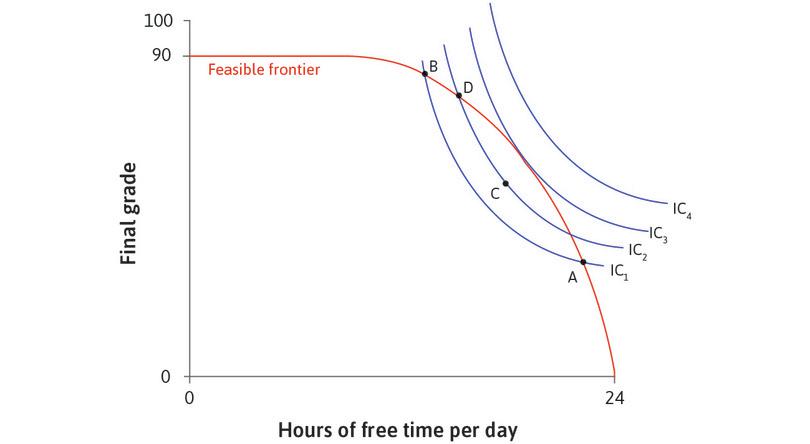
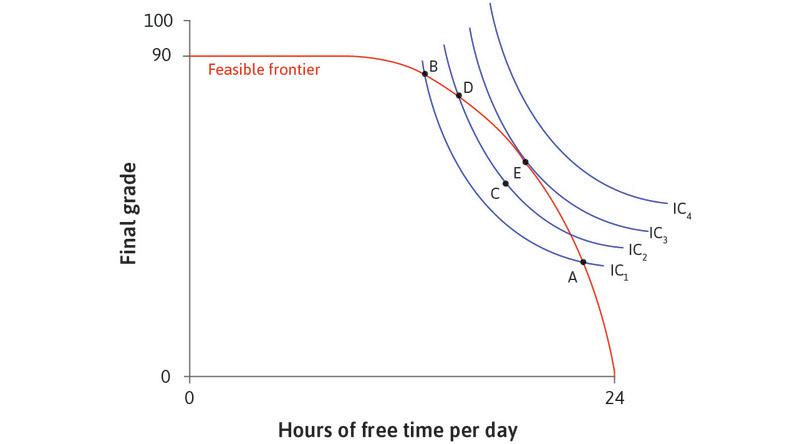
The final step in this decision-making process is to determine the combination of final grade and free time that Alexei will choose. Figure 3.10a brings together his feasible frontier (Figure 3.9) and indifference curves (Figure 3.6). Recall that the indifference curves indicate what Alexei prefers, and their slopes shows the trade-offs that he is willing to make; the feasible frontier is the constraint on his choice, and its slope shows the trade-off he is constrained to make.
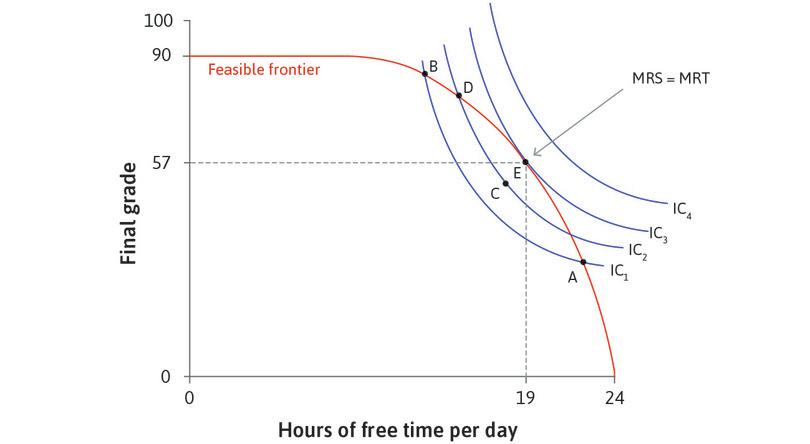
Figure 3.10a shows four indifference curves, labelled IC₁ to IC₄. IC₄ represents the highest level of utility because it is the furthest away from the origin. No combination of grade and free time on IC₄ is feasible, however, because the whole indifference curve lies outside the feasible set. Suppose that Alexei considers choosing a combination somewhere in the feasible set, on IC₁. By working through the steps in Figure 3.10a, you will see that he can increase his utility by moving to points on higher indifference curves until he reaches a feasible choice that maximizes his utility.
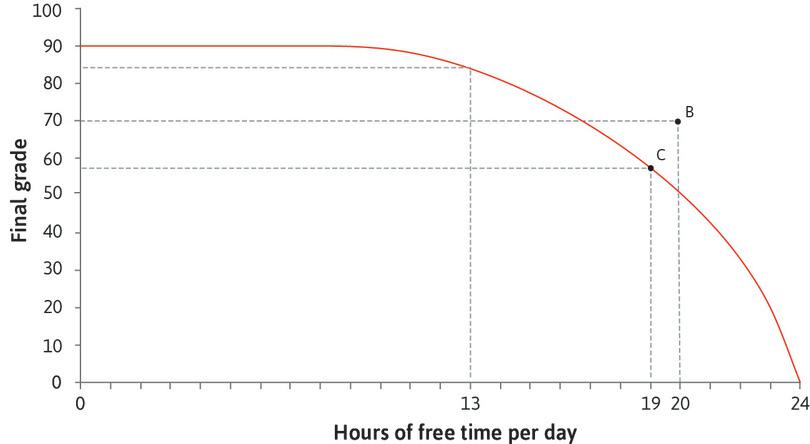
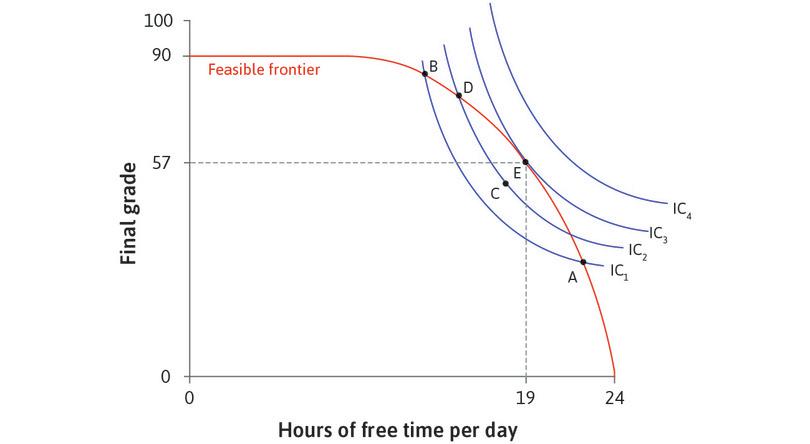
Alexei maximizes his utility at point E, at which his indifference curve is tangent to the feasible frontier. The model predicts that Alexei will:
- choose to spend 5 hours each day studying, and 19 hours on other activities
- obtain a grade of 57 as a result
We can see from Figure 3.10a that at E, the feasible frontier and the highest attainable indifference curve IC₃ are tangent to each other (they touch but do not cross). At E, the slope of the indifference curve is the same as the slope of the feasible frontier. Now, remember that the slopes represent the two trade-offs facing Alexei:
- The slope of the indifference curve is the MRS: It is the trade-off he is willing to make between free time and percentage points.
- The slope of the frontier is the MRT: It is the trade-off that he is constrained to make between free time and percentage points because it is not possible to go beyond the feasible frontier.
Alexei achieves the highest possible utility where the two trade-offs just balance (E). His optimal combination of grade and free time is at the point where the marginal rate of transformation is equal to the marginal rate of substitution.
Leibniz: Optimal allocation of free time: MRT meets MRS
Figure 3.10b shows the MRS (slope of indifference curve) and MRT (slope of feasible frontier) at the points shown in Figure 3.10a. At B and D, the number of points Alexei is willing to trade for an hour of free time (MRS) is greater than the opportunity cost of that hour (MRT), so he prefers to increase his free time. At A, the MRT is greater than the MRS so he prefers to decrease his free time. And, as expected, at E the MRS and MRT are equal.
| B | D | E | A | |
|---|---|---|---|---|
| Free time | 13 | 15 | 19 | 22 |
| Grade | 84 | 78 | 57 | 33 |
| MRT | 2 | 4 | 7 | 9 |
| MRS | 20 | 15 | 7 | 3 |
How many hours does Alexei decide to study?
How many hours does Alexei decide to study?
Figure 3.10b How many hours does Alexei decide to study?
- constrained choice problem
- This problem is about how we can do the best for ourselves, given our preferences and constraints, and when the things we value are scarce. See also: constrained optimization problem.
We have modelled the student’s decision on study hours as what we call a constrained choice problem: a decision-maker (Alexei) pursues an objective (utility maximization in this case) subject to a constraint (his feasible frontier).
In our example, both free time and points in the exam are scarce for Alexei because:
-
Free time and grades are goods: Alexei values both of them.
-
Each has an opportunity cost: More of one good means less of the other.
In constrained choice problems, the solution is the individual’s optimal choice. If we assume that utility maximization is Alexei’s goal, the optimal combination of grade and free time is a point on the feasible frontier at which:
The table in Figure 3.11 summarizes Alexei’s trade-offs.
| The trade-off | Where it is on the diagram | It is equal to … | |
|---|---|---|---|
| MRS | Marginal rate of substitution: The number of percentage points Alexei is willing to trade for an hour of free time | The slope of the indifference curve | |
| MRT, or opportunity cost of free time | Marginal rate of transformation: The number of percentage points Alexei would gain (or lose) by giving up (or taking) another hour of free time | The slope of the feasible frontier | The marginal product of labour |
Alexei’s trade-offs.
Table showing Alexei’s trade-offs.
Figure 3.11 Alexei’s trade-offs.
Exercise 3.6 Exploring scarcity
Describe a situation in which Alexei’s grade points and free time would not be scarce. Remember, scarcity depends on both his preferences and the production function.
Question 3.8 Choose the correct answer(s)
Figure 3.10a shows Alexei’s feasible frontier and his indifference curves for final grade and hours of free time per day. Suppose that all students have the same feasible frontier, but their indifference curves may differ in shape and slope depending on their preferences.
Use the diagram to decide which of the following is (are) correct.
- If Alexei were at a point on the feasible frontier where MRS ? MRT, then he would be willing to give up more of one good than would actually be necessary to get some of the other. Therefore, he will choose to do so until he reaches a point where MRS=MRT.
- Along the feasible frontier, Alexei would be on a higher indifference curve at E than at D. Therefore point D is not the optimal choice.
- Students with flatter indifference curves (more willing to sacrifice more hours of free time for the same number of extra marks) have a lower marginal rate of substitution. Therefore, they will choose bundles to the left of E (such as D) where their indifference curves are tangent to the feasible frontier.
- The points along the feasible frontier to the left of E have higher ratios of final grade per hour of free time, but are not optimal. The optimal point is where marginal rate of substitution equals marginal rate of transformation.
3.6 Hours of work and economic growth
In 1930, John Maynard Keynes, a British economist, published an essay entitled ‘Economic Possibilities for our Grandchildren’, in which he suggested that in the 100 years that would follow, technological improvement would make us, on average, about eight times better off.2 What he called ‘the economic problem, the struggle for subsistence’ would be solved, and we would not have to work more than, say, 15 hours per week to satisfy our economic needs. The question he raised was: how would we cope with all of the additional leisure time?
Keynes’ prediction for the rate of technological progress in countries such as the UK and the US has been approximately right, and working hours have indeed fallen, although much less than he expected—it seems unlikely that average working hours will be 15 hours per week by 2030. An article by Tim Harford in the Undercover Economist column of the Financial Times examines why Keynes’ prediction was wrong.3
As we saw in Unit 2, new technologies raise the productivity of labour. We now have the tools to analyse the effects of increased productivity on living standards, specifically on incomes and the free time of workers.
So far, we have considered Alexei’s choice between studying and free time. We now apply our model of constrained choice to Angela, a self-sufficient farmer who chooses how many hours to work. We assume that Angela produces grain to eat and does not sell it to anyone else. If she produces too little grain, she will starve.
What is stopping her producing the most grain possible? Like the student, Angela also values free time—she gets utility from both free time and consuming grain.
But her choice is constrained: producing grain takes labour time, and each hour of labour means Angela foregoes an hour of free time. The hour of free time sacrificed is the opportunity cost of the grain produced. Like Alexei, Angela faces a problem of scarcity: she has to make a choice between her consumption of grain and her consumption of free time.
To understand her choice, and how it is affected by technological change, we need to model her production function, and her preferences.
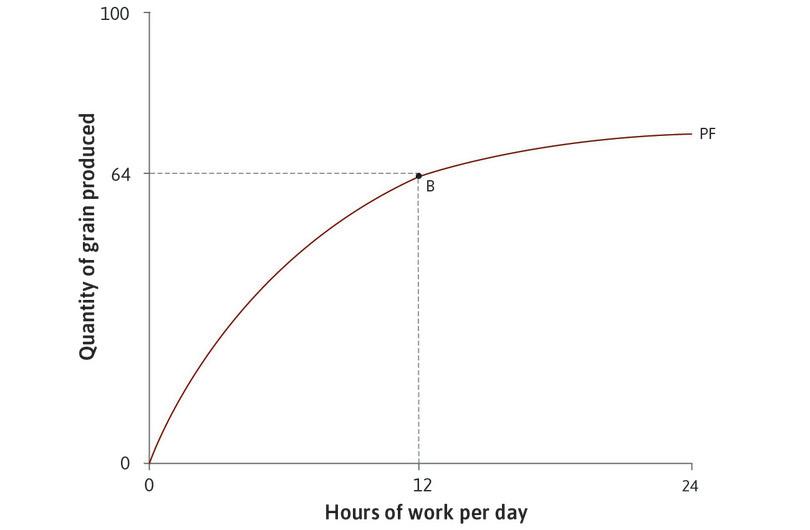
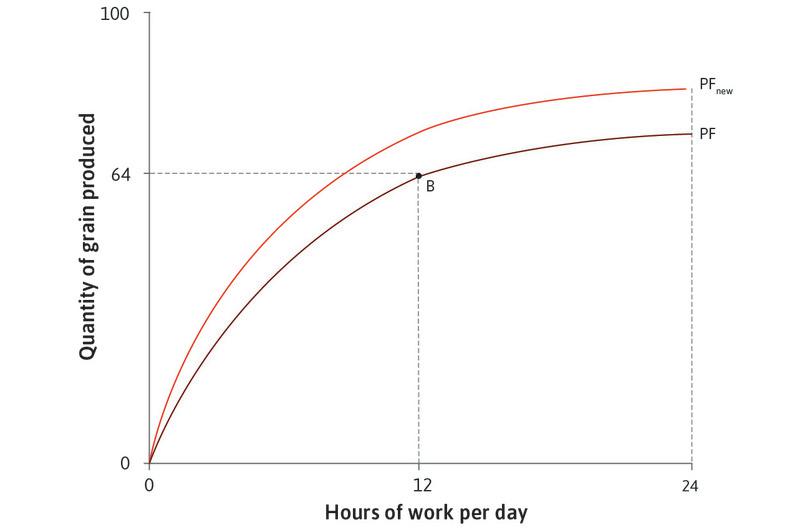
Figure 3.12 shows the initial production function before the change occurs: the relationship between the number of hours worked and the amount of grain produced. Notice that the graph has a similar concave shape to Alexei’s production function: the marginal product of an additional hour’s work, shown by the slope, diminishes as the number of hours increases.
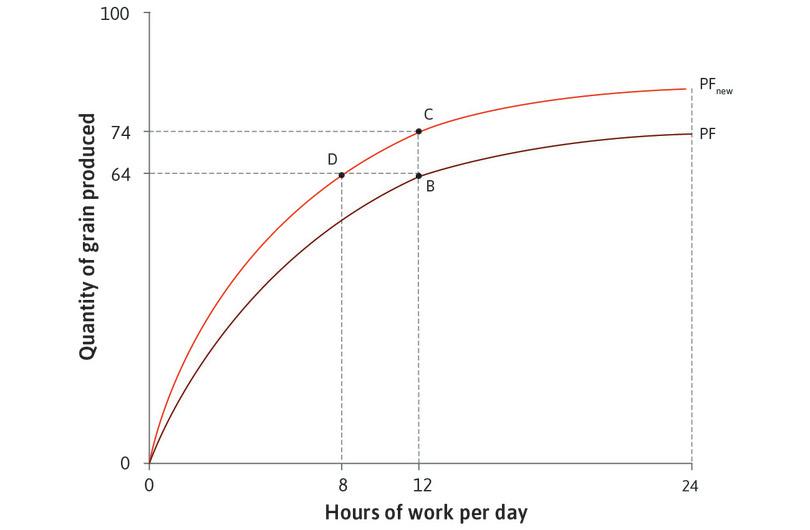
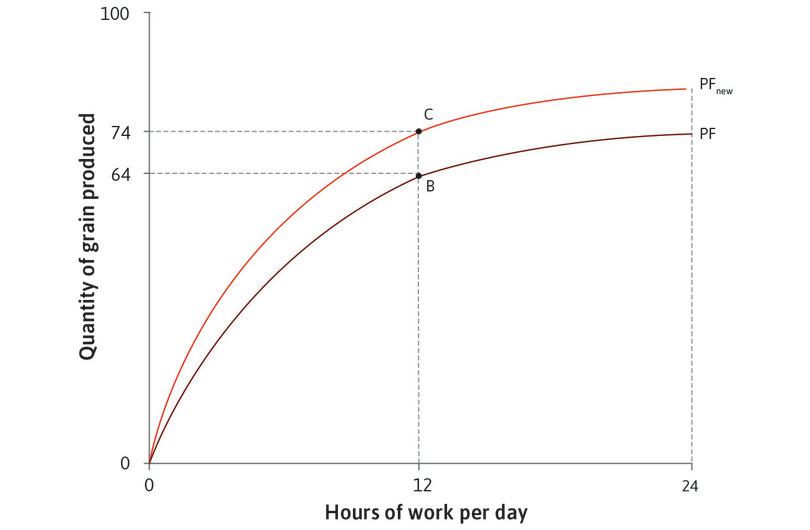
A technological improvement such as seeds with a higher yield, or better equipment that makes harvesting quicker, will increase the amount of grain produced in a given number of hours. The analysis in Figure 3.12 demonstrates the effect on the production function.
Notice that the new production function is steeper than the original one for every given number of hours. The new technology has increased Angela’s marginal product of labour: at every point, an additional hour of work produces more grain than under the old technology.
Leibniz: Modelling technological change
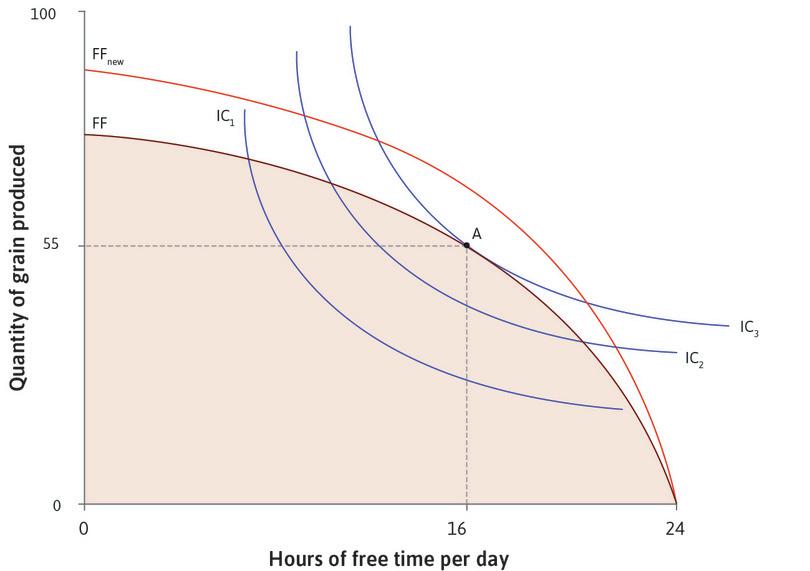
Figure 3.13 shows Angela’s feasible frontier, which is just the mirror image of the production function, for the original technology (FF), and the new one (FFnew).
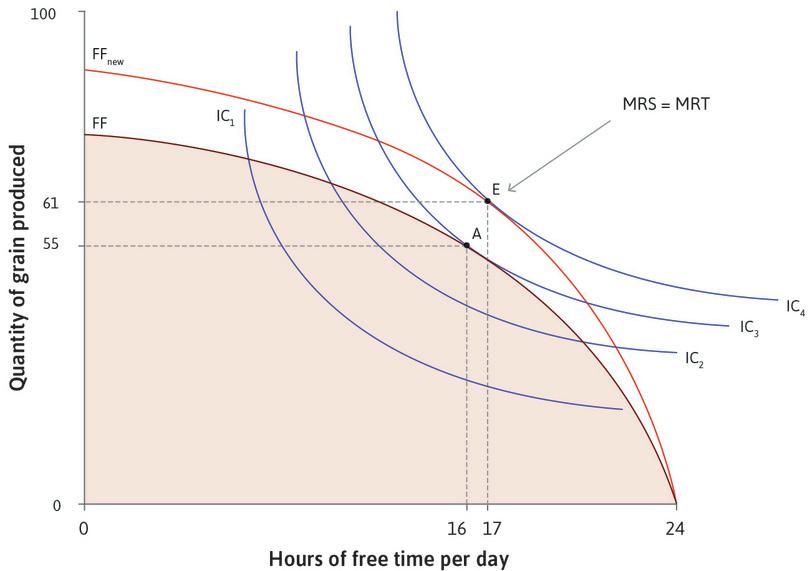
As before, what we call free time is all of the time that is not spent working to produce grain—it includes time for eating, sleeping, and everything else that we don’t count as farm work, as well as her leisure time. The feasible frontier shows how much grain can be consumed for each possible amount of free time. Points B, C, and D represent the same combinations of free time and grain as in Figure 3.12. The slope of the frontier represents the MRT (the marginal rate at which free time can be transformed into grain) or equivalently the opportunity cost of free time. You can see that technological progress expands the feasible set: it gives her a wider choice of combinations of grain and free time.
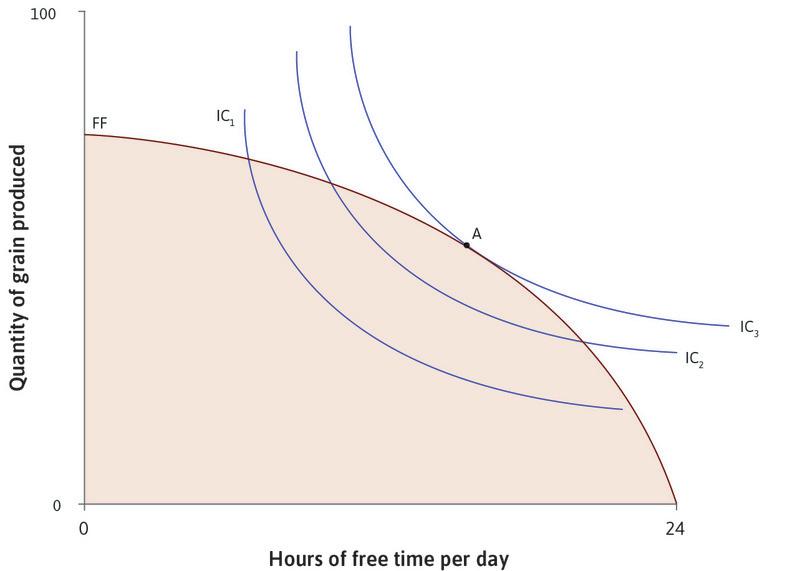
Now we add Angela’s indifference curves to the diagram, representing her preferences for free time and grain consumption, to find which combination in the feasible set is best for her. Figure 3.14 shows that her optimal choice under the original technology is to work for 8 hours a day, giving her 16 hours of free time and 55 units of grain. This is the point of tangency, where her two trade-offs balance out: her marginal rate of substitution (MRS) between grain and free time (the slope of the indifference curve) is equal to the MRT (the slope of the feasible frontier). We can think of the combination of free time and grain at point A as a measure of her standard of living.
Follow the steps in Figure 3.14 to see how her choice changes as a result of technological progress.
Technological change raises Angela’s standard of living: it enables her to achieve higher utility. Note that in Figure 3.14 she increases both her consumption of grain and her free time.
It is important to realize that this is just one possible result. Had we drawn the indifference curves or the frontier differently, the trade-offs Angela faces would have been different. We can say that the improvement in technology definitely makes it feasible to both consume more grain and have more free time, but whether Angela will choose to have more of both depends on her preferences between these two goods, and her willingness to substitute one for the other.
To understand why, remember that technological change makes the production function steeper: it increases Angela’s marginal product of labour. This means that the opportunity cost of free time is higher, giving her a greater incentive to work. But also, now that she can have more grain for each amount of free time, she may be more willing to give up some grain for more free time: that is, reduce her hours of work.
These two effects of technological progress work in opposite directions. In Figure 3.14, the second effect dominates and she chooses point E, with more free time as well as more grain. In the next section, we look more carefully at these two opposing effects, using a different example to disentangle them.
Question 3.9 Choose the correct answer(s)
The figures show Alexei’s production function and his corresponding feasible frontier for final grade and hours of work or free time per day. They show the effect of an improvement in his studying technique, represented by the tilting up of the two curves.
Consider now two cases of further changes in Alexei’s study environment:
Case A. He suddenly finds himself needing to spend 4 hours a day caring for a family member. (You may assume that his marginal product of labour is unaffected for the hours that he studies.)
Case B. For health reasons his marginal product of labour for all hours is reduced by 10%.
Then:
- With Alexei’s marginal product of labour unaffected, the production function remains the same: each number of hours worked yields the same grade as before.
- The feasible frontier shifts to the left and intersects the horizontal axis at 20 hours, since 4 hours a day are now spent on care, so any given number of hours committed to free time per day now corresponds to fewer hours worked and thus a lower mark.
- With the reduction in Alexei’s marginal product of labour, the production function curve becomes flatter. This leads to tilting of the curve inwards, pivoted at the origin.
- The reduction in the marginal product results in a lower mark for every level of hours worked (except at zero), so the feasible frontier pivots around the intercept, rotating downwards.
Exercise 3.7 Your production function
- What could bring about a technological improvement in your production function and those of your fellow students?
- Draw a diagram to illustrate how this improvement would affect your feasible set of grades and study hours.
- Analyse what might happen to your choice of study hours, and the choices that your peers might make.
3.7 Income and substitution effects on hours of work and free time
Imagine that you are looking for a job after you leave college. You expect to be able to earn a wage of $15 per hour. Jobs differ according to the number of hours you have to work—so what would be your ideal number of hours? Together, the wage and the hours of work will determine how much free time you will have, and your total earnings.
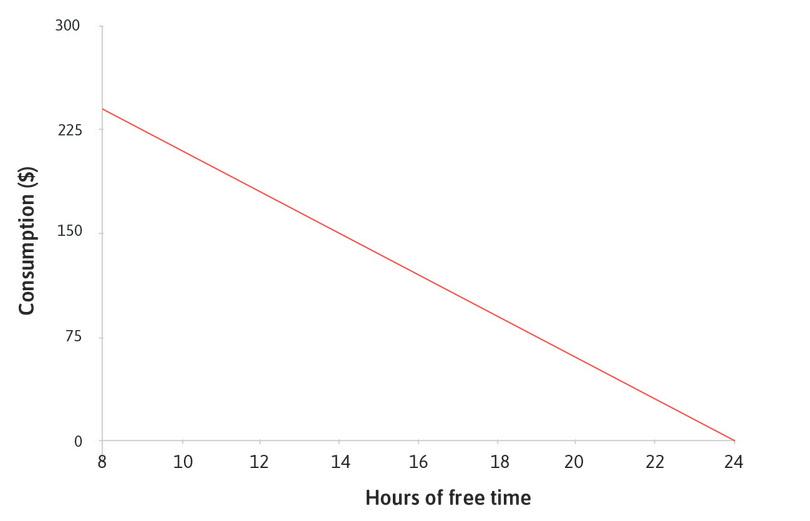
We will work in terms of daily average free time and consumption, as we did for Angela. We will assume that your spending—that is, your average consumption of food, accommodation, and other goods and services—cannot exceed your earnings (for example, you cannot borrow to increase your consumption). If we write w for the wage, and you have t hours of free time per day, then you work for (24 − t) hours, and your maximum level of consumption, c, is given by:
- budget constraint
- An equation that represents all combinations of goods and services that one could acquire that exactly exhaust one’s budgetary resources.
We will call this your budget constraint, because it shows what you can afford to buy.
In the table in Figure 3.15 we have calculated your free time for hours of work varying between 0 and 16 hours per day, and your maximum consumption, when your wage is w = $15.
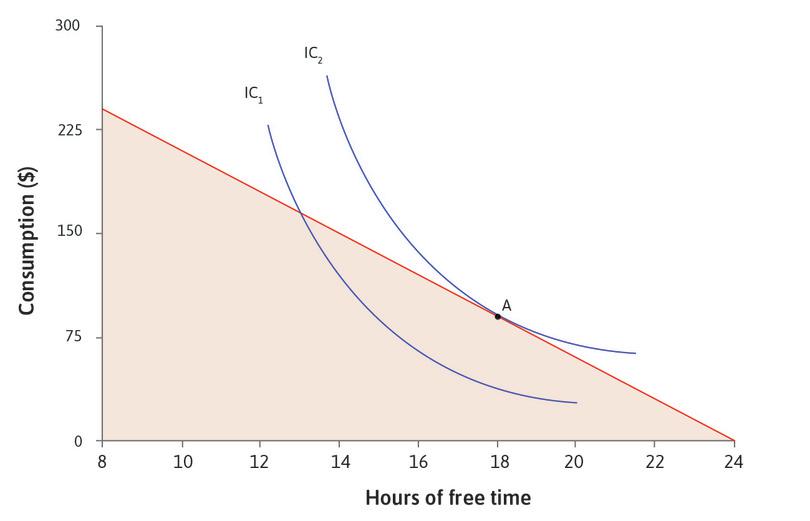
Figure 3.15 shows the two goods in this problem: hours of free time (t) on the horizontal axis, and consumption (c) on the vertical axis. When we plot the points shown in the table, we get a downward-sloping straight line: this is the graph of the budget constraint. The equation of the budget constraint is:
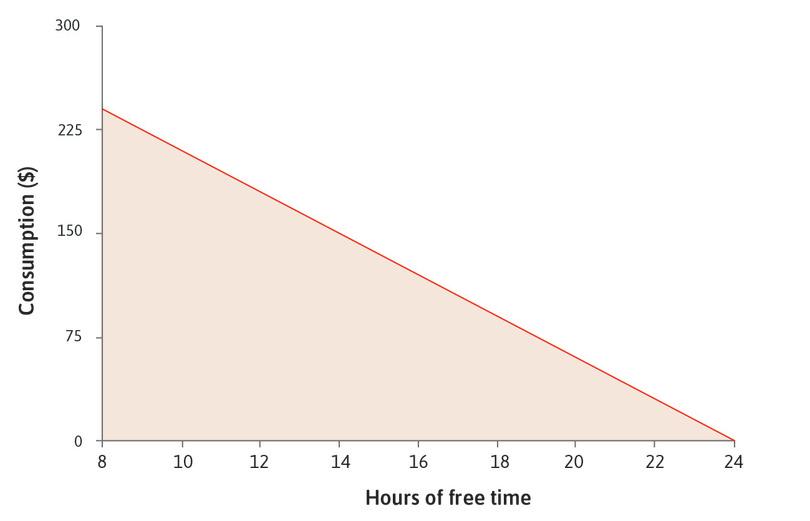
The slope of the budget constraint corresponds to the wage: for each additional hour of free time, consumption must decrease by $15. The area under the budget constraint is your feasible set. Your problem is quite similar to Angela’s problem, except that your feasible frontier is a straight line. Remember that for Angela the slope of the feasible frontier is both the MRT (the rate at which free time could be transformed into grain) and the opportunity cost of an hour of free time (the grain foregone). These vary because Angela’s marginal product changes with her hours of work. For you, the marginal rate at which you can transform free time into consumption, and the opportunity cost of free time, is constant and is equal to your wage (in absolute value): it is $15 for your first hour of work, and still $15 for every hour after that.
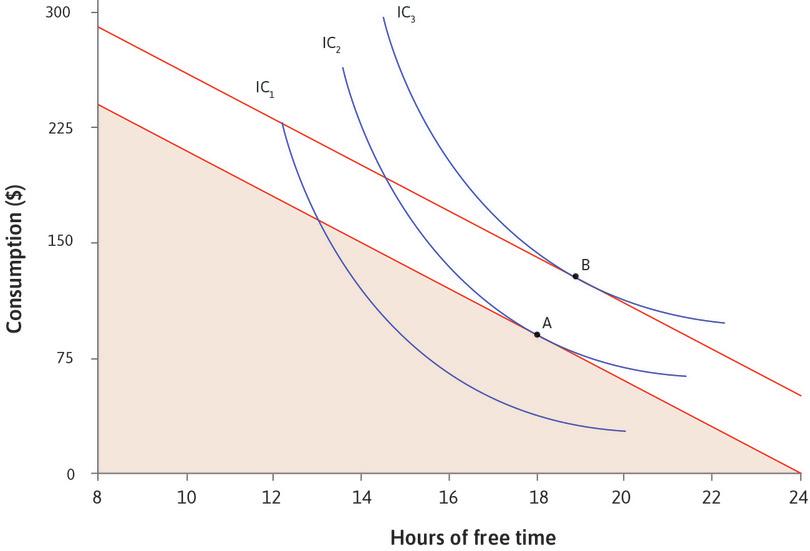
What would be your ideal job? Your preferred choice of free time and consumption will be the combination on the feasible frontier that is on the highest possible indifference curve. Work through Figure 3.15 to find the optimal choice.
If your indifference curves look like the ones in Figure 3.15, then you would choose point A, with 18 hours of free time. At this point your MRS—the rate at which you are willing to swap consumption for time—is equal to the wage ($15, the opportunity cost of time). You would like to find a job in which you can work for 6 hours per day, and your daily earnings would be $90.
Like the student, you are balancing two trade-offs:
| The trade-off | Where it is on the diagram | |
|---|---|---|
| MRS | Marginal rate of substitution: The amount of consumption you are willing to trade for an hour of free time. | The slope of the indifference curve. |
| MRT | Marginal rate of transformation: The amount of consumption you can gain from giving up an hour of free time, which is equal to the wage, w. | The slope of the budget constraint (the feasible frontier) which is equal to the wage. |
Your two trade-offs.
Table showing trade-offs between time and consumption.
Figure 3.16 Your two trade-offs.
Your optimal combination of consumption and free time is the point on the budget constraint where:
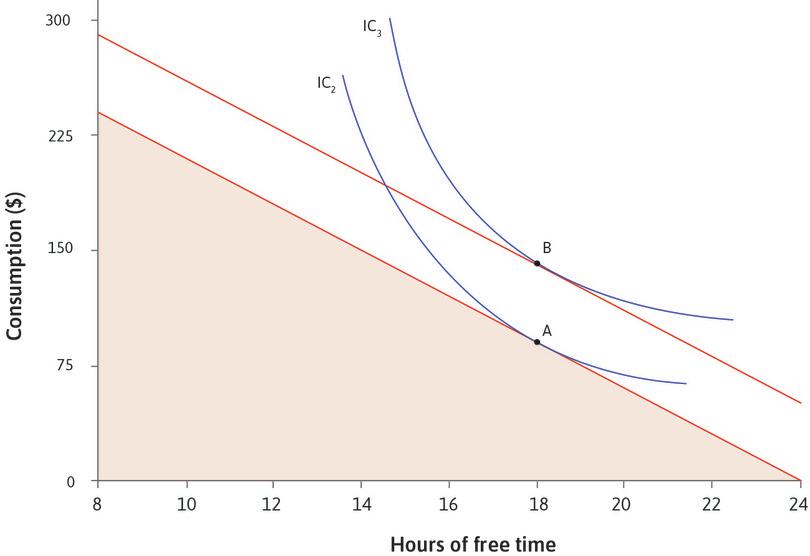
While considering this decision, you receive an email. A mysterious benefactor would like to give you an income of $50 a day—for life (all you have to do is provide your banking details.) You realize at once that this will affect your choice of job. The new situation is shown in Figure 3.17: for each level of free time, your total income (earnings plus the mystery gift) is $50 higher than before. So the budget constraint is shifted upwards by $50—the feasible set has expanded. Your budget constraint is now:
Notice that the extra income of $50 does not change your opportunity cost of time: each hour of free time still reduces your consumption by $15 (the wage). Your new ideal job is at B, with 19.5 hours of free time. B is the point on IC₃ where the MRS is equal to $15. With the indifference curves shown in this diagram, your response to the extra income is not simply to spend $50 more; you increase consumption by less than $50, and you take some extra free time. Someone with different preferences might not choose to increase their free time: Figure 3.18 shows a case in which the MRS at each value of free time is the same on both IC₂ and the higher indifference curve IC₃. This person chooses to keep their free time the same, and consume $50 more.
The effect of additional income for someone whose MRS doesn’t change when consumption rises.
Table showing the effect of additional income for someone whose MRS doesn’t change when consumption rises.
Figure 3.18 The effect of additional income for someone whose MRS doesn’t change when consumption rises.
- income effect
- The effect that the additional income would have if there were no change in the price or opportunity cost.
The effect of additional (unearned) income on the choice of free time is called the income effect. Your income effect, shown in Figure 3.17, is positive—extra income raises your choice of free time. For the person in Figure 3.18, the income effect is zero. We assume that for most goods the income effect will be either positive or zero, but not negative: if your income increased, you would not choose to have less of something that you valued.
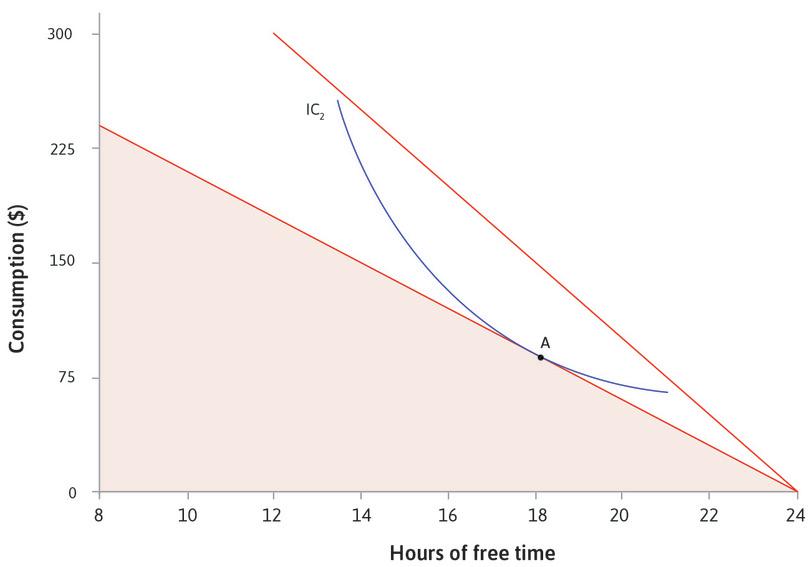
You suddenly realize that it might not be wise to give the mysterious stranger your bank account details (perhaps it is a hoax). With regret you return to the original plan, and find a job requiring 6 hours of work per day. A year later, your fortunes improve: your employer offers you a pay rise of $10 per hour and the chance to renegotiate your hours. Now your budget constraint is:
In Figure 3.19a you can see how the budget constraint changes when the wage rises. With 24 hours of free time (and no work), your consumption would be 0 whatever the wage. But for each hour of free time you give up, your consumption can now rise by $25 rather than $15. So your new budget constraint is a steeper straight line through (24, 0), with a slope equal to $25. Your feasible set has expanded. And now you achieve the highest possible utility at point D, with only 17 hours of free time. So you ask your employer if you can work longer hours—a 7-hour day.
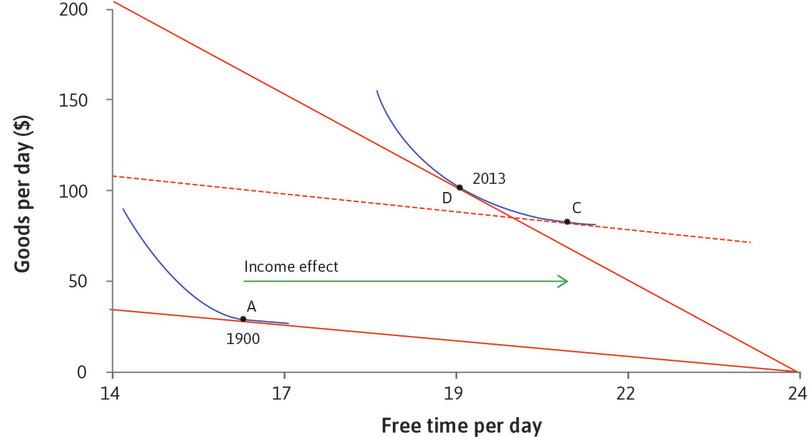
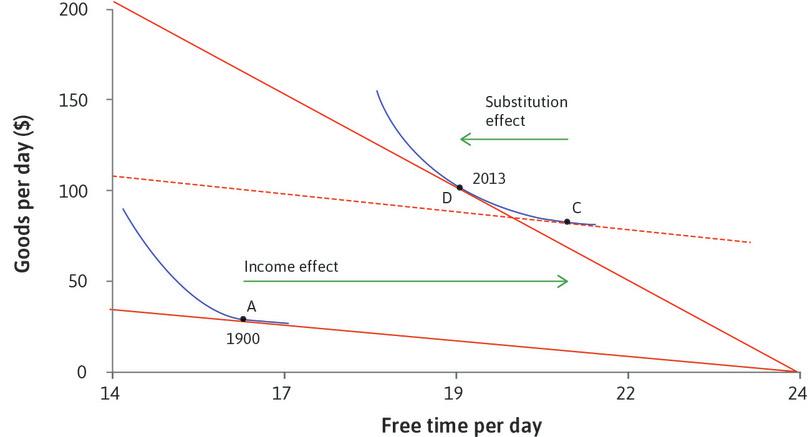
Compare the outcomes in Figure 3.17 and 3.19a. With an increase in unearned income you want to work fewer hours, while the wage increase in Figure 3.19a makes you decide to increase your working hours. Why does this happen? Because there are two effects of a wage increase:
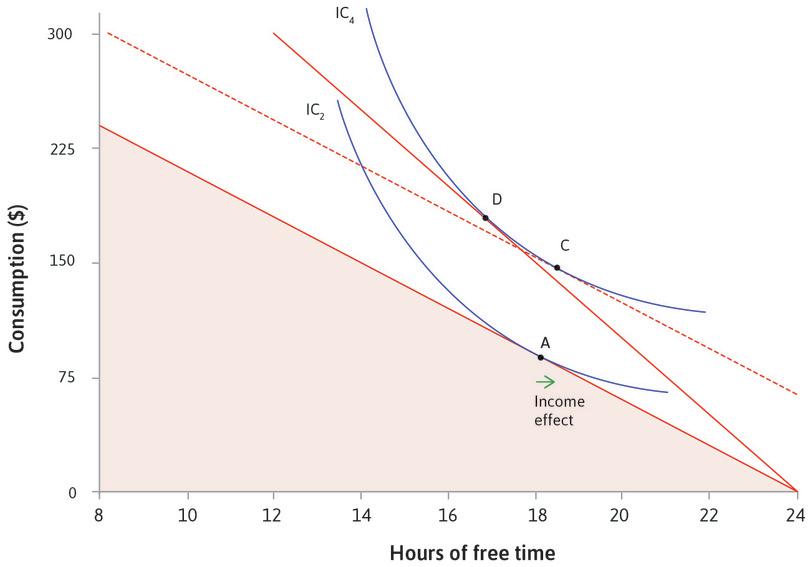
- More income for every hour worked: For each level of free time you can have more consumption, and your MRS is higher: you are now more willing to sacrifice consumption for extra free time. This is the income effect we saw in Figure 3.17—you respond to additional income by taking more free time as well as increasing consumption.
- substitution effect
- The effect that is only due to changes in the price or opportunity cost, given the new level of utility.
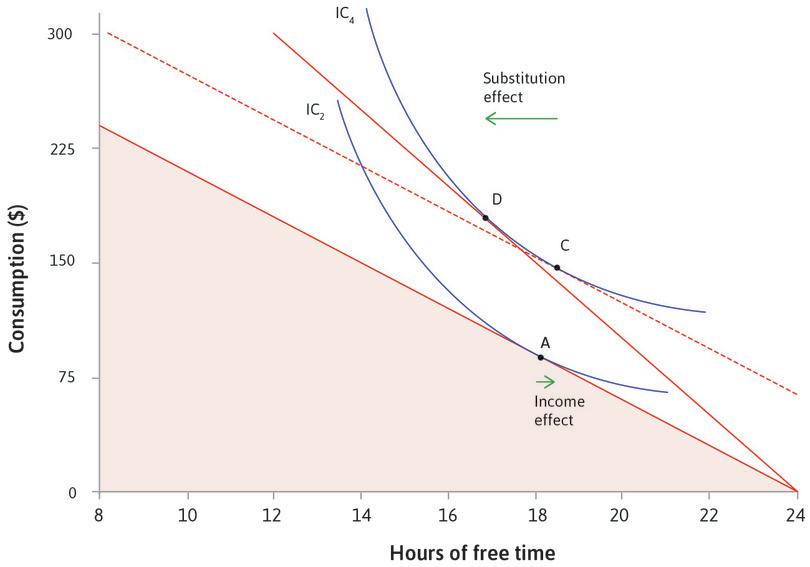
- The budget constraint is steeper: The opportunity cost of free time is now higher. In other words, the marginal rate at which you can transform time into income (the MRT) has increased. And that means you have an incentive to work more—to decrease your free time. This is called the substitution effect.
Leibniz: Mathematics of income and substitution effects
The substitution effect captures the idea that when a good becomes more expensive relative to another good, you choose to substitute some of the other good for it. It is the effect that a change in the opportunity cost would have on its own, for a given utility level.
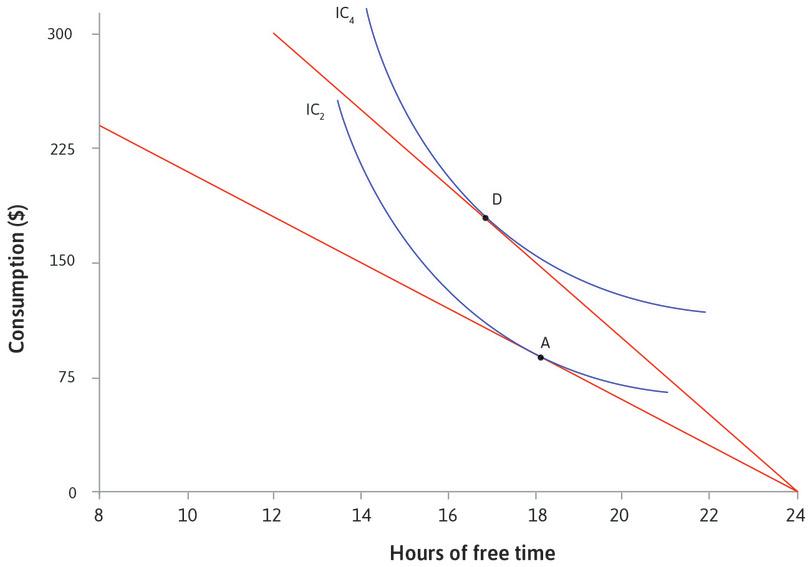
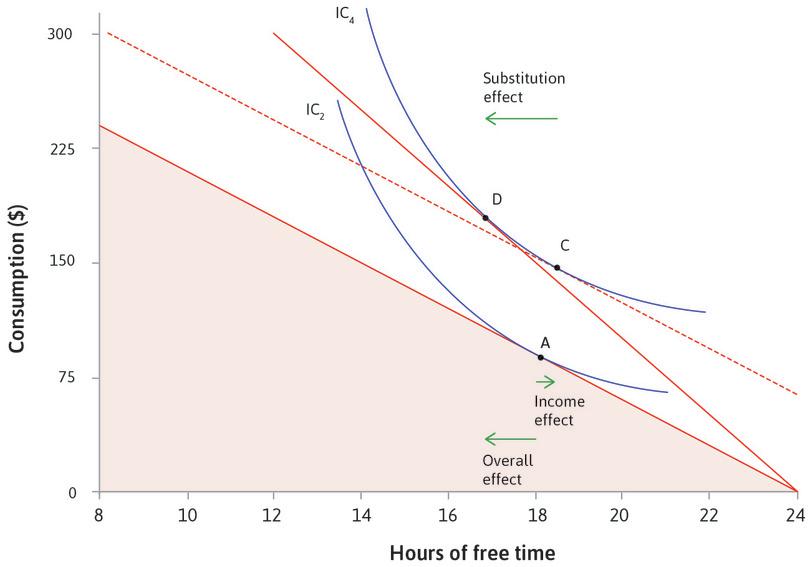
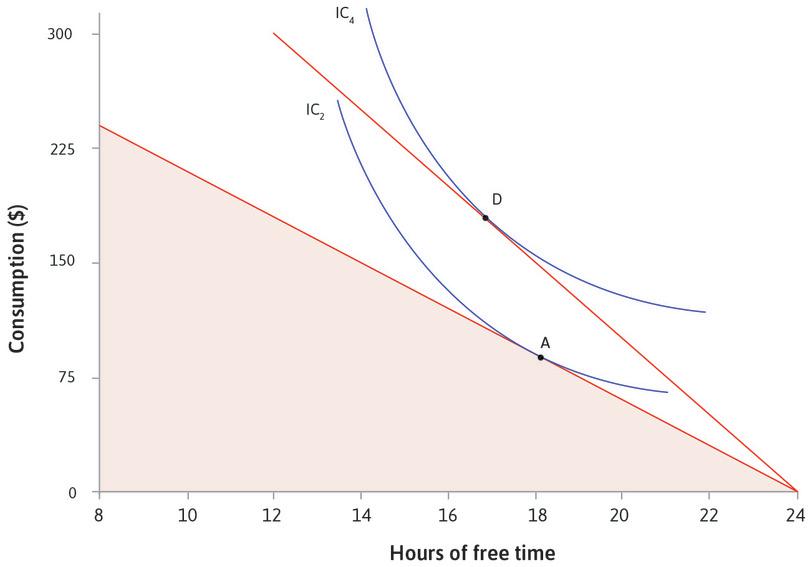
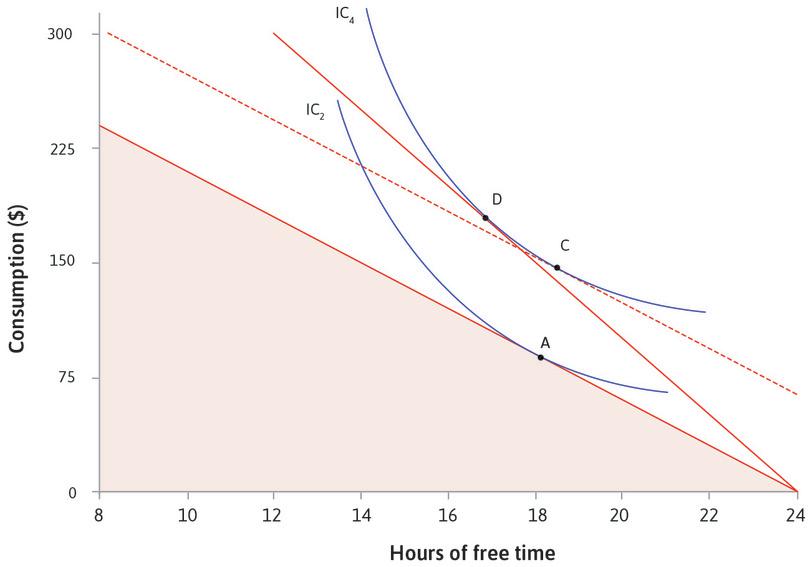
We can show both of these effects in the diagram. Before the wage rise you are at A on IC₂. The higher wage enables you to reach point D on IC₄. Figure 3.19b shows how we can decompose the change from A to D into two parts that correspond to these two effects.
Income and substitution effects
A wage rise:
- raises your income for each level of free time, increasing the level of utility you can achieve
- increases the opportunity cost of free time
So it has two effects on your choice of free time:
- The income effect (because the budget constraint shifts outwards): the effect that the additional income would have if there were no change in the opportunity cost.
- The substitution effect (because the slope of the budget constraint, the MRT, rises): the effect of the change in the opportunity cost, given the new level of utility.
You can see in Figure 3.19b that with indifference curves of this typical shape a substitution effect will always be negative: with a higher opportunity cost of free time you choose a point on the indifference curve with a higher MRS, which is a point with less free time (and more consumption). The overall effect of a wage rise depends on the sum of the income and substitution effects. In Figure 3.19b the negative substitution effect is bigger than the positive income effect, so free time falls.
Technological progress
If you look back at Section 3.6, you will see that Angela’s response to a rise in productivity was also determined by these two opposing effects: an increased incentive to work produced by the rise in the opportunity cost of free time, and an increased desire for free time when her income rises.
We used the model of the self-sufficient farmer to see how technological change can affect working hours. Angela can respond directly to the increase in her productivity brought about by the introduction of a new technology. Employees also become more productive as a result of technological change, and if they have sufficient bargaining power, their wages will rise. The model in this section suggests that, if that happens, technological progress will also bring about a change in the amount of time employees wish to spend working.
The income effect of a higher wage makes workers want more free time, while the substitution effect provides an incentive to work longer hours. If the income effect dominates the substitution effect, workers will prefer fewer hours of work.
Question 3.10 Choose the correct answer(s)
Figure 3.15 depicts your budget constraint when the hourly wage is $15.
Which of the following is (are) true?
- For every additional hour of free time, you have $15 less to spend on consumption, so the slope of the budget constraint is −15.
- The budget constraint is the feasible frontier of the available combinations of free time and consumption. Its slope is constant, so the MRT is constant.
- An increase in the wage rate would make the budget constraint steeper, pivoted at the intercept on the horizontal axis, as every hour of free time would now cost more in foregone consumption.
- A gift would shift the budget constraint outward in a parallel manner, as the consumer could consume more at any given level of free time.
3.8 Is this a good model?
We have looked at three different contexts in which people decide how long to spend working—a student (Alexei), a farmer (Angela), and a wage earner. In each case we have modelled their preferences and feasible set, and the model tells us that their best (utility-maximizing) choice is the level of working hours at which the slope of the feasible frontier is equal to the slope of the indifference curve.
You may have been thinking: this is not what people do!
Billions of people organize their working lives without knowing anything about MRS and MRT (if they did make decisions that way, perhaps we would have to subtract the hours they would spend making calculations). And even if they did make their choice using mathematics, most of us can’t just leave work whenever we want. So how can this model be useful?
Remember from Unit 2 that models help us ‘see more by looking at less’. Lack of realism is an intentional feature of this model, not a shortcoming.
Trial and error replaces calculations
Can a model that ignores how we think possibly be a good model of how we choose?
Milton Friedman, an economist, explained that when economists use models in this way they do not claim that we actually think through these calculations (such as equating MRS to MRT) each time we make a decision. Instead we each try various choices (sometimes not even intentionally) and we tend to adopt habits, or rules of thumb that make us feel satisfied and not regret our decisions.
In his book Essays in positive economics, he described it as similar to playing billiards (pool):
Consider the problem of predicting the shots made by an expert billiard player. It seems not at all unreasonable that excellent predictions would be yielded by the hypothesis that the billiard player made his shots as if he knew the complicated mathematical formulas that would give the optimum directions of travel, could estimate accurately by eye the angles, etc., describing the location of the balls, could make lightning calculations from the formulas, and could then make the balls travel in the direction indicated by the formulas.
Our confidence in this hypothesis is not based on the belief that billiard players, even expert ones, can or do go through the process described. It derives rather from the belief that, unless in some way or other they were capable of reaching essentially the same result, they would not in fact be expert billiard players.4
Similarly, if we see a person regularly choosing to go to the library after lectures instead of going out, or not putting in much work on their farm, or asking for longer shifts after a pay rise, we do not need to suppose that this person has done the calculations we set out. If that person later regretted the choice, next time they might go out a bit more, work harder on the farm, or cut their hours back. Eventually we could speculate they might end up with a decision on work time that is close to the result of our calculations.
That is why economic theory can help to explain, and sometimes even predict, what people do—even though those people are not performing the mathematical calculations that economists make in their models.
The influence of culture and politics
A second unrealistic aspect of the model: employers typically choose working hours, not individual workers, and employers often impose a longer working day than workers prefer. As a result, the hours that many people work are regulated by law, so that beyond some maximum amount neither the employee nor the employer can choose to work. In this case the government has limited the feasible set of hours and goods.
Although individual workers often have little freedom to choose their hours, it may nevertheless be the case that changes in working hours over time, and differences between countries, partly reflect the preferences of workers. If many individual workers in a democracy wish to lower their hours, they may ‘choose’ this indirectly as voters, if not individually as workers. Or they may bargain as members of a trade union for contracts requiring employers to pay higher overtime rates for longer hours.
This explanation stresses culture (meaning changes in preferences or differences in preferences among countries) and politics (meaning differences in laws, or trade union strength and objectives). They certainly help to explain differences in working hours between countries:
Cultures seem to differ. Some northern European cultures highly value their vacation times, while South Korea is famous for the long hours that employees put in. Legal limits on working time differ. In Belgium and France the normal work week is limited to 35–39 hours, while in Mexico the limit is 48 hours and in Kenya even longer.
But, even on an individual level, we may influence the hours we work. For example, employers who advertise jobs with the working hours that most people prefer may find they have more applicants than other employers offering too many (or too few) hours.
Remember, we also judge the quality of a model by whether it provides insight into something that we want to understand. In the next section, we will look at whether our model of the choice of hours of work can help us understand why working hours differ so much between countries and why, as we saw in the introduction, they have changed over time.
Exercise 3.8 Another definition of economics
Lionel Robbins, an economist, wrote in 1932 that: ‘Economics is the science that studies human behaviour as a relationship between given ends and scarce means which have alternative uses.’5
- Give an example from this unit to illustrate the way that economics studies human behaviour as a relationship between ‘given ends and scarce means with alternative uses’.
- Are the ‘ends’ of economic activity, that is, the things we desire, fixed? Use examples from this unit (study time and grades, or working time and consumption) to illustrate your answer.
- The subject matter that Robbins refers to—doing the best you can in a given situation—is an essential part of economics. But is economics limited to the study of ‘scarce means which have alternative uses’? In answering this question, include a contrast between Robbins’ definition and the one given in Unit 1, and note that Robbins wrote this passage at a time when 15% of the British workforce was unemployed.
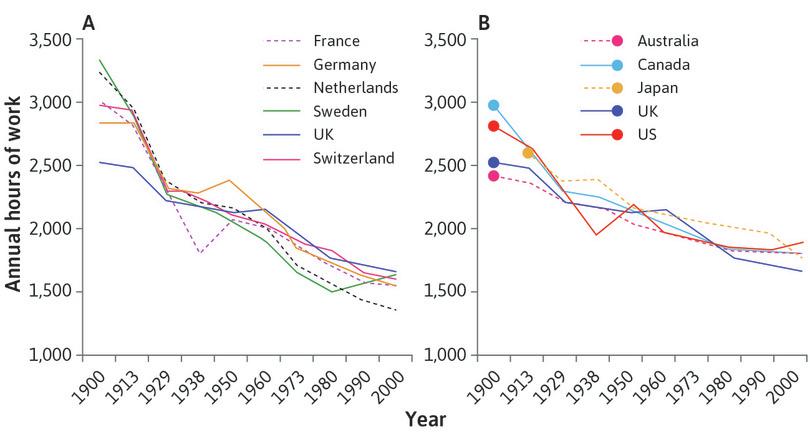
3.9 Explaining our working hours: Changes over time
During the year 1600, the average British worker was at work for 266 days. This statistic did not change much until the Industrial Revolution. Then, as we know from the previous unit, wages began to rise, and working time rose too: to 318 days in 1870.
Meanwhile, in the US, hours of work increased for many workers who shifted from farming to industrial jobs. In 1865 the US abolished slavery, and former slaves used their freedom to work much less. From the late nineteenth century until the middle of the twentieth century, working time in many countries gradually fell. Figure 3.1 at the beginning of this unit showed how annual working hours have fallen since 1870 in the Netherlands, the US and France.6
The simple models we have constructed cannot tell the whole story. Remember that the ceteris paribus assumption can omit important details: things that we have held constant in models may vary in real life.
As we explained in the previous section, our model omitted two important explanations, which we called culture and politics. Our model provides another explanation: economics.
Look at the two points in Figure 3.20, giving estimates of average amounts of daily free time and goods per day for employees in the US in 1900 and in 2013. The slopes of the budget constraints through points A and D are the real wage (goods per hour) in 1900 and in 2013. This shows us the feasible sets of free time and goods that would have made these points possible. Then we consider the indifference curves of workers that would have led workers to choose the hours they did. We cannot measure indifference curves directly: we must use our best guess of what the preferences of workers would have been, given the actions that they took.
How does our model explain how we got from point A to point D? You know from Figure 3.19b that the increase in wages would lead to both an income effect and a substitution effect. In this case, the income effect outweighs the substitution effect, so both free time and goods consumed per day go up. Figure 3.20 is thus simply an application to history of the model illustrated in Figure 3.19b. Work through the steps to see the income and substitution effects.
How could reasoning in this way explain the other historical data that we have?
First, consider the period before 1870 in Britain, when both working hours and wages rose:
- Income effect: At the relatively low level of consumption in the period before 1870, workers’ willingness to substitute free time for goods did not increase much when rising wages made higher consumption possible.
- Substitution effect: But they were more productive and paid more, so each hour of work brought more rewards than before in the form of goods, increasing the incentive to work longer hours.
- Substitution effect dominated: Therefore before 1870 the negative substitution effect (free time falls) was bigger than the positive income effect (free time rises), so work hours rose.
During the twentieth century we saw rising wages and falling working hours. Our model accounts for this change as follows:
- Income effect: By the late nineteenth century workers had a higher level of consumption and valued free time relatively more—their marginal rate of substitution was higher—so the income effect of a wage rise was larger.
- Substitution effect: This was consistent with the period before 1870.
- Income effect now dominates: When the income effect began to outweigh the substitution effect, working time fell.
We should also consider the possibility that preferences change over time. If you look carefully at Figure 3.1 you can see that in the last part of the twentieth century hours of work rose in the US, even though wages hardly increased. Hours of work also rose in Sweden during this period.
The economist Thorsten Veblen (1857–1929) coined the term ‘conspicuous consumption’ in his explanation of why people of lesser means try to mimic the consumption habits of the rich.
Thorstein Veblen. 2007. Theory of the leisure class. Oxford: Oxford University Press.
- conspicuous consumption
- The purchase of goods or services to publicly display one’s social and economic status.
Why? Perhaps Swedes and Americans came to value consumption more over these years. In other words, their preferences changed so that their MRS fell (they became more like today’s South Korean workers). This may have occurred because in both the US and Sweden the share of income gained by the very rich increased considerably, and the lavish consumption habits of the rich set a higher standard for everyone else. According to this explanation, Swedes and Americans were ‘keeping up with the Joneses’ and the Joneses got richer, leading everyone else to change their preferences.
The combined political, cultural and economic influences on our choices may produce some surprising trends. In our ‘Economist in action’ video, Juliet Schor, a sociologist and economist who has written about the paradox that many of the world’s wealthiest people are working more despite gains in technology, asks what this means for our quality of life, and for the environment.
Question 3.11 Choose the correct answer(s)
Figure 3.20 depicts a model of labour supply and consumption for the US in 1900 and 2013. The wage rate is shown to have increased between the two years.
Which of the following are true?
- The substitution effect is the pure effect of the change in the slope of the budget constraint. The movement from A to D is the combined effect of the substitution and income effects.
- The income effect is the effect of a higher income on the choice of free time, which is shown by the parallel shift outwards of the budget constraint, and thus a move from A to C.
- With the indifference curves shown, the income effect of the wage increase is greater than the substitution effect, so overall free time increases and hours of work fall.
- With different indifference curves, the substitution effect could have dominated the income effect, leading to a reduction of free time between 1900 and 2013.
What about the future? The high-income economies will continue to experience a major transformation: the declining role of work in the course of our lifetimes. We go to work at a later age, stop working at an earlier age of our longer lives, and spend fewer hours at work during our working years. Robert Fogel, an economic historian, estimated the total working time, including travel to and from work and housework, in the past. He made projections for the year 2040, defining what he called discretionary time as 24 hours a day minus the amount we all need for biological maintenance (sleeping, eating and personal hygiene). Fogel calculated leisure time as discretionary time minus working time.7
Estimated lifetime hours of work and leisure (1880, 1995, 2040).
Estimated lifetime hours of work and leisure (1880, 1995, 2040).
Figure 3.21 Estimated lifetime hours of work and leisure (1880, 1995, 2040).
Robert William Fogel. 2000. The Fourth Great Awakening and the Future of Egalitarianism. Chicago: University of Chicago Press.
In 1880 he estimated that lifetime leisure time was just a quarter of lifetime work hours. In 1995 leisure time exceeded working time over a person’s entire life. He predicted that lifetime leisure would be three times of lifetime working hours by the year 2040. His estimates are in Figure 3.21.
We do not yet know if Fogel has overstated the future decline in working time, as Keynes once did. But he certainly is right that one of the great changes brought about by the technological revolution is the vastly reduced role of work in the life of an average person.
Exercise 3.9 Scarcity and choice
- Do our models of scarcity and choice provide a plausible explanation for the observed trends in working hours during the twentieth century?
- What other factors, not included in the model, might be important in explaining what has happened?
- Remember Keynes’ prediction that working hours would fall to 15 hours per week in the century after 1930. Why do you think working hours have not changed as he expected? Have people’s preferences changed? The model focuses on the number of hours workers would choose, so do you think that many employees are now working longer than they would like?
- In his essay, Keynes said that people have two types of economic needs or wants: absolute needs that do not depend on the situation of other fellow humans, and relative needs—which he called ‘the desire for superiority’. The phrase ‘keeping up with the Joneses’ captures a similar idea that our preferences could be affected by observing the consumption of others. Could relative needs help to explain why Keynes was so wrong about working hours?
3.10 Explaining our working hours: Differences between countries
Figure 3.2 showed that in countries with higher income (GDP per capita) workers tend to have more free time, but also that there are big differences in annual hours of free time between countries with similar income levels. To analyse these differences using our model, we need a different measure of income that corresponds more closely to earnings from employment. The table in Figure 3.22 shows working hours for five countries, together with the disposable income of an average employee (based on the taxes and benefits for a single person without children).
From these figures we have calculated annual free time, and the average wage (by dividing annual income by annual hours worked). Finally, free time per day and daily consumption are calculated by dividing annual free time and earnings by 365.
| Country | Average annual hours worked per employee | Average annual disposable income (single person, no children) | Average annual free time | Wage (disposable income per hour worked) | Freetime per day | Consumption per day |
|---|---|---|---|---|---|---|
| US | 1,789 | 36,737 | 6,971 | 20.54 | 19.10 | 100.65 |
| South Korea | 2,163 | 39,686 | 6,597 | 18.35 | 18.07 | 108.73 |
| Netherlands | 1,383 | 40,171 | 7,377 | 29.05 | 20.21 | 110.06 |
| Turkey | 1,855 | 17,118 | 6,905 | 9.23 | 18.92 | 46.90 |
| Mexico | 2,226 | 11,046 | 6,534 | 4.96 | 17.90 | 30.26 |
Free time and consumption per day across countries (2013).
Table showing free time and consumption in the US, South Korea, the Netherlands, Turkey and Mexico.
Figure 3.22 Free time and consumption per day across countries (2013).
OECD. Average annual hours actually worked per worker. Accessed June 2016. Net income after taxes calculated in US dollars using PPP exchange rates.
Figure 3.23 shows how we might use this data, with the model of Section 3.7, to understand the differences between the countries. From the data in Figure 3.22, we have plotted daily consumption and free time for a typical worker in each country, with the corresponding budget constraint constructed as before, using a line through (24, 0) with slope equal to the wage. We have no information about the preferences of workers in each country, and we don’t know whether the combinations in the diagram can be interpreted as a choice made by the workers. But, if we assume that they do reflect the workers’ choices, we can consider what the data tells us about the preferences of workers in different countries.
From Figure 3.23, we see that average free time in Mexico and South Korea were virtually the same, although the wage was much higher in South Korea than in Mexico. South Koreans, Americans and Dutch people have about as much to spend per day, but South Koreans have three hours less of free time. Could it be that South Koreans have the same preferences as Americans, so that if the wage increased in South Korea they would make the same choice? This seems unlikely: the substitution effect would lead them to consume more goods and take less free time, and it is implausible to suppose that the income effect of a wage rise would lead them to consume fewer goods. More plausible is the hypothesis that South Koreans and Americans (on average) have different preferences. Follow the steps in Figure 3.23 to see some hypothetical indifference curves that could explain the differences among countries. Notice that the indifference curves for the US and for South Korea cross. This means that South Koreans and Americans must have different preferences.
Point Q in the last step of the figure is the point of intersection of the two indifference curves shown for South Korea and the US. At that point the US indifference curve is steeper than the South Korean one. This means that the average American is willing to give up more units of daily goods for an hour of free time (this is the MRS) than the average South Korean, which is consistent with the idea that South Koreans work exceptionally hard. So it may be important to take account of differences in preferences among countries, or among individuals.
Exercise 3.10 Preferences and culture
Suppose that the points plotted in Figure 3.23 reflect the choices of free time and consumption made by workers in these five countries according to our model.
- Is it possible that people in Turkey and the US have the same preferences? If so, how will a wage rise in Turkey affect consumption and free time? What does this imply about the income and substitution effects?
- Suppose that people in Turkey and South Korea have the same preferences. In that case, what can you say about the income and substitution effects of a wage increase?
- If wages in South Korea increased, would you expect consumption there to be higher or lower than in the Netherlands? Why?
Exercise 3.11 Working hours across countries and time
The figure below illustrates what has happened to working hours in many countries during the twentieth century (the UK is in both charts to aid comparison).
Michael Huberman and Chris Minns. 2007. ‘The times they are not changin’: Days and hours of work in Old and New Worlds, 1870–2000’. Explorations in Economic History 44 (4): pp. 538–567.
- How would you describe what happened?
- How are the countries in Panel A of the figure different from those in Panel B?
- What possible explanations can you suggest for why the decline in working hours was greater in some countries than in others?
- Why do you think that the decline in working hours is faster in most countries in the first half of the century?
- In recent years, is there any country in which working hours have increased? Why do you think this happened?
3.11 Conclusion
We have used a model of decision making under scarcity to analyse choices of hours of work, and understand why working hours have fallen over the last century. People’s preferences with respect to goods and free time are described by indifference curves, and their production function (or budget constraint) determines their feasible set. The choice that maximizes utility is a point on the feasible frontier where the marginal rate of substitution (MRS) between goods and free time is equal to the marginal rate of transformation (MRT).
An increase in productivity or wages alters the MRT, raising the opportunity cost of free time. This provides an incentive to work longer hours (the substitution effect). But higher income may increase the desire for free time (the income effect). The overall change in hours of work depends on which of these effects is bigger.
Concepts introduced in Unit 3
Before you move on, review these definitions:
3.12 References
- Fogel, Robert William. 2000. The Fourth Great Awakening and the Future of Egalitarianism. Chicago: University of Chicago Press.
- Friedman, Milton. 1953. Essays in Positive Economics. Chicago: University of Chicago Press.
- Harford, Tim. 2015. ‘The rewards for working hard are too big for Keynes’s vision’. The Undercover Economist. First published by The Financial Times. Updated 3 August 2015.
- Keynes, John Maynard. 1963. ‘Economic Possibilities for our Grandchildren’. In Essays in Persuasion, New York, NY: W. W. Norton & Co.
- Plant, E. Ashby, K. Anders Ericsson, Len Hill, and Kia Asberg. 2005. ‘Why study time does not predict grade point average across college students: Implications of deliberate practice for academic performance’. Contemporary Educational Psychology 30 (1): pp. 96–116.
- Robbins, Lionel. 1984. An Essay on the Nature and Significance of Economic Science. New York: New York University Press.
- Schor, Juliet B. 1992. The Overworked American: The Unexpected Decline Of Leisure. New York, NY: Basic Books.
- Veblen, Thorstein. 2007. The Theory of the Leisure Class. Oxford: Oxford University Press.
- Whaples, Robert. 2001. ‘Hours of work in U.S. History’. EH.Net Encyclopedia.
-
Elizabeth Ashby Plant, Karl Anders Ericsson, Len Hill, and Kia Asberg. 2005. ‘Why study time does not predict grade point average across college students: Implications of deliberate practice for academic performance.’ Contemporary Educational Psychology 30 (1): pp. 96–116. ↩
-
John Maynard Keynes. 1963. ‘Economic Possibilities for our Grandchildren’. In Essays in Persuasion, New York, NY: W. W. Norton & Co. ↩
-
Tim Harford. 2015. ‘The rewards for working hard are too big for Keynes’s vision’. The Undercover Economist. First published by The Financial Times. Updated 3 August 2015. ↩
-
Milton Friedman. 1953. Essays in positive economics, 7th ed. Chicago: University of Chicago Press. ↩
-
Lionel Robbins. 1984. An essay on the nature and significance of economic science, 3rd ed. New York: New York University Press. ↩
-
Robert Whaples. 2001. ‘Hours of work in U.S. History’ EH.Net Encyclopedia. ↩
-
Robert William Fogel. 2000. The fourth great awakening and the future of egalitarianism: The political realignment of the 1990s and the fate of egalitarianism. Chicago: University of Chicago Press. ↩