Leibniz

3.2.1 Indifference curves and the marginal rate of substitution

Alexei cares about his exam grade and his free time. We have seen that his preferences can be represented graphically using indifference curves, and that his willingness to trade off grade points for free time—his marginal rate of substitution—is represented by the slope of the indifference curve. Here we show how to represent his preferences mathematically.

utility

A numerical indicator of the value that one places on an outcome, such that higher valued outcomes will be chosen over lower valued ones when both are feasible.

Remember that an indifference curve joins together combinations of grade points and free time that give Alexei the same amount of utility. Preferences can be represented mathematically by writing down a utility function, which tells us how a person’s ‘units of utility’ depend on the goods available. Alexei only cares about two goods: his hours of free time and his exam grade. If he has $t$ units of free time and $y$ grade points, his utility is given by a function:

$$U(t,\ y)$$

Since both grade and free time are goods—Alexei would like to have as much of each as possible—the utility function must have the property that increasing either $t$ or $y$ would increase $U$. In this case, we say that utility depends positively on $t$ and $y$.

indifference curve

A curve of the points which indicate the combina­tions of goods that provide a given level of utility to the individual.

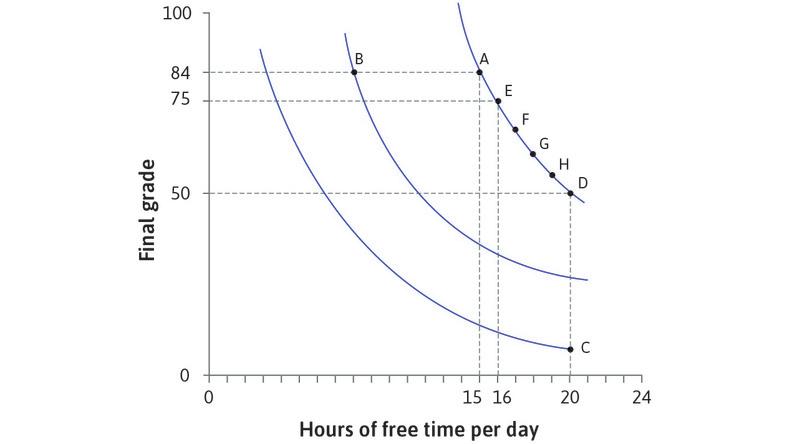
Alexei’s utility function has two arguments. Just as a function of one variable may be represented graphically by a curve on a plane, a function of two variables may be represented by a surface in three-dimensional space. Since three-dimensional diagrams are awkward to handle, economists analyse utility graphically using the same technique that is used to represent the three-dimensional space we live in: a contour map. Contours are lines joining points of equal height above sea level. Similarly, indifference curves are the contours of the utility surface, joining points of equal utility.

In Alexei’s case, an indifference curve shows all the combinations of free time and exam grade that give him the same level of utility. The equation of a typical indifference curve is:

$$U(t,\ y)=c$$

where the constant $c$ stands for the utility level achieved on the curve. Different values of $c$ correspond to different indifference curves: if we increase $c$ we obtain a new indifference curve that is above and to the right of the old one. You can see three of Alexei’s indifference curves in Figure 3.6 of the text, which we reproduce as Figure 1 below.

The marginal rate of substitution

Given any combination $(t,\ y)$ of free time and grade, Alexei’s marginal rate of substitution (MRS) (that is, his willingness to trade grade points for an extra hour of free time) is given by the slope of the indifference curve $U(t,\ y)=c$ through that point.

How can we calculate the slope of the indifference curve $U(t,\ y)=c$?

To do this, we need to use the partial derivatives of the utility function. For example, $\partial U/\partial t$ captures how utility changes as $(t)$ increases, holding $y$ constant. In economics the partial derivative $\partial U/\partial t$ is called the marginal utility of free time. Similarly $\partial U/\partial y$ is the marginal utility of grade points. We have already noted that utility depends positively on $t$ and $y$. In other words, Alexei’s marginal utilities are both positive.

We calculate the slope of the indifference curve using a technique called implicit differentiation, which we shall meet again in later Leibnizes. In the present case, the method involves considering how exam grades would need to change if free time increased by a small amount, in order to keep utility constant.

Suppose both $t$ and $y$ change by small amounts $\Delta t$ and $\Delta y$. The small increments formula for functions of two variables gives an approximation to the change in utility $\Delta U$, expressing it as the sum of a ‘free time effect’ and an ‘exam grade effect’:

$$\Delta U \approx \frac{\partial U}{\partial t} \Delta t + \frac{\partial U}{\partial y} \Delta y$$

If the changes $\Delta t$ and $\Delta y$ are such that Alexei stays on the same indifference curve, then his utility does not change; thus $\Delta U = 0$, which implies that

$$\frac{\partial U}{\partial t} \Delta t + \frac{\partial U}{\partial y} \Delta y \approx 0$$

Rearranging,

$$\frac{ \Delta y}{\Delta t} \approx - \frac{\partial U}{\partial t} \left/ \frac{\partial U}{\partial y} \right.$$

The changes $\Delta t$ and $\Delta y$ together produce a small movement along an indifference curve. So if we now take the limit as $\Delta t\to 0$, the left-hand side approaches the slope of that curve and the approximation becomes an equation.

Thus the slope of the indifference curve through any point $(t,\ y)$ is given by the formula:

$$\frac{dy}{dt} = -\frac{\partial U}{\partial t} \left/ \frac{\partial U}{\partial y} \right.$$

marginal rate of substitution (MRS)

The trade-off that a person is willing to make between two goods. At any point, this is the slope of the indifference curve. See also: marginal rate of transformation.

The right-hand side of this equation is negative, since both marginal utilities are positive: increasing either free time or the exam grade increases Alexei’s utility. Thus indifference curves slope downward, as in the diagram. To reduce confusion, we usually define the marginal rate of substitution (MRS) as the absolute value of the slope. So:

$$\text{MRS} = \left| \frac{\partial U}{\partial t} \left/ \frac{\partial U}{\partial y} \right. \right|$$

or, in words,

$$\text{marginal rate of substitution} = \left| \frac{\text{marginal utility of free time}}{\text{marginal utility of exam grade}} \right|$$

Defining the MRS as a positive number allows us to say, for example, that the MRS is higher (Alexei is more willing to trade off grade points for free time) at points where the indifference curve is steeper, whereas the slope of the indifference curve is more negative at such points.

The MRS is the rate at which Alexei is prepared to trade grade points for additional hours of free time. The equation above, expressing the MRS as a ratio of marginal utilities, may be interpreted as follows: the MRS is approximately equal to the extra utility obtained from one more unit of free time, divided by the extra utility obtained from an additional grade point. As usual with interpretations of exact statements involving calculus in terms of individual units, the approximation is a good one if units are small quantities.

Convex preferences

Each indifference curve in Figure 1 becomes flatter as one moves along it to the right:

marginal rate of substitution (MRS)

The trade-off that a person is willing to make between two goods. At any point, this is the slope of the indifference curve. See also: marginal rate of transformation.

Alexei’s MRS falls if his free time becomes greater and his exam grade decreases in such a way as to keep his utility constant. This property of Alexei’s preferences is known as diminishing marginal rate of substitution and is usually assumed when we draw indifference curves with two goods.

Another way to describe this assumption is to note that Alexei’s indifference curves are convex. In algebraic terms, if we rewrite the equation of an indifference curve $U(t,\ y)=c$ in the form $y=g(t,\ c)$, then $g(t,\ c)$ is a decreasing and convex function of $t$ for given $c$. We say that Alexei has convex preferences.

A person whose preferences are convex always prefers mixtures of goods to extremes of either good. If we draw a line between two points on the same indifference curve, then each point on the line is a mixture of the two end-points. When the indifference curves are convex, all points on the line between the end-points give higher utility than the end-points.

We shall give an example of a utility function displaying diminishing MRS in the next section.

Read more: Sections 14.2 (for the small increments formula) and 15.1 (for contours and implicit differentiation) of Malcolm Pemberton and Nicholas Rau. 2015. Mathematics for economists: An introductory textbook, 4th ed. Manchester: Manchester University Press.

An example: The Cobb-Douglas utility function

In this section, we look at a particular utility function that is often used in economic modelling. We derive expressions for the marginal utilities and the marginal rate of substitution, and verify their properties.

As before, Alexei cares about free time and his exam grade. Suppose that his utility function is:

$$U (t,\ y)= t^\alpha y^\beta$$

where $\alpha$ and $\beta$ are positive constants. This function has some very convenient mathematical properties. It is called a Cobb-Douglas function after the two people who introduced it into economics.

To find the marginal utilities of free time and exam grade, we must find the partial derivatives of the utility function. Differentiating $U$ with respect to $t$, we see that the marginal utility of free time is:

$$\frac{\partial U}{\partial t} = \alpha t^{\alpha -1} y^\beta$$

We know from the utility function that $t^{\alpha -1} y^\beta = U/t$, which gives us a simpler expression for the marginal utility of free time:

$$\frac{\partial U}{\partial t} = \frac{\alpha U}{t}$$

Similarly, the marginal utility of the exam grade is:

$$\frac{\partial U}{\partial y} = \beta t^{\alpha} y^{\beta-1} = \frac{\beta U}{y}$$

Notice that when $t$ and $y$ are positive, so is $U$. Hence the assumption that $\alpha$ is also positive implies that $\partial U/\partial t \gt0$. Similarly, $\beta\gt0$ implies that $\partial U/\partial y \gt0$. In other words, the assumption that both $\alpha$ and $\beta$ are positive ensures that ‘goods are good’: Alexei’s utility rises as free time or grade points increase.

In the previous section, we defined the marginal rate of substitution (MRS) between free time and grade points as the absolute value of the slope of an indifference curve, and showed that it was equal to the ratio of the marginal utility of free time to the marginal utility of the exam grade. With the Cobb-Douglas utility function:

$$\text{MRS} = \frac{\partial U}{\partial t} \left/ \frac{\partial U}{\partial y} \right. = \frac{\alpha U}{t} \left/ \frac{\beta U}{y} \right. = \frac{\alpha y}{\beta t}$$

The indifference curves are downward sloping in $(t,\ y)$ space, so as we move to the right along an indifference curve, $t$ rises and $y$ falls, and thus $y/t$ falls. Since $\alpha$ and $\beta$ are positive, MRS also falls. Thus, the Cobb-Douglas utility function implies diminishing MRS.

Read more: Sections 15.1 and 15.2 of Malcolm Pemberton and Nicholas Rau. 2015. Mathematics for economists: An introductory textbook, 4th ed. Manchester: Manchester University Press.