Leibniz

3.4.1 Marginal rate of transformation

Alexei’s decision of how much to study is constrained by the feasible set of combinations of free time and grade points. So he faces a trade-off: to get a good grade at the end of his course, he has to give up some free time. The marginal rate of transformation (MRT) measures the size of the trade-off. Here we show how the MRT can be calculated from the production function.

The equation of the feasible frontier

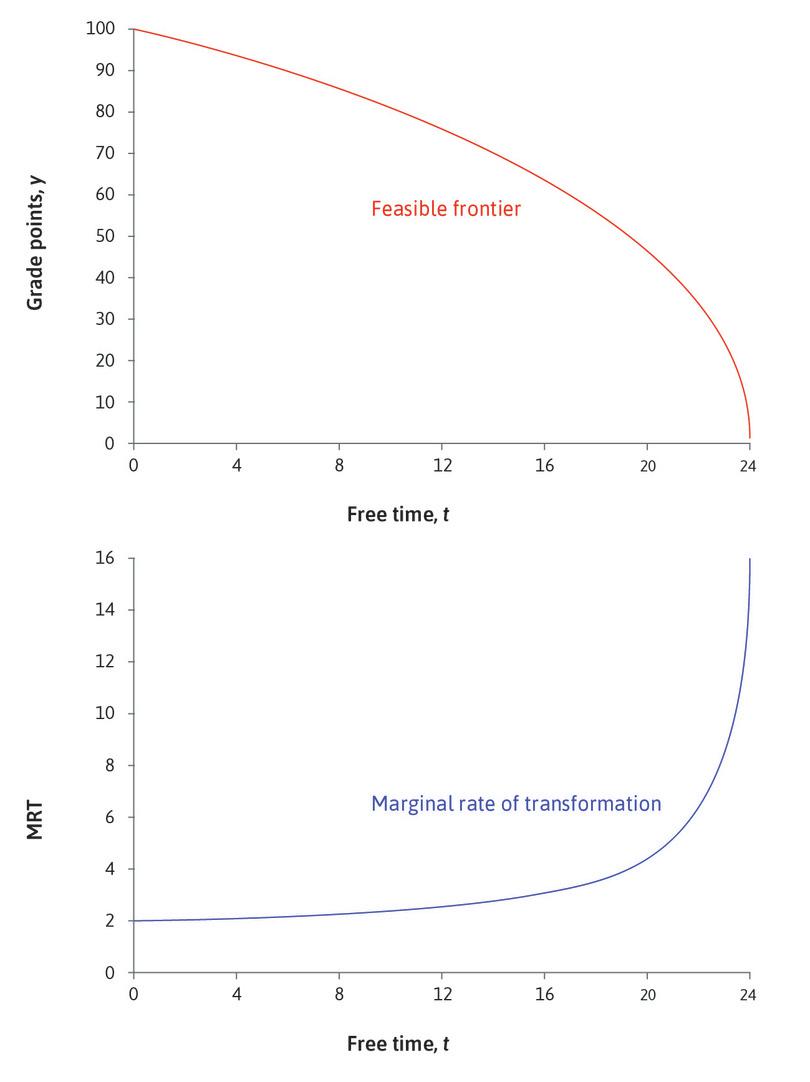
Figure 1 shows Alexei’s feasible set. Recall that we constructed the feasible frontier using a production function that relates exam grade to hours of study.

To see this mathematically, let Alexei’s production function be (as before):

$$y = f(h)$$

where $y$ is his output (the exam grade) and $h$ his hours of study, and $f$ is an increasing function.

The feasible frontier is the relationship between the grade and free time. If Alexei takes $t$ hours of free time, his hours of study are:

$$h=24-t$$

Substituting this into the production function, we obtain the equation of the feasible frontier:

$$y = f(24 - t)$$

Calculating the marginal rate of transformation

We have seen diagrammatically that the MRT is related to the slope of the feasible frontier. We can find the slope by differentiating the equation of the feasible frontier. When Alexei has $t$ hours of free time, the rate at which his grade changes as free time increases is given by:

$$\frac{dy}{dt} = f'(24-t)\frac{d}{dt}(24 -t)$$

using the composite function rule (sometimes called the chain rule). Simplifying,

$$\frac{dy}{dt} = - f'(24-t)$$

The right-hand side of this equation is negative, since $f$ is an increasing function. Thus, the frontier slopes downward, as in the diagram. The slope of the feasible frontier at the point $(t,\ f(24 -t))$ is the negative quantity $-f'(24 -t)$.

marginal rate of transformation (MRT)

The quantity of some good that must be sacrificed to acquire one additional unit of another good. At any point, it is the slope of the feasible frontier. See also: marginal rate of substitution.

The negative slope tells us that the grade decreases as free time increases. The marginal rate of transformation (MRT) is the rate at which the grade increases as free time is given up, which is given by the absolute value of the slope, a positive quantity:

$$\text{MRT}=f'(24-t)$$

The meaning of the MRT is as follows: if free time increases by a small amount, say $\Delta t$ hours, the exam grade decreases by approximately $f'(24-t)\Delta t$ grade points. Or if free time decreases by $\Delta t$ hours, the exam grade increases by approximately $f'(24-t)\Delta t$ grade points. Figure 2 shows the feasible frontier for the production function $y=28h^{1.6}$ (which has a similar, but not identical, shape to Alexei’s feasible frontier). The lower panel shows the MRT, which rises as we move to the right along the frontier, increasing free time and lowering the exam grade.

To summarize, the MRT measures the rate at which grade points have to be given up if hours of free time increase, and can be found by simply differentiating the production function. Since the number of hours of study $h$ is equal to $24 - t$, the MRT is the same as the marginal product of labour $f'(h)$. The fact that the MRT rises as we move along the frontier in the direction of more free time and fewer hours of study is a consequence of diminishing returns to labour: since $f'(h)$ is a decreasing function of $h$, it rises when $h$ falls.