Unit 5 Property and power: Mutual gains and conflict
Themes and capstone units
How institutions influence the balance of power in economic interactions, and affect the fairness and efficiency of the allocations that result
- Technology, biology, economic institutions, and people’s preferences are all important determinants of economic outcomes.
- Power is the ability to do and get the things we want in opposition to the intentions of others.
- Interactions between economic actors can result in mutual gains, but also in conflicts over how the gains are distributed.
- Institutions influence the power and other bargaining advantages of actors.
- The criteria of efficiency and fairness can help evaluate economic institutions and the outcomes of economic interactions.
Perhaps one of your distant ancestors considered that the best way to get money was by shipping out with a pirate like Blackbeard or Captain Kidd. If he had settled on Captain Bartholomew Roberts’ pirate ship the Royal Rover, he and the other members of the crew would have been required to consent to the ship’s written constitution. This document (called The Royal Rover’s Articles) guaranteed, among other things, that:1
Article I
Every Man has a Vote in the Affairs on the Moment; has equal title to fresh Provisions …Article III
No person to Game at Cards or Dice for Money.Article IV
The Lights and Candles to be put out at eight a-Clock at Night; If any of the Crew after that Hour still remained enclined for drinking, they are to do so on the open Deck …Article X
The Captain and Quarter Master to receive two Shares of a Prize (the booty from a captured ship); the Master, Boatswain, and Gunner one Share and a half, and other Officers one and a Quarter (everyone else to receive one share, called his Dividend.)Article XI
The Musicians to have Rest on the Sabbath Day but the other six Days and Nights none without special Favour.
The Royal Rover and its Articles were not unusual. During the heyday of European piracy in the late seventeenth and early eighteenth centuries, most pirate ships had written constitutions that guaranteed even more powers to the crew members. Their captains were democratically elected (‘the Rank of Captain being obtained by the Suffrage of the Majority’). Many captains were also voted out, at least one for cowardice in battle. Crews also elected one of their number as the quartermaster who, when the ship was not in a battle, could countermand the captain’s orders.
If your ancestor had served as a lookout and had been the first to spot a ship that was later taken as a prize, he would have received as a reward ‘the best Pair of Pistols on board, over and above his Dividend’. Were he to have been seriously wounded in battle, the articles guaranteed him compensation for the injury (more for the loss of a right arm or leg than for the left). He would have worked as part of a multiracial, multi-ethnic crew of which probably about a quarter were of African origin, and the rest primarily of European descent, including Americans.
The result was that a pirate crew was often a close-knit group. A contemporary observer lamented that the pirates were ‘wickedly united, and articled together’. Sailors of captured merchant ships often happily joined the ‘roguish Commonwealth’ of their pirate captors.
Another unhappy commentator remarked: ‘These Men whom we term … the Scandal of human Nature, who were abandoned to all Vice … were strictly just among themselves.’ If they were Responders in the ultimatum game (explained in Unit 4, Section 4.10), by this description they would have rejected any offer less than half of the pie!
5.1 Institutions and power
Nowhere else in the world during the late seventeenth and early eighteenth century did ordinary workers have the right to vote, to receive compensation for occupational injuries, or to be protected from the kinds of checks on arbitrary authority that were taken for granted on the Royal Rover. The Royal Rover’s articles laid down in black and white the understandings among the pirates about their working conditions. They determined who did what aboard the ship and what each person would get. For example, the size of the helmsman’s dividend compared to that of the gunner. There were also unwritten informal rules of appropriate behaviour that the pirates followed by custom, or to avoid condemnation by their crewmates.
Institutions
Institutions are written and unwritten rules that govern:
- what people do when they interact in a joint project
- the distribution of the products of their joint effort
These rules, both written and unwritten, were the institutions that governed the interactions among the crew members of the Royal Rover.
- incentive
- Economic reward or punishment, which influences the benefits and costs of alternative courses of action.
The institutions provided both the constraints (no drinking after 8 p.m. unless on deck) and the incentives (the best pair of pistols for the lookout who spotted a ship that was later taken). In the terminology of game theory from the previous unit, we could say that they were the ‘rules of the game’, specifying, as in the ultimatum game in Section 4.10, who can do what, when they can do it, and how the players’ actions determine their payoffs.
In this unit, we use the terms ‘institutions’ and ‘rules of the game’ interchangeably.
Experiments in Unit 4 showed us that the rules of the game affect:
- how the game is played
- the size of the total payoff available to those participating
- how this total is divided
For example, in the ultimatum game the rules (institutions) specify the size of the pie, who gets to be the Proposer, what the Proposer can do (offer any fraction of the pie), what the Responder can do (accept or refuse), and who gets what as a result.
We also saw that changing the rules of the game changes the outcome. In particular, when there are two Responders in the ultimatum game, they are more likely to accept lower offers because each is not sure what the other will do. And this means that the Proposer can make a lower offer, and obtain a higher payoff.
Since institutions determine who can do what, and how payoffs are distributed, they determine the power individuals have to get what they want in interactions with others.
Power
The ability to do and get the things we want in opposition to the intentions of others.
Power in economics takes two main forms:
- It may set the terms of an exchange: By making a take-it-or-leave-it offer (as in the ultimatum game).
- It may impose or threaten to impose heavy costs: Unless the other party acts in a way that benefits the person with power.
- bargaining power
- The extent of a person’s advantage in securing a larger share of the economic rents made possible by an interaction.
The rules of the ultimatum game determine the ability that the players have to obtain a high payoff—the extent of their advantage when dividing the pie—which is a form of power called bargaining power. The power to make a take-it-or-leave-it offer gives the Proposer more bargaining power than the Responder, and usually results in the Proposer getting more than half of the pie. Still, the Proposer’s bargaining power is limited because the Responder has the power to refuse. If there are two Responders, the power to refuse is weaker, so the Proposer’s bargaining power is increased.
In experiments the assignment of the role Proposer or Responder, and hence the assignment of bargaining power, is usually done by chance. In real economies, the assignment of power is definitely not random.
In the labour market, the power to set the terms of the exchange typically lies with those who own the factory or business: they are the ones proposing the wage and other terms of employment. Those seeking employment are like Responders, and since usually more than one person is applying for the same job, their bargaining power may be low, just as in the ultimatum game with more than one Responder. Also, because the place of employment is the employer’s private property, the employer may be able to exclude the worker by firing her unless her work is up to the specifications of the employer.
Remember from Units 1 and 2 that the productivity of labour started to increase in Britain around the middle of the seventeenth century. But it was not until the middle of the nineteenth century that a combination of shifts in the supply and demand for labour, and new institutions such as trade unions and the right to vote for workers, gave wage earners the bargaining power to raise wages substantially.
We will see in the next unit how the labour market, along with other institutions, gives both kinds of power to employers. In Unit 7 we explain how some firms have the power to set high prices for their products, and in Unit 10, how the credit market gives power to banks and other lenders over people seeking mortgages and loans.
The power to say no
Suppose we allow a Proposer simply to divide up a pie in any way, without any role for the Responder other than to take whatever he gets (if anything). Under these rules, the Proposer has all the bargaining power and the Responder none. There is an experimental game like this, and it is called (you guessed it) the dictator game.
There are many past and present examples of economic institutions that are like the dictator game, in which there is no option to say no. Examples include today’s remaining political dictatorships, such as The Democratic People’s Republic of Korea (North Korea), and slavery, as it existed in the US prior to the end of the American Civil War in 1865. Criminal organizations involved in drugs and human trafficking would be another modern example, in which power may take the form of physical coercion or threats of violence.
In a capitalist economy in a democratic society, institutions exist to protect people against violence and coercion, and to ensure that most economic interactions are conducted voluntarily. Later in this unit we study the outcome of an interaction involving coercion, and how it changes with the power to say no.
5.2 Evaluating institutions and outcomes: The Pareto criterion
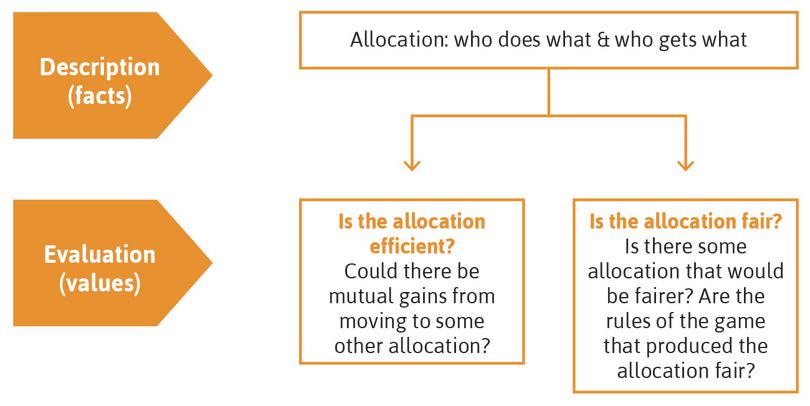
Whether it is fishermen seeking to make a living while not depleting the fish stocks, or farmers maintaining the channels of an irrigation system, or two people dividing up a pie, we want to be able to both describe what happens and to evaluate it—is it better or worse than other potential outcomes? The first involves facts; the second involves values.
- allocation
- A description of who does what, the consequences of their actions, and who gets what as a result.
We call the outcome of an economic interaction an allocation.
In the ultimatum game, for example, the allocation describes the proposed division of the pie by the Proposer, whether it was rejected or accepted, and the resulting payoffs to the two players.
The Pareto criterion
According to the Pareto criterion, allocation A dominates allocation B if at least one party would be better off with A than B, and nobody would be worse off.
We say that A Pareto-dominates B.
- Pareto dominant
- Allocation A Pareto-dominates allocation B if at least one party would be better off with A than B, and nobody would be worse off. See also: Pareto efficient.
Now suppose that we want to compare two possible allocations, A and B, that may result from an economic interaction. Can we say which is better? Suppose we find that everyone involved in the interaction would prefer allocation A. Then most people would agree that A is a better allocation than B. This criterion for judging between A and B is called the Pareto criterion, after Vilfredo Pareto, an Italian economist and sociologist.
Note that when we say an allocation makes someone ‘better off’ we mean that they prefer it, which does not necessarily mean they get more money.
Great economists Vilfredo Pareto
Vilfredo Pareto (1848–1923), an Italian economist and sociologist, earned a degree in engineering for his research on the concept of equilibrium in physics. He is mostly remembered for the concept of efficiency that bears his name. He wanted economics and sociology to be fact-based sciences, similar to the physical sciences that he had studied when he was younger.
His empirical investigations led him to question the idea that the distribution of wealth resembles the familiar bell curve, with a few rich and a few poor in the tails of the distribution and a large middle-income class. In its place he proposed what came to be called Pareto’s law, according to which, across the ages and differing types of economy, there were very few rich people and a lot of poor people.
His 80–20 rule—derived from Pareto’s law—asserted that the richest 20% of a population typically held 80% of the wealth. Were he living in the US in 2015, he would have to revise that to 90% of the wealth held by the richest 20%, suggesting that his law might not be as universal as he had thought.
In Pareto’s view, the economic game was played for high stakes, with big winners and losers. Not surprisingly, then, he urged economists to study conflicts over the division of goods, and he thought the time and resources devoted to these conflicts were part of what economics should be about.2 In his most famous book, the Manual of Political Economy (1906), he wrote that: ‘The efforts of men are utilized in two different ways: they are directed to the production or transformation of economic goods, or else to the appropriation of goods produced by others.’
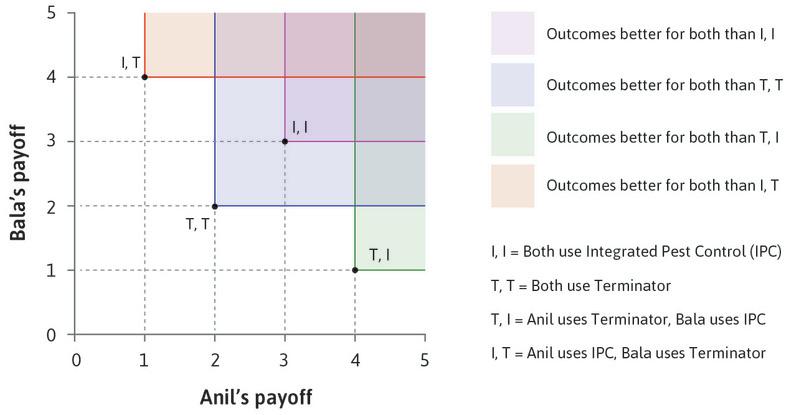
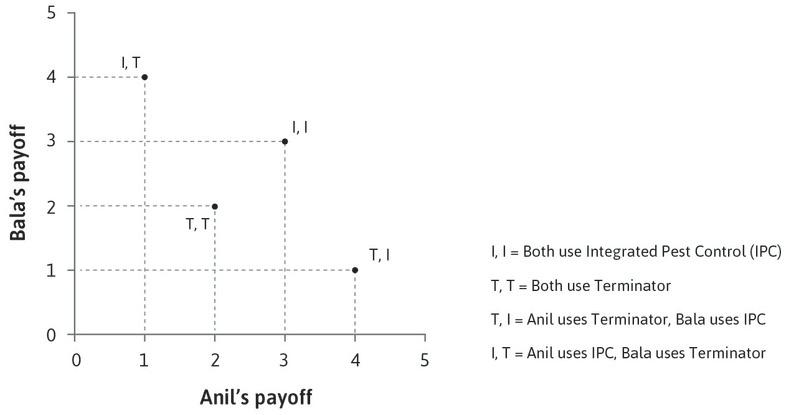
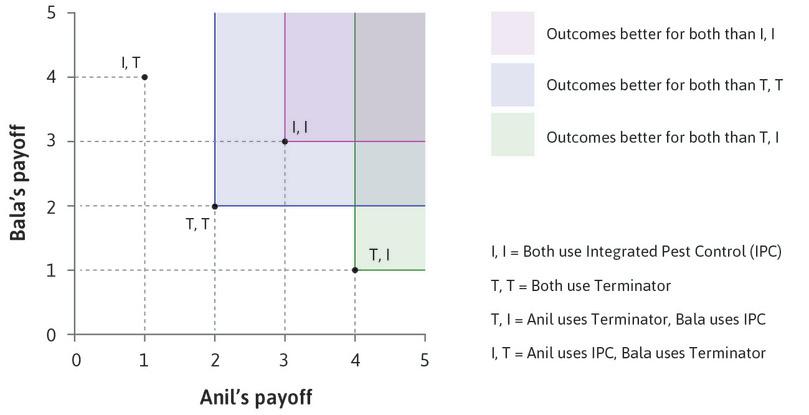
Figure 5.1 compares the four allocations in the pest control game from Unit 4 by the Pareto criterion (using a similar method to the comparison of technologies in Unit 2). We assume that Anil and Bala are self-interested, so they prefer allocations with a higher payoff for themselves.
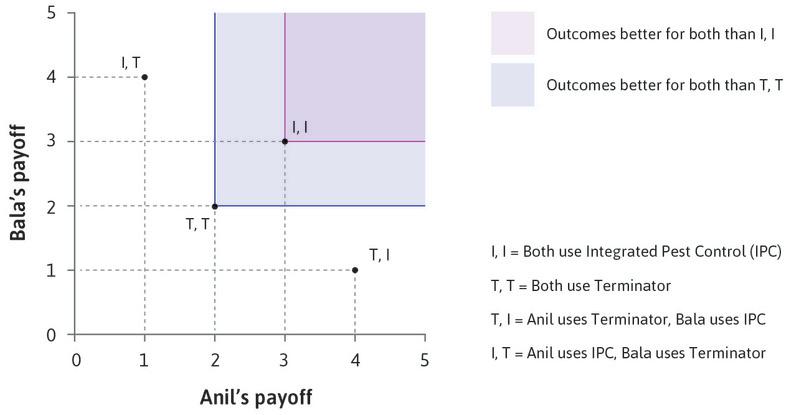
The blue rectangle with its corner at allocation (T, T) shows that (I, I) Pareto dominates (T, T). Follow the steps in Figure 5.1 to see more comparisons.
You can see from this example that the Pareto criterion may be of limited help in comparing allocations. Here, it tells us only that (I, I) is better than (T, T).
- Pareto efficient
- An allocation with the property that there is no alternative technically feasible allocation in which at least one person would be better off, and nobody worse off.
The diagram also shows that three of the four allocations are not Pareto-dominated by any other. An allocation with this property is called Pareto efficient.
Pareto efficiency
An allocation that is not Pareto-dominated by any other allocation is described as Pareto efficient.
- Pareto criterion
- According to the Pareto criterion, a desirable attribute of an allocation is that it be Pareto-efficient. See also: Pareto dominant.
If an allocation is Pareto efficient, then there is no alternative allocation in which at least one party would be better off and nobody worse off. The concept of Pareto efficiency is very widely used in economics and sounds like a good thing, but we need to be careful with it:
- There is often more than one Pareto-efficient allocation: In the pest-control game there are three.
- The Pareto criterion does not tell us which of the Pareto-efficient allocations is better: It does not give us any ranking of (I, I), (I, T) and (T, I).
- If an allocation is Pareto efficient, this does not mean we should approve of it: Anil playing IPC and Bala free riding by playing Terminator is Pareto efficient, but we (and Anil) may think this is unfair. Pareto efficiency has nothing to do with fairness.
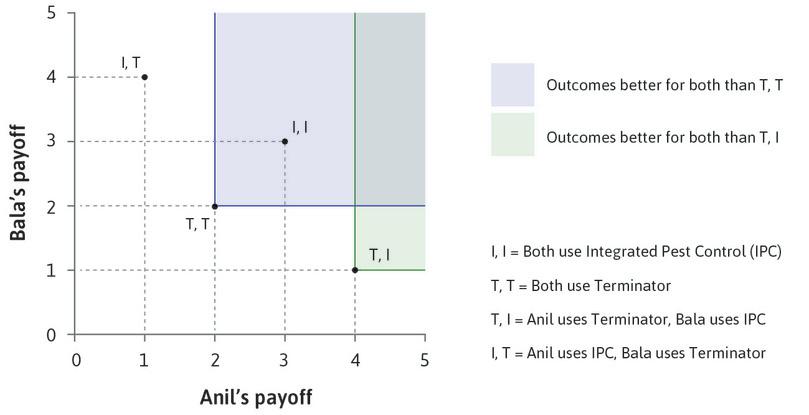
- Allocation (T, I) is Pareto efficient and (T, T) is not (it is Pareto inefficient): But the Pareto criterion does NOT tell us which is better.
There are many Pareto-efficient allocations that we would not evaluate favourably. If you look back at Figure 4.5 you can see that any split of Anil’s lottery winnings (including giving Bala nothing) would be Pareto efficient (choose any point on the boundary of the feasible set of outcomes, and draw the rectangle with its corner at that point: there are no feasible points above and to the right). But some of these splits would seem very unfair. Similarly, in the ultimatum game an allocation of one cent to the Responder and $99.99 to the Proposer is also Pareto efficient, because there is no way to make the Responder better off without making the Proposer worse off.
The same is true of problems such as the allocation of food. If some people are more than satisfied while others are starving, we might say in everyday language: ‘This is not a sensible way to provide nutrition. It is clearly inefficient.’ But Pareto efficiency means something different. A very unequal distribution of food can be Pareto efficient as long as all the food is eaten by someone who enjoys it even a little.
Question 5.1 Choose the correct answer(s)
Which of the following statements about the outcome of an economic interaction is correct?
- If the allocation is Pareto efficient, there is no allocation that Pareto dominates it: that is, no allocation where someone is better off without others being worse off.
- Pareto-efficient allocations can be very unfair, in which case it is likely that at least one participant would not be happy with the outcome.
- There can be more than one Pareto-efficient outcome. We saw that three of the four allocations in the pest control game were Pareto efficient.
- It is possible that one of the participants is worse off in the Pareto-efficient outcome, in which case it would not be better by the Pareto criterion. In the pest control game (T, I) is efficient but not better than (T, T).
5.3 Evaluating institutions and outcomes: Fairness
Although the Pareto criterion can help us to evaluate allocations, we will also want to use another criterion: justice. We will ask, is it fair?
Suppose, in the ultimatum game, the Proposer offered one cent from a total of $100. As we saw in Unit 4, Responders in experiments around the world typically reject such an offer, apparently judging it to be unfair. Many of us would have a similar reaction if we witnessed two friends, An and Bai, walking down the street. They spot a $100 bill, which An picks up. She offers one cent to her friend Bai, and says she wants to keep the rest.
We might be outraged. But we might think differently if we discovered that, though both An and Bai had worked hard all their lives, An had just lost her job and was homeless while Bai was well off. Letting An keep $99.99 might then seem fair. Thus we might apply a different standard of justice to the outcome when we know all of the facts.
We could also apply a standard of fairness not to the outcome of the game, but to the rules of the game. Suppose we had observed An proposing an even split, allocating $50 to Bai. Good for An, you say, that seems like a fair outcome. But if this occurred because Bai pulled a gun on An, and threatened that unless she offered an even split she would shoot her, we would probably judge the outcome to be unfair.
- substantive judgements of fairness
- Judgements based on the characteristics of the allocation itself, not how it was determined. See also: procedural judgements of fairness.
- procedural judgements of fairness
- An evaluation of an outcome based on how the allocation came about, and not on the characteristics of the outcome itself, (for example, how unequal it is). See also: substantive judgements of fairness.
The example makes a basic point about fairness. Allocations can be judged unfair because of:
- How unequal they are: In terms of income, for example, or subjective wellbeing. These are substantive judgments of fairness.
- How they came about: For example by force, or by competition on a level playing field. These are procedural judgements of fairness.
Substantive and procedural judgements
To make a substantive judgement about fairness, all you need to know is the allocation itself. However, for procedural evaluations we also need to know the rules of the game and other factors that explain why this allocation occurred.
Two people making substantive evaluations of fairness about the same situation need not agree, of course. For example, they may disagree about whether fairness should be evaluated in terms of income or happiness. If we measure fairness using happiness as the criterion, a person with a serious physical or mental handicap may need much more income than a person without such disabilities to be equally satisfied with his or her life.3
Substantive judgements
These are based on inequality in some aspect of the allocation such as:
- Income: The reward in money (or some equivalent measure) of the individual’s command over valued goods and services.
- Happiness: Economists have developed indicators by which subjective wellbeing can be measured.
- Freedom: The extent that one can do (or be) what one chooses without socially imposed limits.
Exercise 5.1 Substantive fairness
Consider the society you live in, or another society with which you are familiar.
- To make society fairer, would you want greater equality of income, happiness, or freedom? Why? Would there be a trade-off between these aspects?
- Are there other things that should be more equal to achieve greater fairness in this society?
Procedural judgements
The rules of the game that brought about the allocation may be evaluated according to aspects such as:
- Voluntary exchange of private property acquired by legitimate means: Were the actions resulting in the allocation the result of freely chosen actions by the individuals involved, for example each person buying or selling things that they had come to own through inheritance, purchase, or their own labour? Or was fraud or force involved?
- Equal opportunity for economic advantage: Did people have an equal opportunity to acquire a large share of the total to be divided up, or were they subjected to some kind of discrimination or other disadvantage because of their race, sexual preference, gender, or who their parents were?
- Deservingness: Did the rules of the game that determined the allocation take account of the extent to which an individual worked hard, or otherwise upheld social norms?
Exercise 5.2 Procedural fairness
Consider the society in which you live, or another society with which you are familiar. How fair is this society, according to the procedural judgements of fairness listed above?
We can use these differing judgements to evaluate an outcome in the ultimatum game. The experimental rules of the game will appear to most people’s minds as procedurally fair:
- Proposers are chosen randomly.
- The game is played anonymously.
- Discrimination is not possible.
- All actions are voluntary. The Responder can refuse to accept the offer, and the Proposer is typically free to propose any amount.
Substantive judgements are evaluations of the allocation itself: how the pie is shared. We know from the behaviour of experimental subjects that many people would judge an allocation in which the Proposer took 90% of the pie to be unfair.
Evaluating fairness
The rules of the game in the real economy are a long way from the fair procedures of the ultimatum game, and procedural judgements of unfairness are very important to many people, as we will see in Unit 19 (Economic inequality).
People’s values about what is fair differ. Some, for example, regard any amount of inequality as fair, as long as the rules of the game are fair. Others judge an allocation to be unfair if some people are seriously deprived of basic needs, while others consume luxuries.
The American philosopher John Rawls (1921–2002) devised a way to clarify these arguments, which can sometimes help us to find common ground on questions of values. We follow three steps:
- We adopt the principle that fairness applies to all people: For example, if we swapped the positions of An and Bai, so that it was Bai instead of An who picked up $100, we would still apply exactly the same standard of justice to evaluate the outcome.
- Imagine a veil of ignorance: Since fairness applies to everyone, including ourselves, Rawls asks us to imagine ourselves behind what he called a veil of ignorance, not knowing the position that we would occupy in the society we are considering. We could be male or female, healthy or ill, rich or poor (or with rich or poor parents), in a dominant or an ethnic minority group, and so on. In the $100 on the street game, we would not know if we would be the person picking up the money, or the person responding to the offer.
- From behind the veil of ignorance, we can make a judgement: For example, the choice of a set of institutions—imagining as we do so that we will then become part of the society we have endorsed, with an equal chance of having any of the positions occupied by individuals in that society.
The veil of ignorance invites you, in making a judgement about fairness, to put yourself in the shoes of others quite different from yourself. You would then, Rawls argued, be able to evaluate the constitutions, laws, inheritance practices, and other institutions of a society as an impartial outsider.
Exercise 5.3 Splitting the profits in a partnership
Suppose you and a partner are starting a business involving each of you selling a new app to the public. You are deciding how to divide the profits and are considering four alternatives. The profits could be split:
- equally
- in proportion to how many apps each of you sells
- in inverse proportion to how much income each of you has from other sources (for example, if one of you has twice the income of the other, the profits could be split one-third to the former and two-thirds to the latter)
- in proportion to how many hours each of you has spent selling.
Order these alternatives according to your preference and give arguments based on the concepts of fairness introduced in this section. If the order depends on other facts about this joint project, say what other facts you would need.
Neither philosophy, nor economics, nor any other science, can eliminate disagreements about questions of value. But economics can clarify:
- How the dimensions of unfairness may be connected: For example, how the rules of the game that give special advantages to one or another group may affect the degree of inequality.
- The trade-offs between the dimensions of fairness: For example, do we have to compromise on the equality of income if we also want equality of opportunity?
- Public policies to address concerns about unfairness: Also, whether these policies compromise other objectives.
5.4 A model of choice and conflict
In the remainder of this unit we explore some economic interactions and evaluate the resulting allocations. As in the experiments in Unit 4, we will see that both cooperation and conflict occur. As in the experiments, and in history, we will find that the rules matter.
Recall the model in Unit 3 of the farmer, Angela, who produces a crop. We will develop the model into a sequence of scenarios involving two characters:
- Initially, Angela works the land on her own, and gets everything she produces.
- Next, we introduce a second person, who does not farm, but would also like some of the harvest. He is called Bruno.
- At first, Bruno can force Angela to work for him. In order to survive, she has to do what he says.
- Later, the rules change: the rule of law replaces the rule of force. Bruno can no longer coerce Angela to work. But he owns the land and if she wants to farm his land, she must agree, for example, to pay him some part of the harvest.
- Eventually, the rules of the game change again in Angela’s favour. She and her fellow farmers achieve the right to vote and legislation is passed that increases Angela’s claim on the harvest.
For each of these steps we will analyse the changes in terms of both Pareto efficiency and the distribution of income between Angela and Bruno. Remember that:
- We can determine objectively whether an outcome is Pareto efficient or not.
- But whether the outcome is fair depends on your own analysis of the problem, using the concepts of substantive and procedural fairness.
- marginal rate of transformation (MRT)
- The quantity of some good that must be sacrificed to acquire one additional unit of another good. At any point, it is the slope of the feasible frontier. See also: marginal rate of substitution.
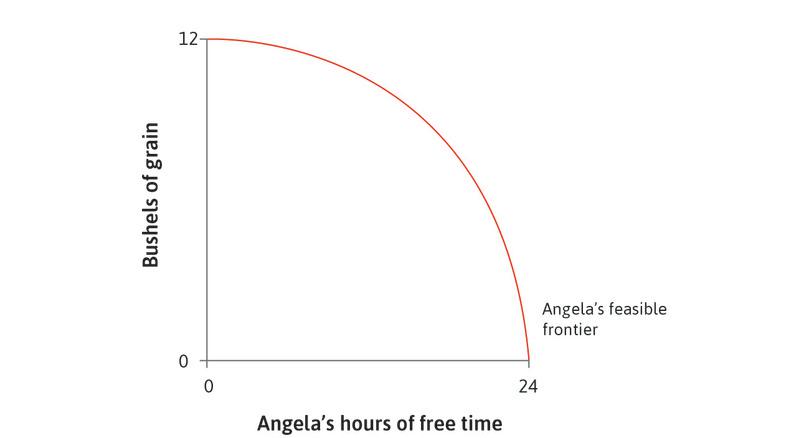
As before, Angela’s harvest depends on her hours of work, through the production function. She works the land, and enjoys the remainder of the day as free time. In Unit 3 she consumed the grain that this activity produced. Recall that the slope of the feasible frontier is the marginal rate of transformation (MRT) of free time into grain.
- marginal rate of substitution (MRS)
- The trade-off that a person is willing to make between two goods. At any point, this is the slope of the indifference curve. See also: marginal rate of transformation.
Angela values both grain and free time. Again, we represent her preferences as indifference curves, showing the combinations of grain and free time that she values equally. Remember that the slope of the indifference curve is called the marginal rate of substitution (MRS) between grain and free time.
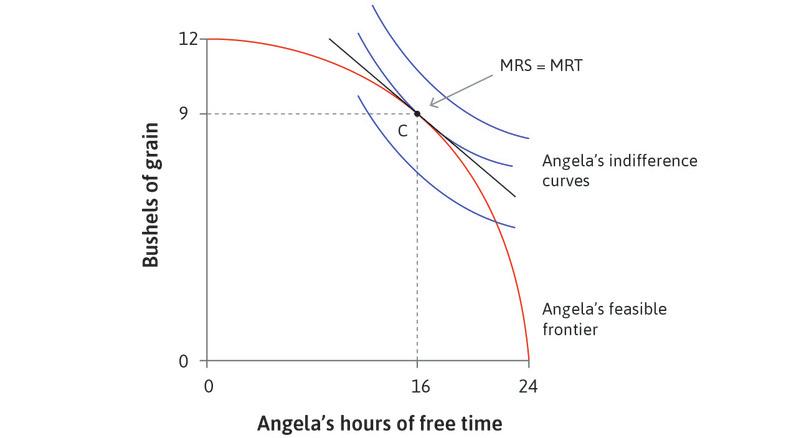
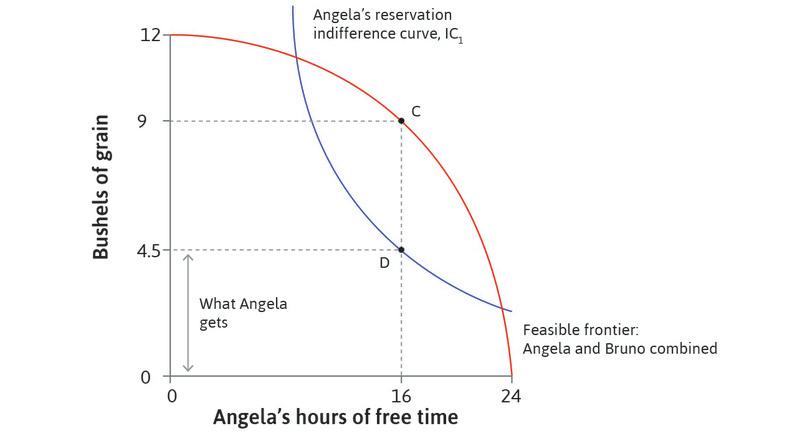
Angela works the land on her own
Figure 5.2 shows Angela’s indifference curves and her feasible frontier. The steeper the indifference curve, the more Angela values free time relative to grain. You can see that the more free time she has (moving to the right), the flatter the curves—she values free time less.
In this unit, we make a particular assumption (called quasi-linearity) about Angela’s preferences that you can see in the shape of her indifference curves. As she gets more grain, her MRS does not change. So the curves have the same slope as you move up the vertical line at 16 hours of free time. More grain does not change her valuation of free time relative to grain.
Why might this be? Perhaps she does not eat it all, but sells some and uses the proceeds to buy other things she needs. This is just a simplification (called quasi-linearity) that makes our model easier to understand. Remember: when drawing indifference curves for the model in this unit, simply shift them up and down, keeping the MRS constant at a given amount of free time.
Leibniz: Quasi-linear preferences
Angela is free to choose her typical hours of work to achieve her preferred combination of free time and grain. Work through Figure 5.2 to determine the allocation.
Figure 5.2 shows that the best Angela can do, given the limits set by the feasible frontier, is to work for 8 hours. She has 16 hours of free time, and produces and consumes 9 bushels of grain. This is the number of hours of work where the marginal rate of substitution is equal to the marginal rate of transformation. She cannot do better than this! (If you’re not sure why, go back to Unit 3 and check.)
Leibniz: Angela’s choice of working hours
A new character appears
But now, Angela has company. The other person is called Bruno; he is not a farmer but will claim some of Angela’s harvest. We will study different rules of the game that explain how much is produced by Angela, and how it is divided between her and Bruno. For example, in one scenario, Bruno is the landowner and Angela pays some grain to him as rent for the use of the land.
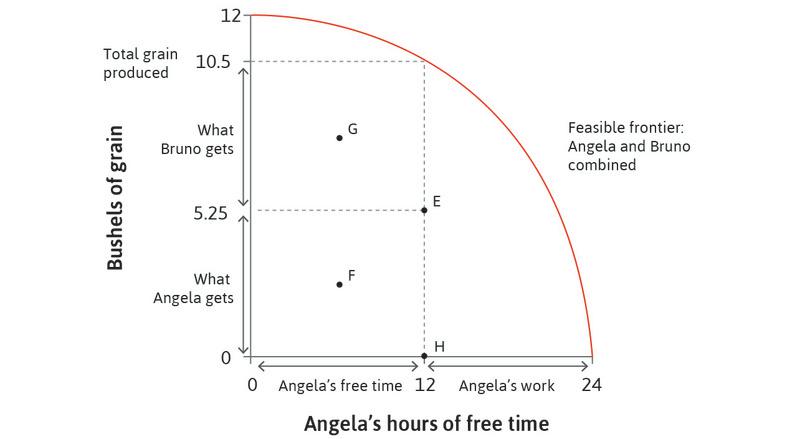
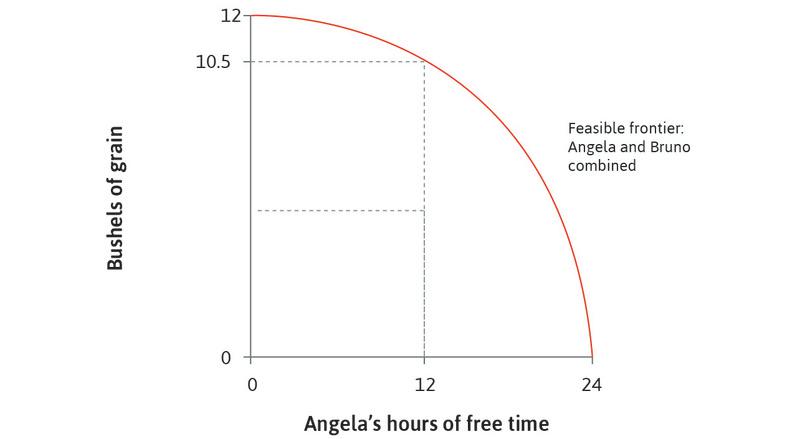
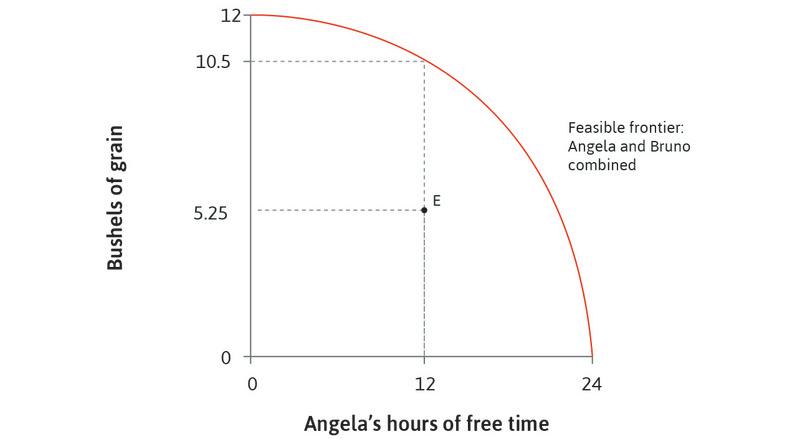
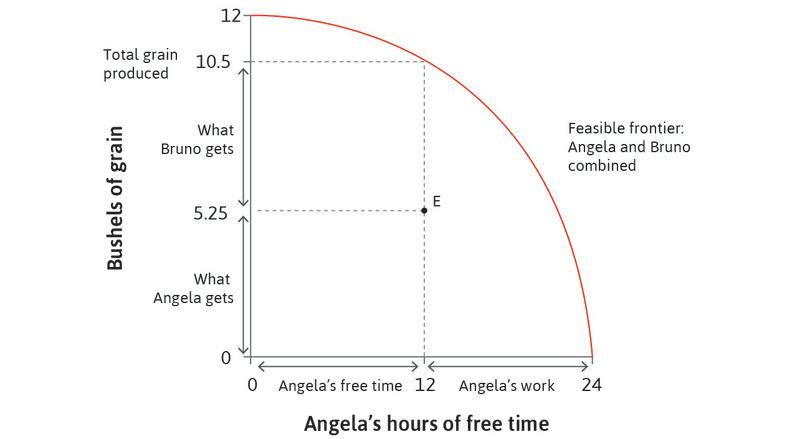
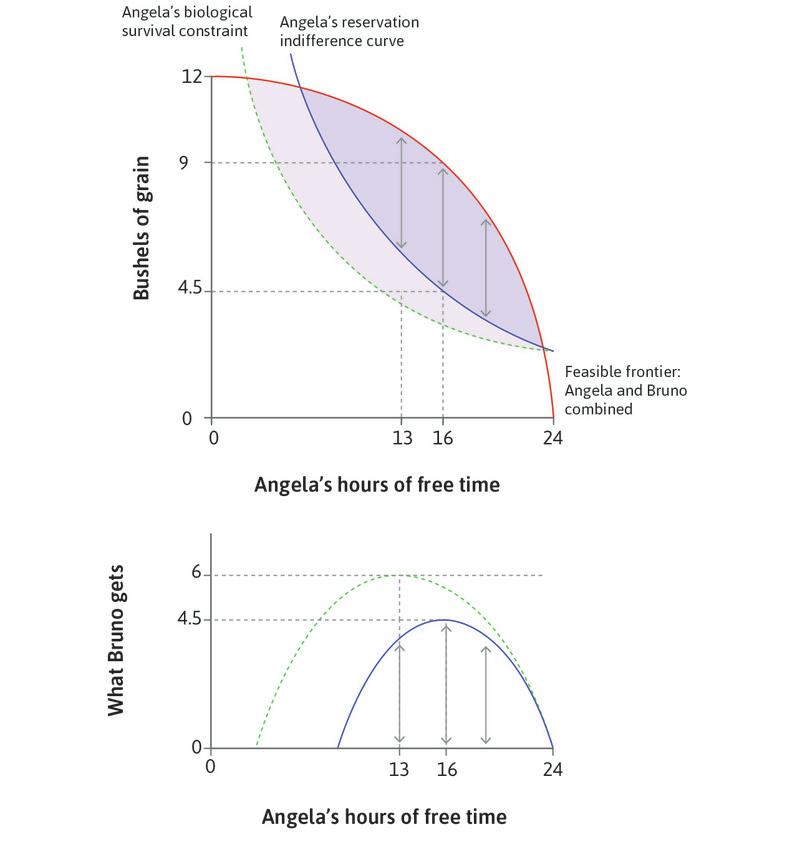
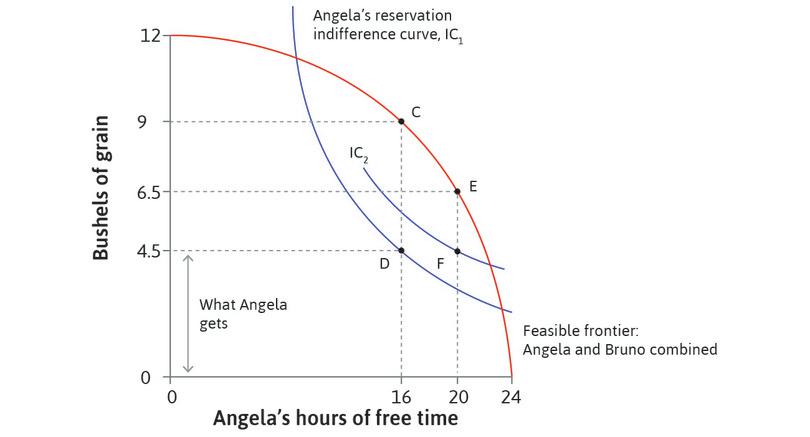
Figure 5.3 shows Angela and Bruno’s combined feasible frontier. The frontier indicates how many bushels of grain Angela can produce given how much free time she takes. For example, if she takes 12 hours free time and works for 12 hours, then she produces 10.5 bushels of grain. One possible outcome of the interaction between Angela and Bruno is that 5.25 bushels go to Bruno, and Angela retains the other 5.25 bushels for her own consumption.
Work through Figure 5.3 to find out how each possible allocation is represented in the diagram, showing how much work Angela did and how much grain she and Bruno each got.
Which allocations are likely to occur? Not all of them are even possible. For example, at point H Angela works 12 hours a day and receives nothing (Bruno takes the entire harvest), so Angela would not survive. Of the allocations that are at least possible, the one that will occur depends on the rules of the game.
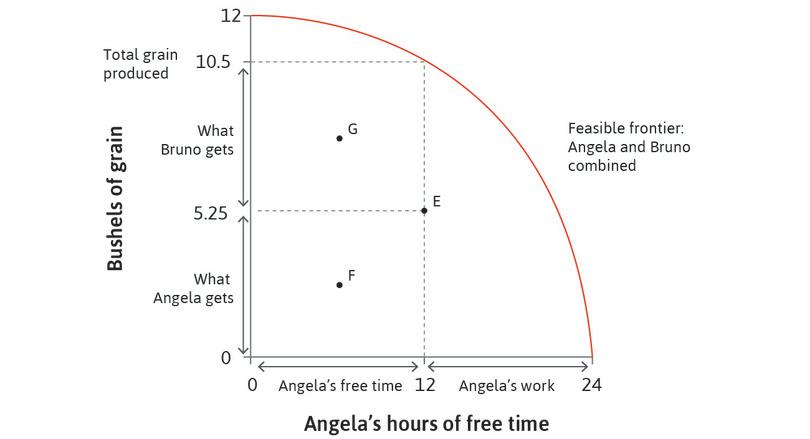
Exercise 5.4 Using indifference curves
In Figure 5.3, point F shows an allocation in which Angela works more and gets less than at point E, and point G shows the case in which she works more and gets more.
By sketching Angela’s indifference curves, work out what you can say about her preferences between E, F and G, and how this depends on the slope of the curves.
Question 5.2 Choose the correct answer(s)
Figure 5.3 shows Angela and Bruno’s combined feasible set, and four allocations that might result from an interaction between them.
From the figure, we can conclude that:
- Angela’s indifference curves are downward-sloping. If the indifference curve through G was sufficiently flat, the other three points would all lie below it.
- Whatever the slope of her indifference curves, Angela would prefer E to F, as it gives her more grain and more free time.
- Bruno gets an amount of grain equal to the vertical distance from the allocation to the feasible frontier. So G is the worst of the four allocations for him.
- Angela could be indifferent between G and E—one of her indifference curves could pass through both points.
5.5 Technically feasible allocations
Initially Angela could consume (or sell) everything she produced. Now Bruno has arrived, and he has a gun. He has the power to implement any allocation that he chooses. He is even more powerful than the dictator in the dictator game (in which a Proposer dictates how a pie is to be divided). Why? Bruno can determine the size of the pie, as well as how it is shared.
Unlike the experimental subjects in Unit 4, in this model Bruno and Angela are entirely self-interested. Bruno wants only to maximize the amount of grain he can get. Angela cares only about her own free time and grain (as described by her indifference curves).
We now make another important assumption. If Angela does not work the land, Bruno gets nothing (there are no other prospective farmers that he can exploit). What this means is that Bruno’s reservation option (what he gets if Angela does not work for him) is zero. As a result, Bruno thinks about the future: he will not take so much grain that Angela will die. The allocation must keep her alive.
- technically feasible
- An allocation within the limits set by technology and biology.
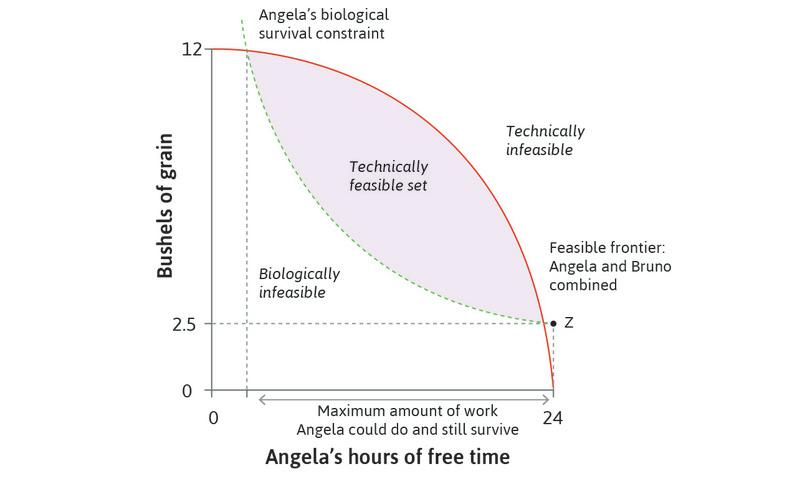
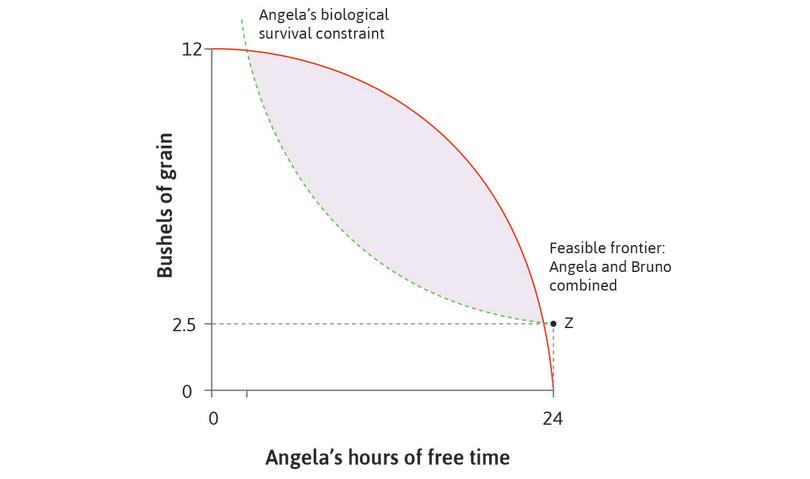
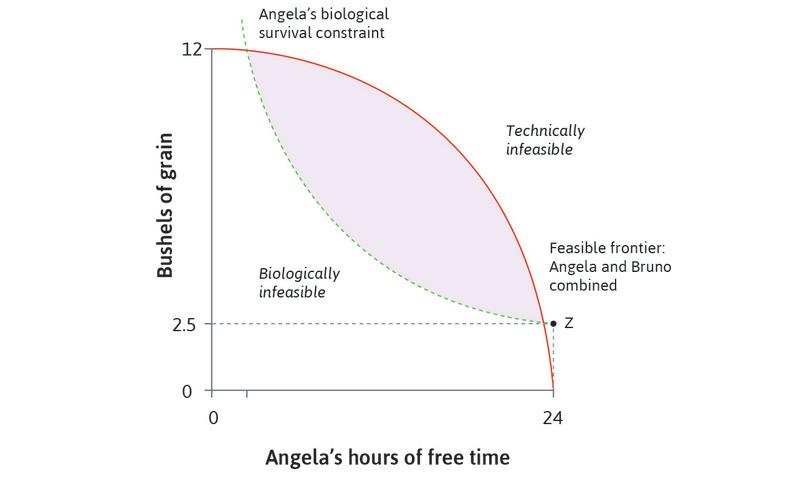
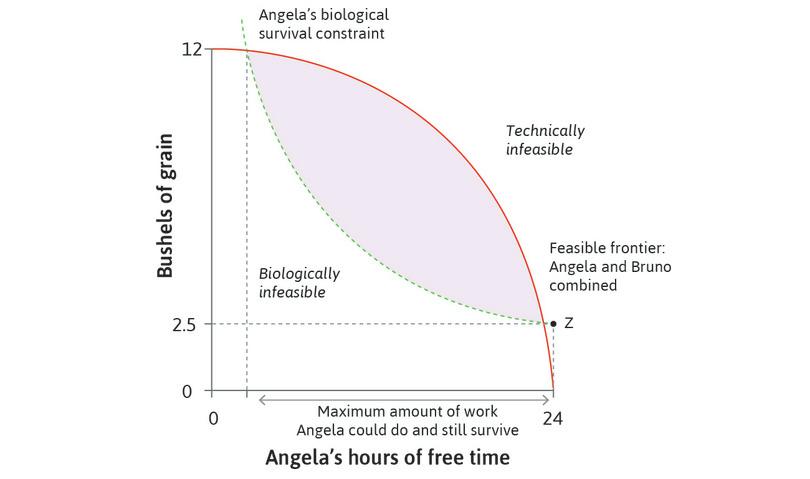
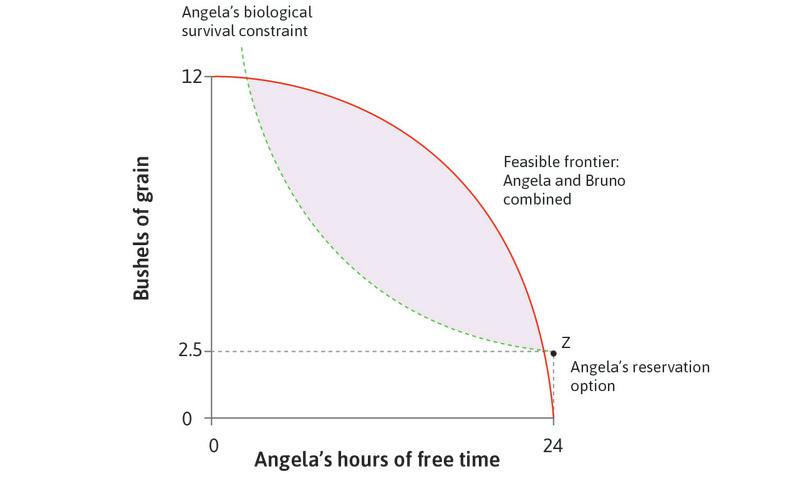
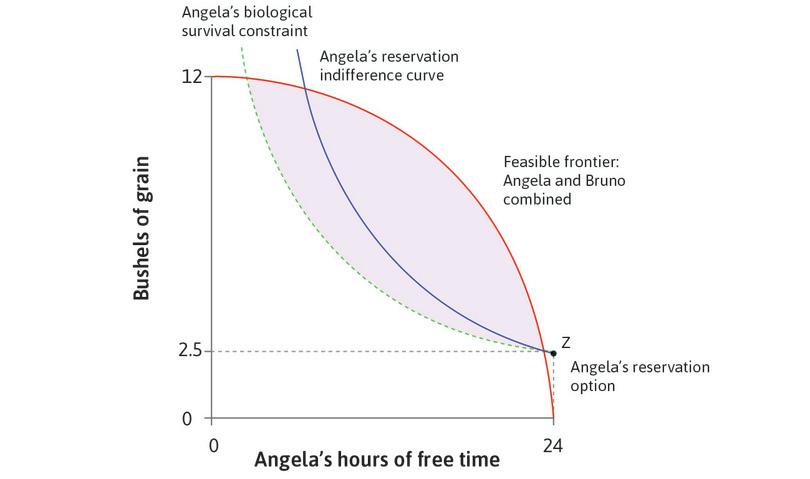
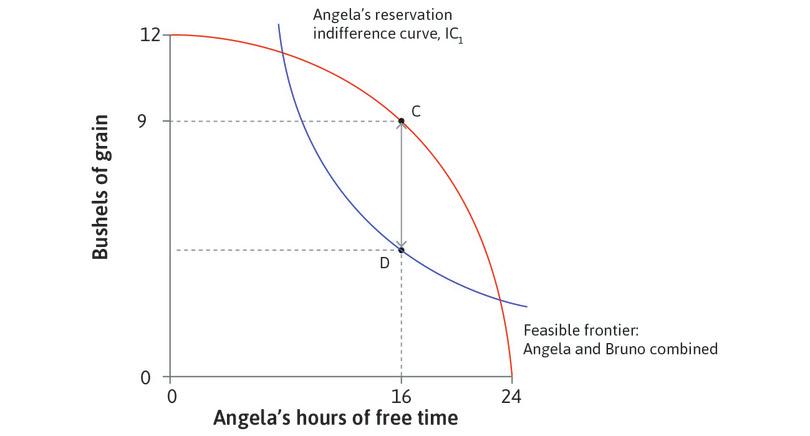
First, we will work out the set of technically feasible combinations of Angela’s hours of work and the amount of grain she receives: that is, all the combinations that are possible within the limitations of the technology (the production function) and biology (Angela must have enough nutrition to do the work and survive).
- biologically feasible
- An allocation that is capable of sustaining the survival of those involved is biologically feasible.
Figure 5.4 shows how to find the technically feasible set. We already know that the production function determines the feasible frontier. This is the technological limit on the total amount consumed by Bruno and Angela, which in turn depends on the hours that Angela works. Angela’s biological survival constraint shows the minimum amount of grain that she needs for each amount of work that she does; points below this line would leave her so undernourished or overworked that she would not survive. This constraint shows what is biologically feasible. Notice that if she expends more energy working, she needs more food; that’s why the curve rises from right to left from point Z as her hours of work increase. The slope of the biological survival constraint is the marginal rate of substitution between free time and grain in securing Angela’s survival.
The fact that Angela’s survival might be in jeopardy is not a hypothetical example. During the Industrial Revolution, life expectancy at birth in Liverpool, UK, fell to 25 years: slightly more than half of what it is today in the poorest countries in the world. In many parts of the world today, farmers’ and workers’ capacity to do their jobs is limited by their caloric intake.
Note that there is a maximum amount of work that would allow her barely to survive (because of the calories she burns up working). As we saw in Unit 2, throughout human history people crossed the survival threshold when the population outran the food supply. This is the logic of the Malthusian population trap. The productivity of labour placed a limit on how large the population could be.
Exercise 5.5 Changing conditions for production
Using Figure 5.4, explain how you would represent the effects of each of the following:
- an improvement in growing conditions such as more adequate rainfall
- Angela having access to half the land that she had previously
- the availability to Angela of a better designed hoe making it physically easier to do the work of farming.
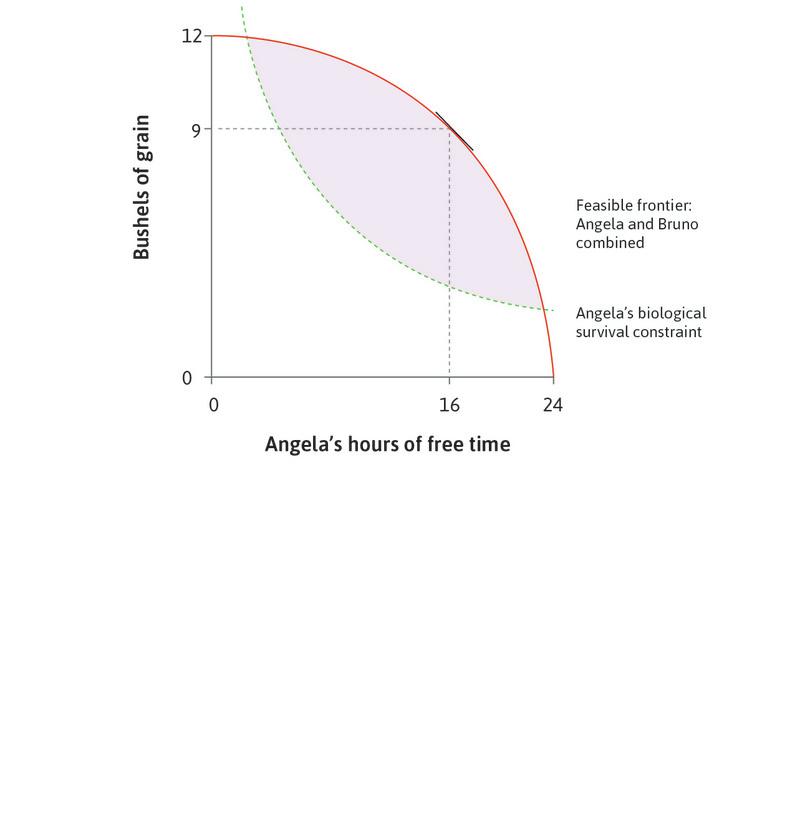
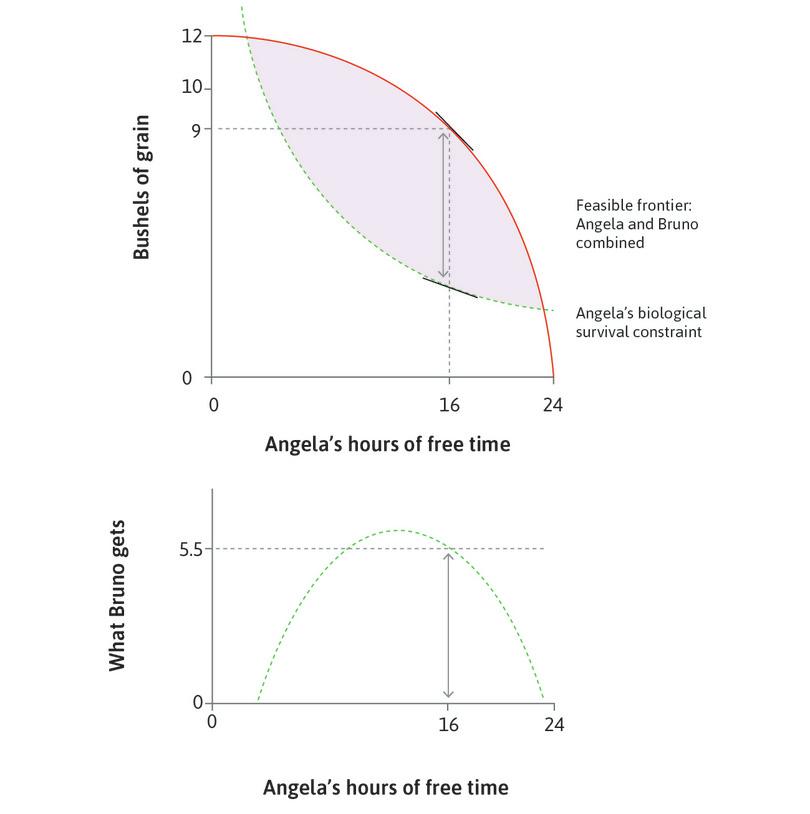
In Angela’s case, it is not only the limited productivity of her labour that might jeopardize her survival, but also how much of what she produces is taken by Bruno. If Angela could consume everything she produced (the height of the feasible frontier) and choose her hours of work, her survival would not be in jeopardy since the biological survival constraint is below the feasible frontier for a wide range of working hours. The question of biological feasibility arises because of Bruno’s claims on her output.
In Figure 5.4, the boundaries of the feasible solutions to the allocation problem are formed by the feasible frontier and the biological survival constraint. This lens-shaped shaded area gives the technically possible outcomes. We can now ask what will actually happen—which allocation will occur, and how does this depend on the institutions governing Bruno’s and Angela’s interaction?
Question 5.3 Choose the correct answer(s)
Figure 5.4 shows Angela and Bruno’s feasible frontier, and Angela’s biological survival constraint.
Based on this figure, which of the following is correct?
- At 24 hours of work (or 0 hours of free time), Angela’s biological survival constraint is above the feasible frontier. This means that at this point she cannot produce enough grain to survive.
- Angela would not produce any grain if she did not work. This is not technically feasible because she needs 2.5 bushels of grain to survive.
- Technology that boosted grain production would increase the amount of grain that could be produced for any given number of working hours, shifting the feasible frontier up and thus expanding the technically feasible set.
- If Angela did not need as much grain to survive, the biological survival constraint would be lower, making the technically feasible set bigger.
5.6 Allocations imposed by force
With the help of his gun, Bruno can choose any point in the lens-shaped technically feasible set of allocations. But which will he choose?
He reasons like this:
- Bruno
- For any number of hours that I order Angela to work, she will produce the amount of grain shown by the feasible frontier. But I’ll have to give her at least the amount shown by the biological survival constraint for that much work, so that I can continue to exploit her. I get to keep the difference between what she produces and what I give her. Therefore I should find the hours of Angela’s work for which the vertical distance between the feasible frontier and the biological survival constraint (Figure 5.5) is the greatest.
- economic rent
- A payment or other benefit received above and beyond what the individual would have received in his or her next best alternative (or reservation option). See also: reservation option.
The amount that Bruno will get if he implements this strategy is his economic rent, meaning the amount he gets over what he would get if Angela were not his slave (which, in this model, we set at zero).
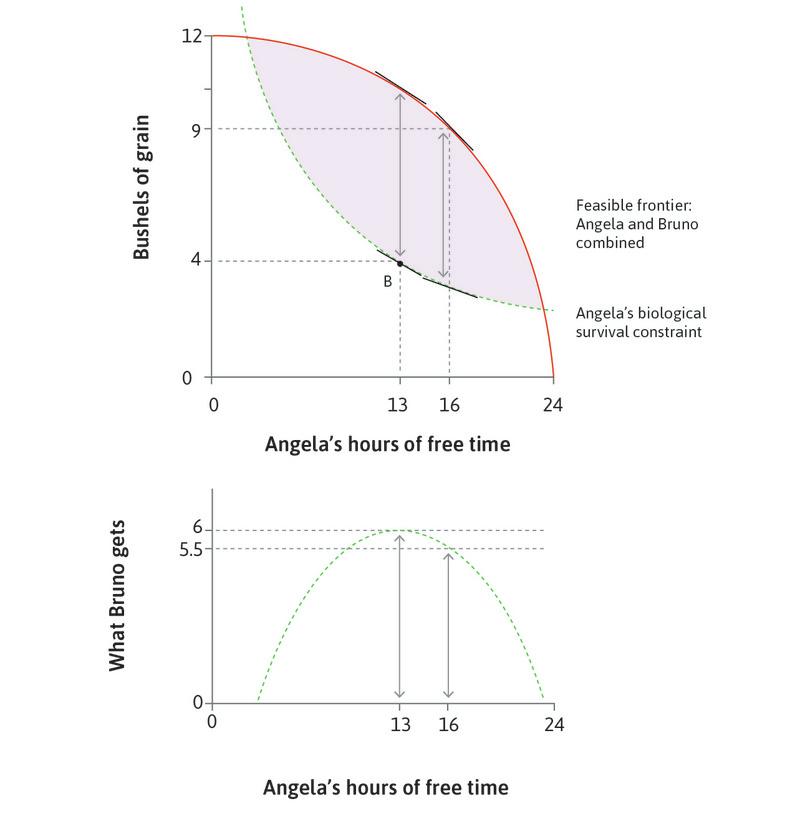
Bruno first considers letting Angela continue to work 8 hours a day, producing 9 bushels, as she did when she had free access to the land. For 8 hours of work she needs 3.5 bushels of grain to survive. So Bruno could take 5.5 bushels without jeopardizing his future opportunities to benefit from Angela’s labour.
Bruno is studying Figure 5.5 and asks for your help. You have noticed that the MRS on the survival constraint is less than the MRT at 8 hours of work:
- You
- Bruno, your plan cannot be right. If you forced her to work a little more, she’d only need a little more grain to have the energy to work longer, because the biological survival constraint is relatively flat at 8 hours of work. But the feasible frontier is steep, so she would produce a lot more if you imposed longer hours.
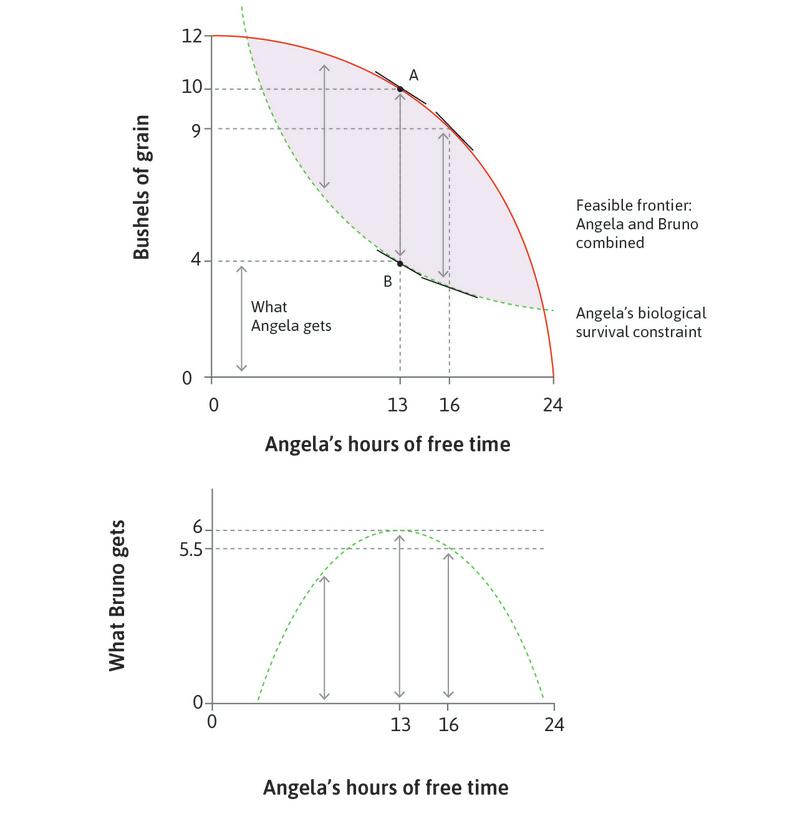
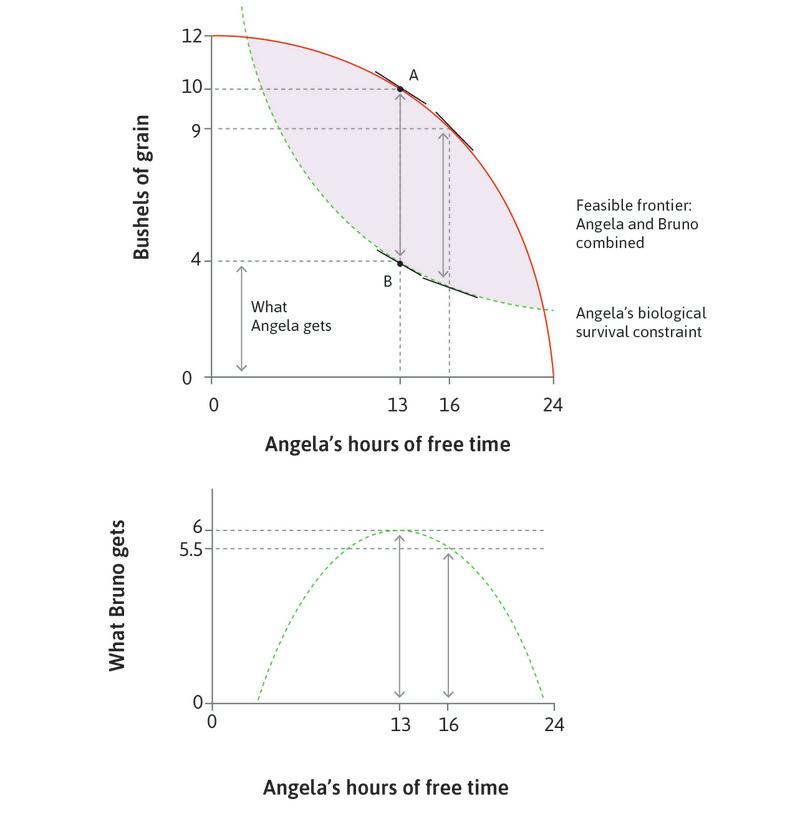
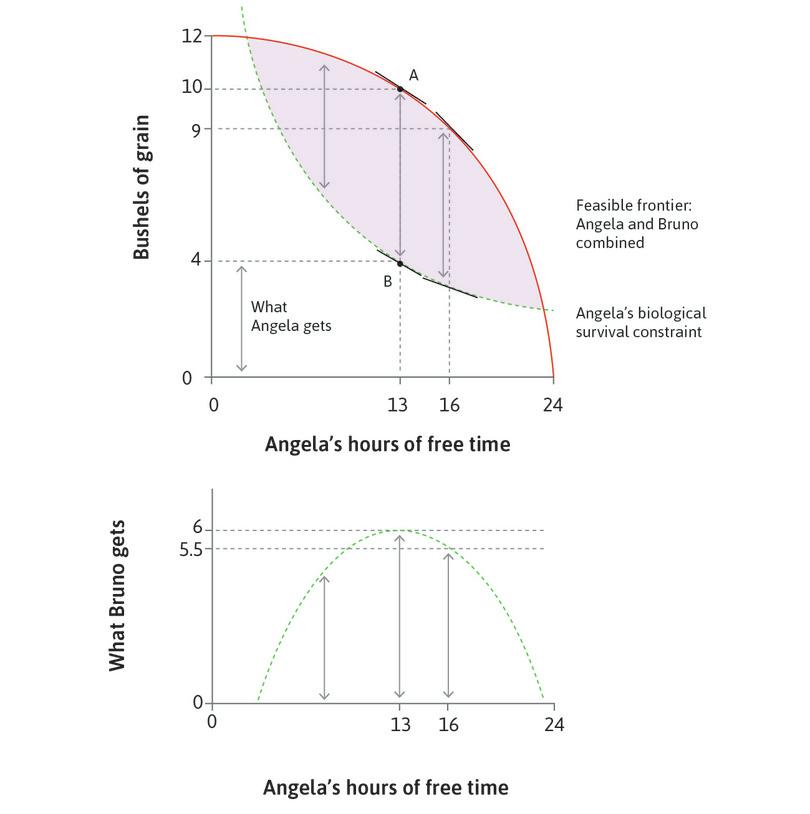
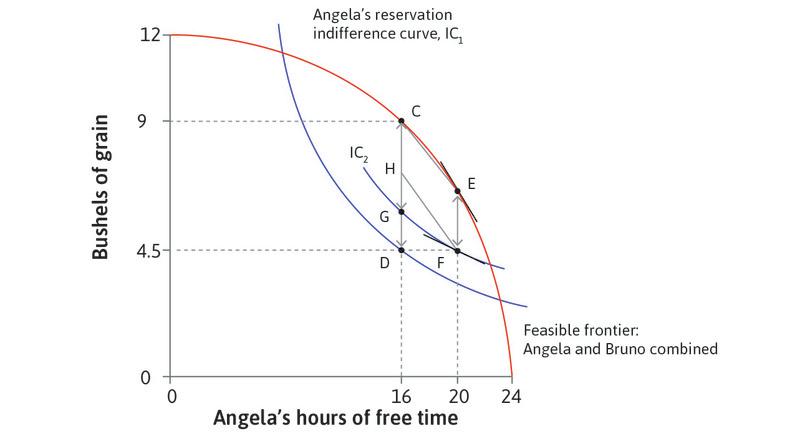
You demonstrate the argument to him using the analysis in Figure 5.5, which indicates that the vertical distance between the feasible frontier and the biological survival constraint is the greatest when Angela works for 11 hours. If Bruno commands Angela to work for 11 hours, then she will produce 10 bushels and Bruno will get to keep 6 bushels for himself. We can use Figure 5.5 to find out how many bushels of grain Bruno will get for any technically feasible allocation.
The lower panel in the last step in Figure 5.5 shows how the amount Bruno can take varies with Angela’s free time. The graph is hump-shaped, and peaks at 13 hours of free time and 11 hours of work. Bruno maximizes his amount of grain at allocation B, commanding Angela to work for 11 hours.
Notice how the slopes of the feasible frontier and the survival constraint (the MRT and MRS) help us to find the number of hours where Bruno can take the maximum amount of grain. To the right of 13 hours of free time (that is, if Angela works less than 11 hours) the biological survival constraint is flatter than the feasible frontier (MRS < MRT). This means that working more hours (moving to the left) would produce more grain than what Angela needs for the extra work. To the left of 13 hours of free time (Angela working more), the reverse is true: MRS > MRT. Bruno’s economic rent is greatest at the hours of work where the slopes of the two frontiers are equal.
That is:
Question 5.4 Choose the correct answer(s)
Figure 5.5 shows Angela and Bruno’s feasible frontier, and Angela’s biological survival constraint.
If Bruno can impose the allocation:
- At the technically feasible point where Angela produces the most grain, she needs all of the grain to survive, so there would be none for Bruno.
- The distance between the feasible frontier and Angela’s survival constraint, and thus Bruno’s share, is maximized where MRS = MRT.
- At 8 hours of work (16 hours of free time) the feasible frontier is steeper than the biological survival constraint. Thus MRT > MRS.
- Bruno would indeed choose 13 hours of free time for Angela, but the maximum he can claim without making Angela unable to work is 6 bushels of grain: the vertical distance between the feasible frontier and the survival constraint.
New institutions: Law and private property
- private property
- The right and expectation that one can enjoy one’s possessions in ways of one’s own choosing, exclude others from their use, and dispose of them by gift or sale to others who then become their owners.
The economic interaction described in this section takes place in an environment where Bruno has the power to enslave Angela. If we move from a scenario of coercion to one in which there is a legal system that prohibits slavery and protects private property and the rights of landowners and workers, we can expect the outcome of the interaction to change.
- power
- The ability to do (and get) the things one wants in opposition to the intentions of others, ordinarily by imposing or threatening sanctions.
In Unit 1, we defined private property as the right to use and exclude others from the use of something, and the right to sell it (or to transfer these rights to others). From now on we will suppose that Bruno owns the land and can exclude Angela if he chooses. How much grain he will get as a result of his private ownership of the land will depend on the extent of his power over Angela in the new situation.
- economic rent
- A payment or other benefit received above and beyond what the individual would have received in his or her next best alternative (or reservation option). See also: reservation option.
- gains from exchange
- The benefits that each party gains from a transaction compared to how they would have fared without the exchange. Also known as: gains from trade. See also: economic rent.
When people participate voluntarily in an interaction, they do so because they expect the outcome to be better than their reservation option—the next-best alternative. In other words, they do so in pursuit of economic rents. Economic rents are also sometimes called gains from exchange, because they are how much a person gains by engaging in the exchange compared to not engaging.
- surplus, joint
- The sum of the economic rents of all involved in an interaction. Also known as: total gains from exchange or trade.
- bargaining power
- The extent of a person’s advantage in securing a larger share of the economic rents made possible by an interaction.
The sum of the economic rents is termed the surplus (or sometimes the joint surplus, to emphasize that it includes all of the rents). How much rent they will each get—how they will share the surplus—depends on their bargaining power. And that, as we know, depends on the institutions governing the interaction.
In the example above, Angela was forced to participate and Bruno chose her working hours to maximize his own economic rent. Next we look at the situation where she can simply say no. Angela is no longer a slave, but Bruno still has the power to make a take-it-or-leave-it offer, just like the Proposer in the ultimatum game.
5.7 Economically feasible allocations and the surplus
We check back on Angela and Bruno, and immediately notice that Bruno is now wearing a suit, and is no longer armed. He explains that this is no longer needed because there is now a government with laws administered by courts, and professional enforcers called the police. Bruno now owns the land, and Angela must have permission to use his property. He can offer a contract allowing her to farm the land, and give him part of the harvest in return. But the law requires that exchange is voluntary: Angela can refuse the offer.
- Bruno
- It used to be a matter of power, but now both Angela and I have property rights: I own the land, and she owns her own labour. The new rules of the game mean that I can no longer force Angela to work. She has to agree to the allocation that I propose.
- You
- And if she doesn’t?
- Bruno
- Then there is no deal. She doesn’t work on my land, I get nothing, and she gets barely enough to survive from the government.
- You
- So you and Angela have the same amount of power?
- Bruno
- Certainly not! I am the one who gets to make a take-it-or-leave-it offer. I am like the Proposer in the ultimatum game, except that this is no game. If she refuses she goes hungry.
- You
- But if she refuses you get zero?
- Bruno
- That will never happen.
Why does he know this? Bruno knows that Angela, unlike the subjects in the ultimatum game experiments, is entirely self-interested (she does not punish an unfair offer). If he makes an offer that is just a tiny bit better for Angela than not working at all and getting subsistence rations, she will accept it.
Now he asks you a question similar to the one he asked earlier:
- Bruno
- In this case, what should my take-it-or-leave-it offer be?
You answered before by showing him the biological survival constraint. Now the limitation is not Angela’s survival, but rather her agreement. You know that she values her free time, so the more hours he offers her to work, the more he is going to have to pay.
- You
- Why don’t you just look at Angela’s indifference curve that passes through the point where she does not work at all and barely survives? That will tell you how much is the least you can pay her for each of the hours of free time she would give up to work for you.
- reservation option
- A person’s next best alternative among all options in a particular transaction. Also known as: fallback option. See also: reservation price.
- reservation indifference curve
- A curve that indicates allocations (combinations) that are as highly valued as one’s reservation option.
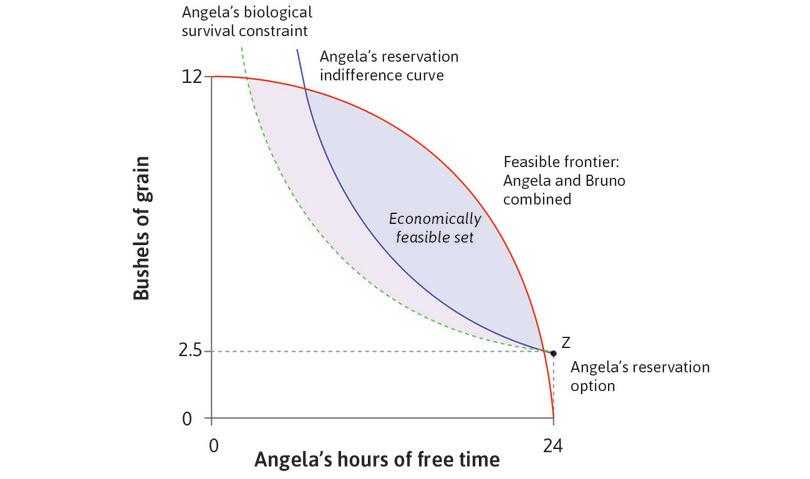
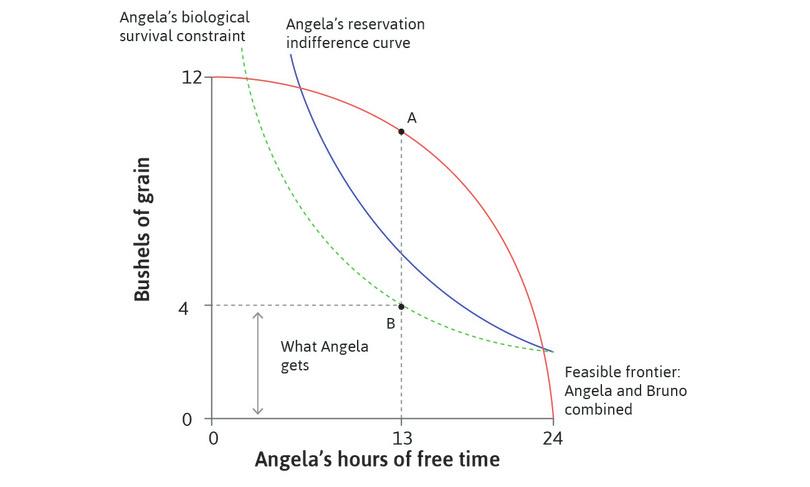
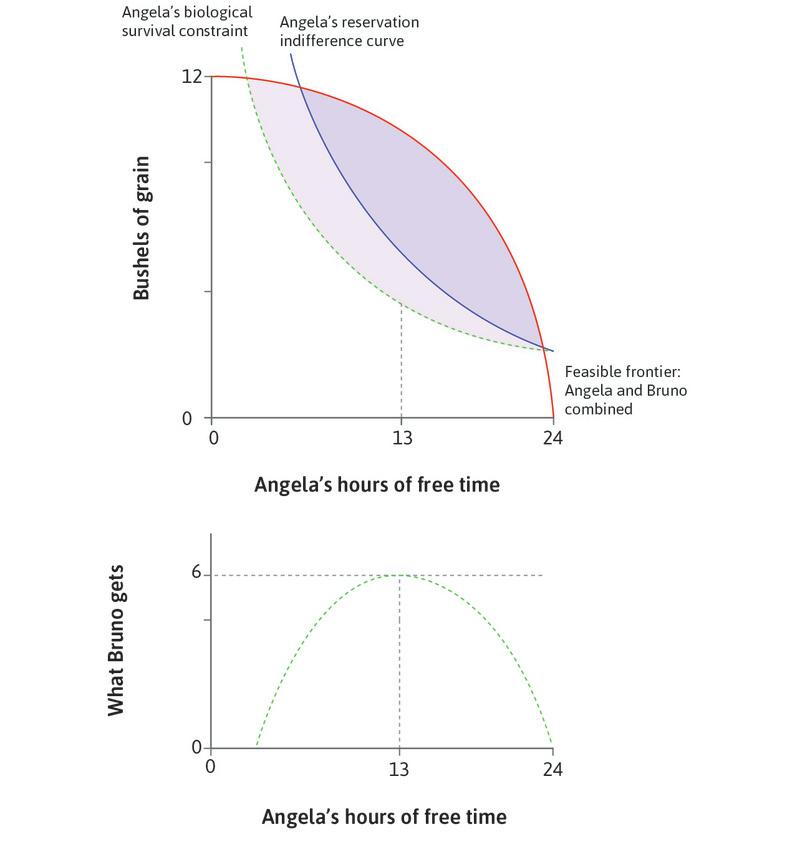
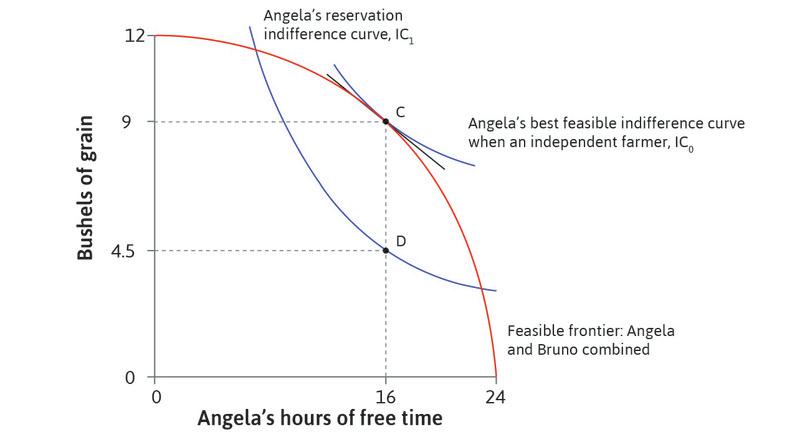
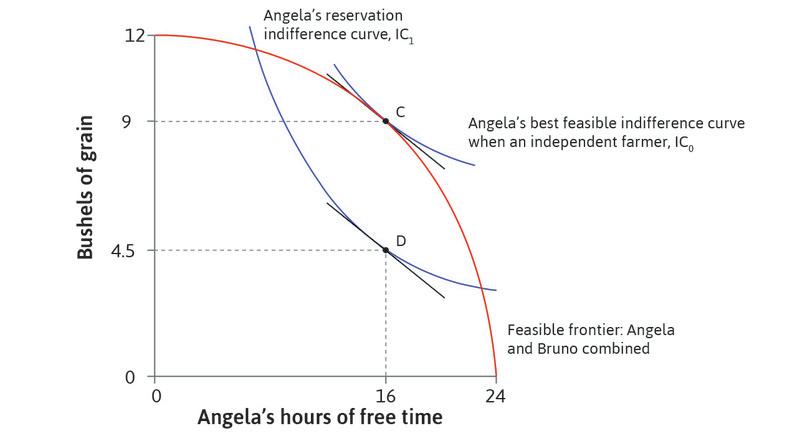
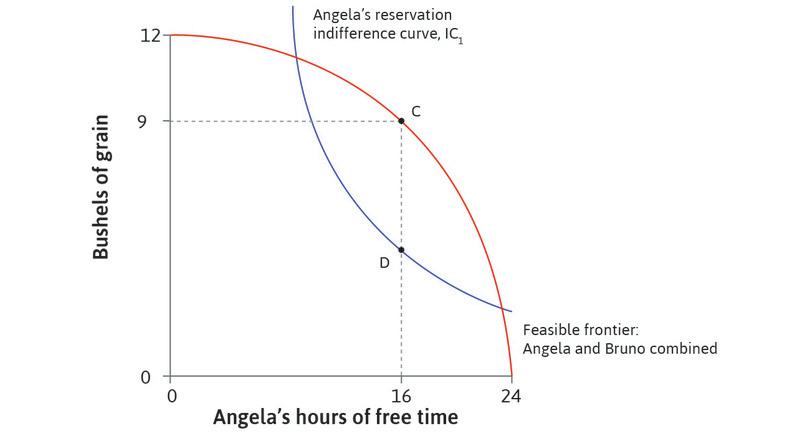
Point Z in Figure 5.6 is the allocation in which Angela does no work and gets only survival rations (from the government, or perhaps her family). This is her reservation option: if she refuses Bruno’s offer, she has this option as a backup. Follow the steps in Figure 5.6 to see Angela’s reservation indifference curve: all of the allocations that have the same value for her as the reservation option. Below or to the left of the curve she is worse off than in her reservation option. Above and to the right she is better off.
The set of points bounded by the reservation indifference curve and the feasible frontier is the set of all economically feasible allocations, now that Angela has to agree to the proposal that Bruno makes. Bruno thanks you for this handy new tool for figuring out the most he can get from Angela.
The biological survival constraint and the reservation indifference curve have a common point (Z): at that point, Angela does no work and gets subsistence rations from the government. Other than that, the two curves differ. The reservation indifference curve is uniformly above the biological survival constraint. The reason, you explain to Bruno, is that however hard she works along the survival constraint, she barely survives; and the more she works the less free time she has, so the unhappier she is. Along the reservation indifference curve, by contrast, she is just as well off as at her reservation option, meaning that being able to keep more of the grain that she produces compensates exactly for her lost free time.
Exercise 5.6 Biological and economic feasibility
Using Figure 5.6:
- Explain why a point on the biological survival constraint is higher (more grain is required) when Angela has fewer hours of free time. Why does the curve also get steeper when she works more?
- Explain why the biologically feasible set is not equal to the economically feasible set.
- Explain (by shifting the curves) what happens if a more nutritious kind of grain is available for Angela to grow and consume.
We can see that both Angela and Bruno may benefit if a deal can be made. Their exchange—allowing her to use his land (that is, not using his property right to exclude her) in return for her sharing some of what she produces—makes it possible for both to be better off than if no deal had been struck.
- As long as Bruno gets some of the crop he will do better than if there is no deal.
- As long as Angela’s share makes her better off than she would have been if she took her reservation option, taking account of her work hours, she will also benefit.
This potential for mutual gain is why their exchange need not take place at the point of a gun, but can be motivated by the desire of both to be better off.
- Pareto improvement
- A change that benefits at least one person without making anyone else worse off. See also: Pareto dominant.
All of the allocations that represent mutual gains are shown in the economically feasible set in Figure 5.6. Each of these allocations Pareto-dominates the allocation that would occur without a deal. In other words, Bruno and Angela could achieve a Pareto improvement.
This does not mean that both parties will benefit equally. If the institutions in effect give Bruno the power to make a take-it-or-leave-it offer, subject only to Angela’s agreement, he can capture the entire surplus (minus the tiny bit necessary to get Angela to agree). Bruno knows this already.
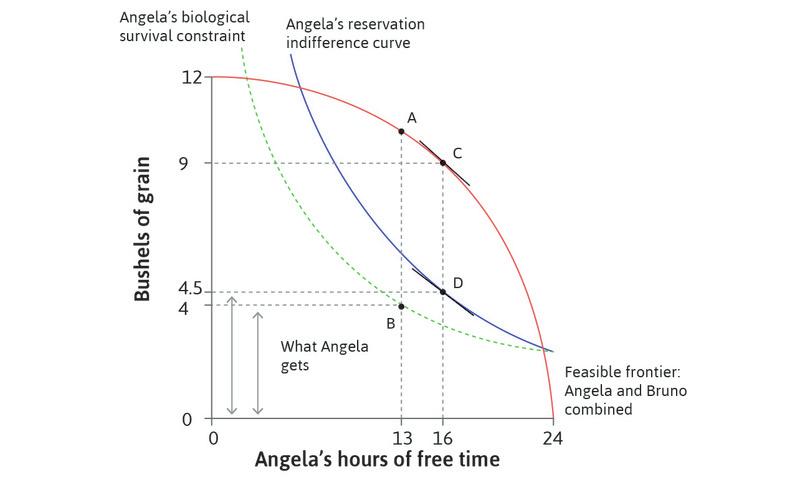
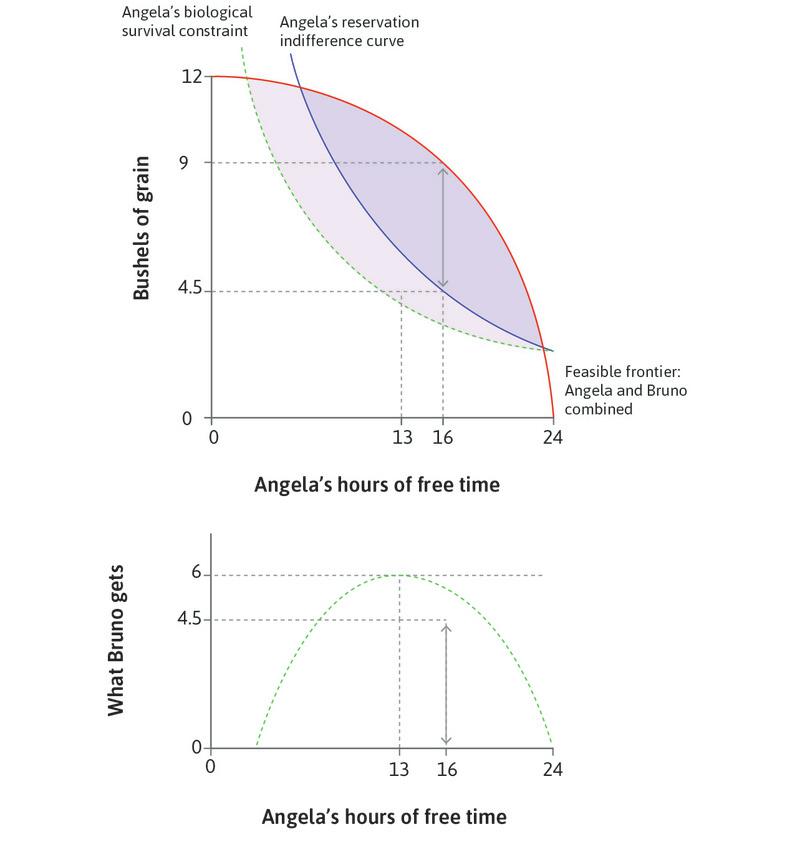
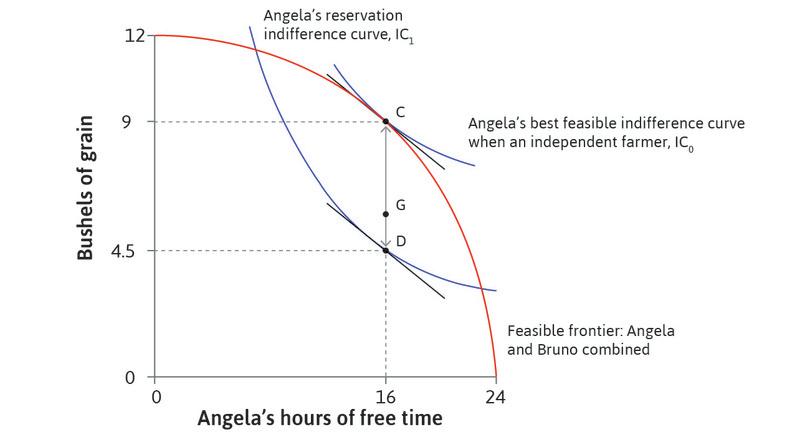
Once you have explained the reservation indifference curve to him, Bruno knows which allocation he wants. He maximizes the amount of grain he can get at the maximum height of the lens-shaped region between Angela’s reservation indifference curve and the feasible frontier. This will be where the MRT on the feasible frontier is equal to the MRS on the indifference curve. Figure 5.7a shows that this allocation requires Angela to work for fewer hours than she did under coercion.
So Bruno would like Angela to work for 8 hours and give him 4.5 bushels of grain (allocation D). How can he implement this allocation? All he has to do is to make a take-it-or-leave-it offer of a contract allowing Angela to work the land, in return for a land rent of 4.5 bushels per day. (This is a sharecropping contract, in which a landowner allows a farmer to use land in return for a share of the crop.) If Angela has to pay 4.5 bushels (CD in Figure 5.7a) then she will choose to produce at point C, where she works for 8 hours. You can see this in the figure; if she produced at any other point on the feasible frontier and then gave Bruno 4.5 bushels, she would have lower utility—she would be below her reservation indifference curve. But she can achieve her reservation utility by working for 8 hours, so she will accept the contract.
Exercise 5.7 Why Angela works for 8 hours
Angela’s income is the amount she produces minus the land rent she pays to Bruno.
- Using Figure 5.7a, suppose Angela works 11 hours. Would her income (after paying land rent) be greater or less than when she works 8 hours? Suppose instead, she works 6 hours, how would her income compare with when she works 8 hours?
- Explain in your own words why she will choose to work 8 hours.
Since Angela is on her reservation indifference curve, only Bruno benefits from this exchange. All of the joint surplus goes to Bruno. His economic rent (equal to the land rent she pays him) is the surplus.
Remember that when Angela could work the land on her own she chose allocation C. Notice now that she chooses the same hours of work when she has to pay rent. Why does this happen? However much rent Angela has to pay, she will choose her hours of work to maximize her utility, so she will produce at a point on the feasible frontier where the MRT is equal to her MRS. And we know that her preferences are such that her MRS doesn’t change with the amount of grain she consumes, so it will not be affected by the rent. This means that if she can choose her hours, she will work for 8 hours irrespective of the land rent (as long as this gives her at least her reservation utility).
Leibniz: Angela’s choice of working hours when she pays rent
Figure 5.7b shows how the surplus (which Bruno gets) varies with Angela’s hours. You will see that the surplus falls as Angela works more or less than 8 hours. It is hump-shaped, like Bruno’s rent in the case of coercion. But the peak is lower when Bruno needs Angela to agree to the proposal.
Exercise 5.8 Take it or leave it?
- Why is it Bruno, and not Angela, who has the power to make a take-it-or-leave-it offer?
- Can you imagine a situation in which the farmer, not the landowner, might have this power?
Question 5.5 Choose the correct answer(s)
Figure 5.6 shows Angela and Bruno’s feasible frontier, Angela’s biological survival constraint, and her reservation indifference curve.
Based on this figure, which of the following is correct?
- The economically feasible set is the area between the reservation indifference curve and the feasible frontier. This is smaller than the technically feasible set, which is the area between the biological survival constraint and the feasible frontier.
- The reservation indifference curve is steeper than the biological survival constraint, in other words the MRS is larger on the former than on the latter.
- A point cannot be economically feasible if it is not technically feasible. The figure shows that the economically feasible set lies inside the technically feasible set.
- When the ration is 2 bushels, Angela’s reservation option is Z = (24, 2). If it increases to 3 bushels, her reservation option is (24, 3), which is on a higher indifference curve that lies above the survival constraint at all points. This will now be her reservation indifference curve.
Question 5.6 Choose the correct answer(s)
Figure 5.7a shows Angela and Bruno’s feasible frontier, Angela’s biological survival constraint and her reservation indifference curve. B is the outcome under coercion, while D is the outcome under voluntary exchange when Bruno makes a take-it-or-leave-it offer.
Looking at this graph, we can conclude that:
- Bruno’s reservation option is to receive nothing. Under voluntary exchange, Bruno receives the whole of the surplus: the amount in excess of what Angela needs to either survive or be willing to work. So this is his economic rent.
- Bruno’s amount of grain is the distance AB under coercion, and CD under voluntary exchange. So he is better off under coercion.
- Bruno offers an allocation Angela is only just willing to accept. She is indifferent between D and her reservation option, so her rent is zero.
- Angela will have more hours of free time at D under voluntary exchange than under coercion.
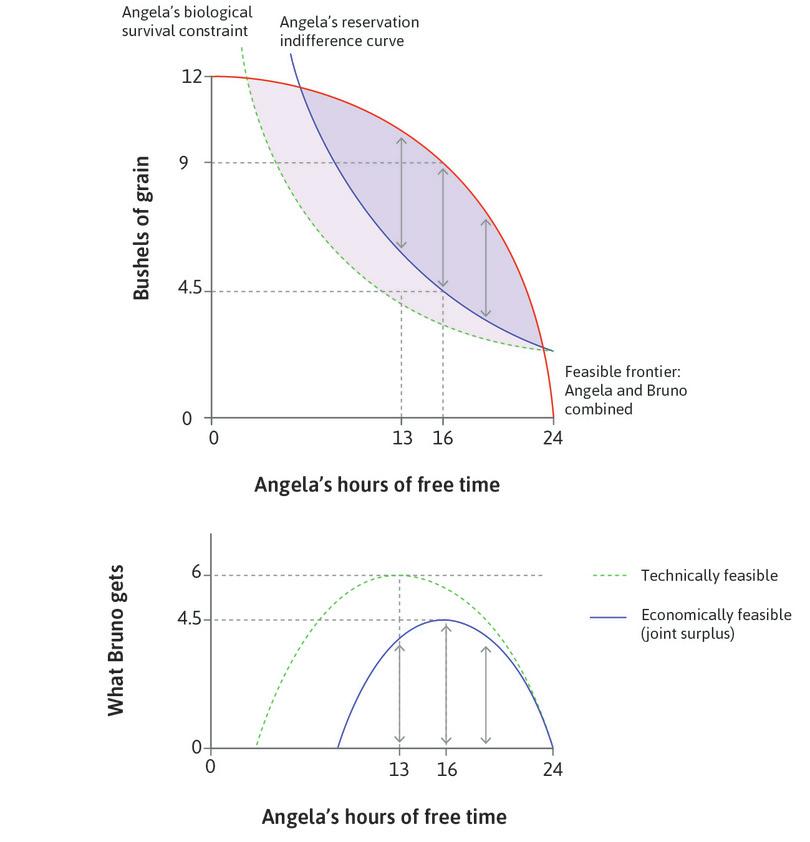
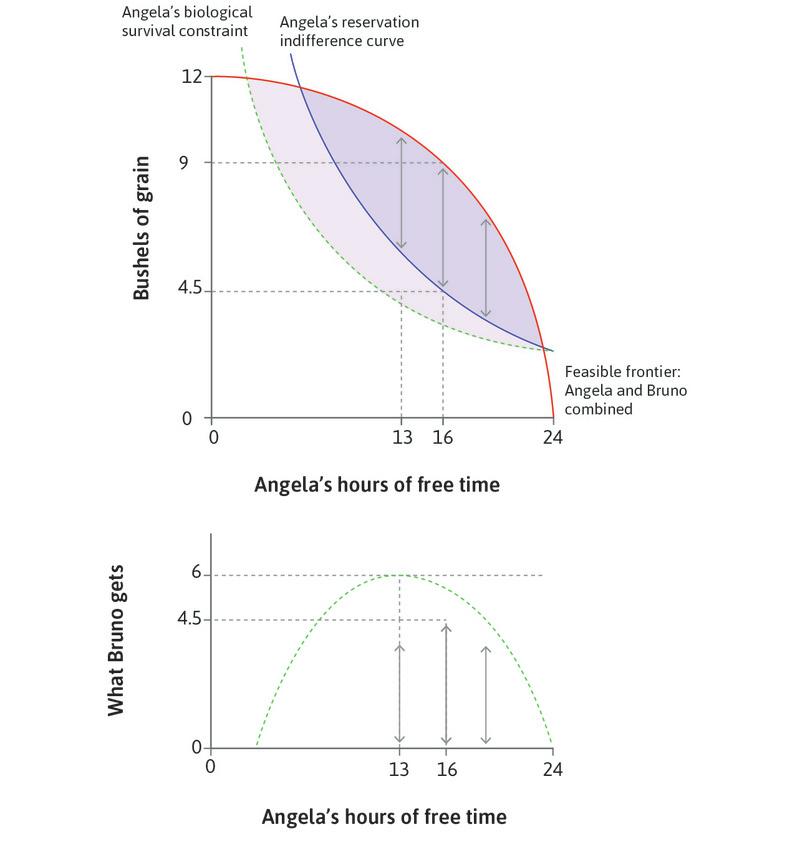
5.8 The Pareto efficiency curve and the distribution of the surplus
Angela chose to work for 8 hours, producing 9 bushels of grain, both when she had to pay rent, and also when she did not. In both cases there is a surplus of 4.5 bushels: the difference between the amount of grain produced, and the amount that would give Angela her reservation utility.
The two cases differ in who gets the surplus. When Angela had to pay land rent, Bruno took the whole surplus, but when she could work the land for herself she obtained all of the surplus. Both allocations have two important properties:
- All the grain produced is shared between Angela and Bruno.
- The MRT on the feasible frontier is equal to the MRS on Angela’s indifference curve.
This means that the allocations are Pareto efficient.
To see why, remember that Pareto efficiency means that no Pareto improvement is possible: it is impossible to change the allocation to make one party better off without making the other worse off.
The first property is straightforward: it means that no Pareto improvement can be achieved simply by changing the amounts of grain they each consume. If one consumed more, the other would have to have less. On the other hand, if some of the grain produced was not being consumed, then consuming it would make one or both of them better off.
The second property, MRS = MRT, means that no Pareto improvement can be achieved by changing Angela’s hours of work and hence the amount of grain produced.
If the MRS and MRT were not equal, it would be possible to make both better off. For example, if MRT > MRS, Angela could transform an hour of her time into more grain than she would need to get the same utility as before, so the extra grain could make both of them better off. But if MRT = MRS, then any change in the amount of grain produced would only be exactly what is needed to keep Angela’s utility the same as before, given the change in her hours.
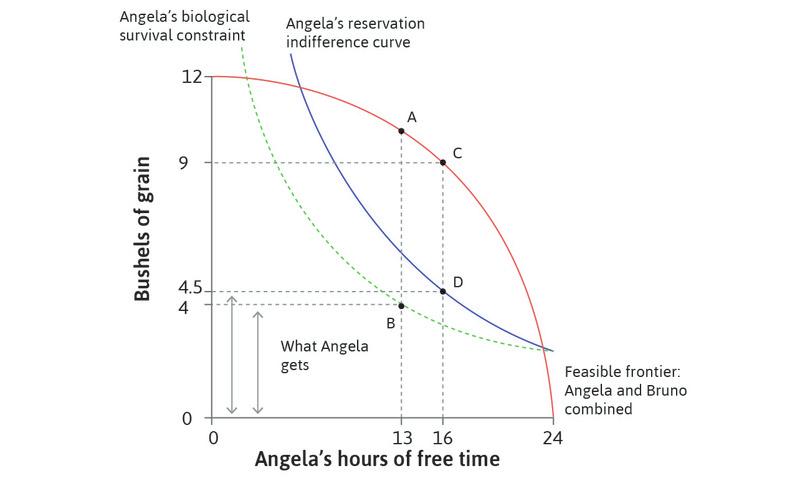
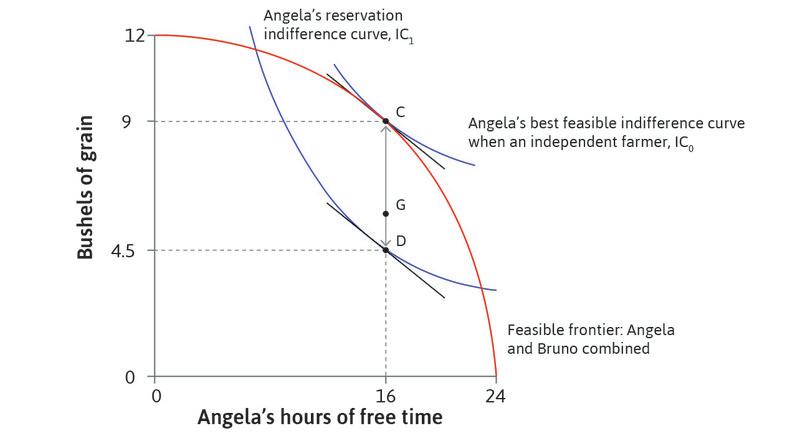
Figure 5.8 shows that there are many other Pareto-efficient allocations in addition to these two. Point C is the outcome when Angela is an independent farmer. Compare the analysis in Figure 5.8 with Bruno’s take-or-leave-it offer, and see the other Pareto-efficient allocations.
Pareto efficiency and the Pareto efficiency curve
- A Pareto-efficient allocation has the property that there is no alternative technically feasible allocation in which at least one person would be better off, and nobody worse off.
- The set of all such allocations is the Pareto efficiency curve. It is also referred to as the contract curve.
Figure 5.8 shows that in addition to the two Pareto-efficient allocations we have observed (C and D), every point between C and D represents a Pareto-efficient allocation. CD is called the Pareto efficiency curve: it joins together all the points in the feasible set for which MRS = MRT. (You will also hear it called the contract curve, even in situations where there is no contract, which is why we prefer the more descriptive term Pareto efficiency curve.)
Leibniz: The Pareto efficiency curve
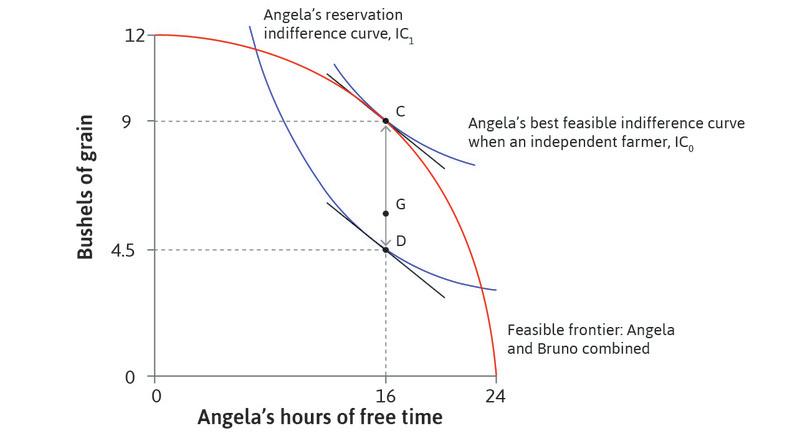
At each allocation on the Pareto efficiency curve Angela works for 8 hours and there is a surplus of 4.5 bushels, but the distribution of the surplus is different—ranging from point D where Angela gets none of it, to point C where she gets it all. At the hypothetical allocation G, both receive an economic rent: Angela’s rent is GD, Bruno’s is GC, and the sum of their rents is equal to the surplus.
Question 5.7 Choose the correct answer(s)
Figure 5.8 shows the Pareto efficiency curve CD for the interaction between Angela and Bruno.
Which of the following statements is correct?
- All points on CD are Pareto efficient, so none of them is Pareto-dominated. (Comparing C and D, we see that Bruno prefers D and Angela prefers C.)
- The Pareto efficiency curve, by definition, joins all the economically feasible points where MRS = MRT.
- All the points on CD are Pareto efficient. It does not make any sense to say that one point on CD is more efficient than another.
- All the points on CD are Pareto efficient, but Bruno and Angela are not indifferent. Some points (like C) are better for Angela, while others (like D) are better for Bruno.
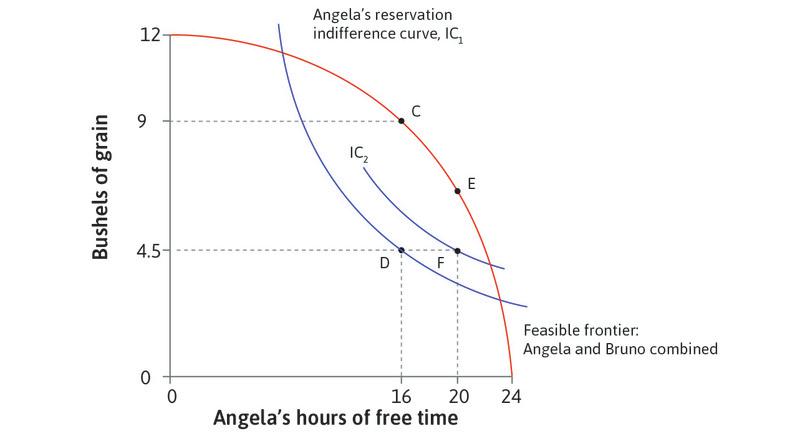
5.9 Politics: Sharing the surplus
Bruno thinks that the new rules, under which he makes an offer that Angela will not refuse, are not so bad after all. Angela is also better off than she had been when she had barely enough to survive. But she would like a share in the surplus.
She and her fellow farm workers lobby for a new law that limits working time to 4 hours a day, while requiring that total pay is at least 4.5 bushels. They threaten not to work at all unless the law is passed.
- Bruno
- Angela, you and your colleagues are bluffing.
- Angela
- No, we are not: we would be no worse off at our reservation option than under your contract, working the hours and receiving the small fraction of the harvest that you impose!
Angela and her fellow workers win, and the new law limits the working day to 4 hours.
How did things work out?
Before the short-hours law Angela worked for 8 hours and received 4.5 bushels of grain. This is point D in Figure 5.9. The new law implements the allocation in which Angela and her friends work 4 hours, getting 20 hours of free time and the same number of bushels. Since they have the same amount of grain and more free time, they are better off. Figure 5.9 shows they are now on a higher indifference curve.
The new law has increased Angela’s bargaining power and Bruno is worse off than before. You can see she is better off at F than at D. She is also better off than she would be with her reservation option, which means she is now receiving an economic rent.
Angela’s rent can be measured, in bushels of grain, as the vertical distance between her reservation indifference curve (IC1 in Figure 5.9) and the indifference curve she is able to achieve under the new legislation (IC2). We can think of the economic rent as:
- The maximum amount of grain per year that Angela would give up to live under the new law rather than in the situation before the law was passed.
- Or (because Angela is obviously political) the amount she would be willing to pay so that the law passed, for example by lobbying the legislature or contributing to election campaigns.
Question 5.8 Choose the correct answer(s)
In Figure 5.9, D and F are the outcomes before and after the introduction of a new law that limits Angela’s work time to four hours a day while requiring a minimum pay of 4.5 bushels. Based on this information, which of the following statements are correct?
- It is not a Pareto improvement, because Bruno is worse off (gets less grain) at F than at D.
- At outcome F, where Angela works for 4 hours, MRT > MRS (compare the slopes of the feasible frontier and indifference curve). So it cannot be Pareto efficient. (For example, Bruno could be better off, without making Angela worse off, if they could move to the left along IC2.)
- At F, Angela is above her reservation indifference curve and is thus receiving an economic rent. Bruno’s reservation option is to receive nothing, so the grain he receives at F is an economic rent for him.
- At D, Bruno obtained rent equal to CD, and Angela obtained no rent. At F his rent is much lower. The law has increased Angela’s bargaining power and reduced Bruno’s.
5.10 Bargaining to a Pareto-efficient sharing of the surplus
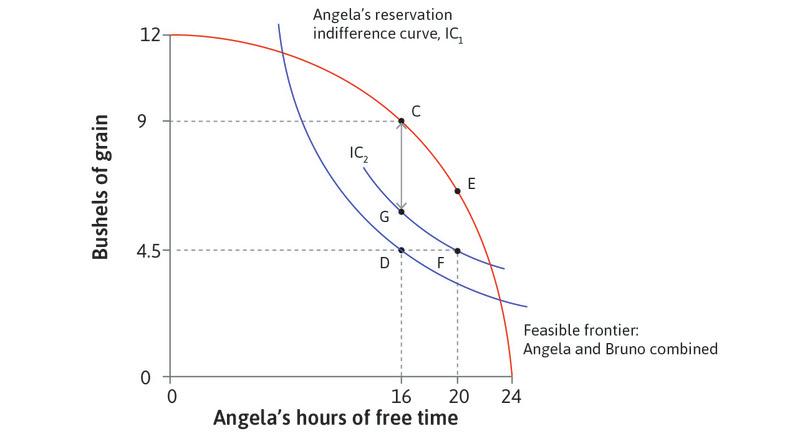
Angela and her friends are pleased with their success. She asks what you think of the new policy.
- Pareto efficiency curve
- The set of all allocations that are Pareto efficient. Often referred to as the contract curve, even in social interactions in which there is no contract, which is why we avoid the term. See also: Pareto efficient.
- You
- Congratulations, but your policy is far from the best you could do.
- Angela
- Why?
- You
- Because you are not on the Pareto efficiency curve! Under your new law, Bruno is getting 2 bushels, and cannot make you work more than 4 hours. So why don’t you offer to continue to pay him 2 bushels, in exchange for agreeing to let you keep anything you produce above that? Then you get to choose how many hours you work.
The small print in the law allows a longer work day if both parties agree, as long as the workers’ reservation option is a 4-hour day if no agreement is reached.
- You
- Now redraw Figure 5.9 and use the concepts of the joint surplus and the Pareto efficiency curve from Figure 5.8 to show Angela how she can get a better deal.
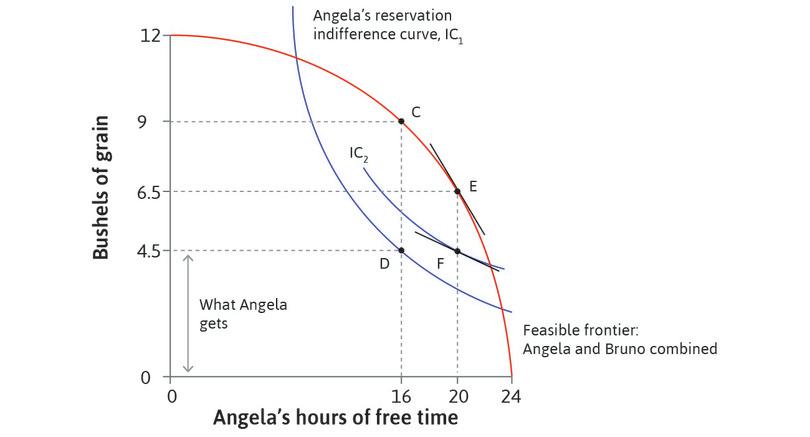
- You
- Look at Figure 5.10. The surplus is largest at 8 hours of work. When you work for 4 hours the surplus is smaller, and you pay most of it to Bruno. If you increase the surplus, you can pay him the same amount, and your own surplus will be bigger—so you will be better off. Follow the steps in Figure 5.10 to see how this works.
The move away from point D (at which Bruno had all the bargaining power and obtained all the gains from exchange) to point H where Angela is better off consists of two distinct steps:
-
From D to F, the outcome is imposed by new legislation. This was definitely not win-win: Bruno lost because his economic rent at F is less than the maximum feasible rent that he got at D. Angela benefitted.
-
Once at the legislated outcome, there were many win-win possibilities open to them. They are shown by the segment GH on the Pareto efficiency curve. Win-win alternatives to the allocation at F are possible by definition, because F was not Pareto efficient.
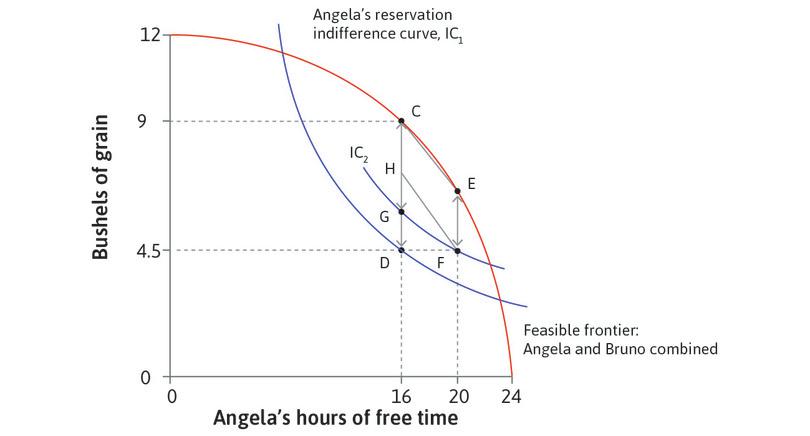
Bruno wants to negotiate. He is not happy with Angela’s proposal of H.
- Bruno
- I am no better off under this new plan than I would be if I just accepted the legislation that the farmers passed.
- You
- But Bruno, Angela now has bargaining power, too. The legislation changed her reservation option, so it is no longer 24 hours of free time at survival rations. Her reservation option is now the legislated allocation at point F. I suggest you make her a counter offer.
- Bruno
- Angela, I’ll let you work the land for as many hours as you choose if you pay me half a bushel more than EF.
They shake hands on the deal.
Because Angela is free to choose her work hours, subject only to paying Bruno the extra half bushel, she will work 8 hours where MRT = MRS. Because this deal lies between G and H, it is a Pareto improvement over point F. Moreover, because it is on the Pareto-efficient curve CD, we know there are no further Pareto improvements to be made. This is true of every other allocation on GH—they differ only in the distribution of the mutual gains, as some favour Angela while others favour Bruno. Where they end up will depend on their bargaining power.
Question 5.9 Choose the correct answer(s)
In Figure 5.10, Angela and Bruno are at allocation F, where she receives 3 bushels of grain for 4 hours of work.
From the figure, we can conclude that:
- Along EF, MRS < MRT. So EF is not Pareto efficient—there are other allocations where both would be better off.
- In area GHF, Angela is on a higher indifference curve than IC2, and Bruno has more grain than EF, so both are better off.
- Points on GD are Pareto efficient, but below G, Angela is on a lower indifference curve than at F, so she would be worse off.
- Points on GH are all Pareto efficient, but Bruno and Angela are not indifferent. He prefers points nearer to G, and she prefers points nearer to H.
5.11 Angela and Bruno: The moral of the story
Angela’s farming skills and Bruno’s ownership of land provided an opportunity for mutual gains from exchange.
The same is true when people directly exchange, or buy and sell, goods for money. Suppose you have more apples than you can consume, and your neighbour has an abundance of pears. The apples are worth less to you than to your neighbour, and the pears are worth more to you. So it must be possible to achieve a Pareto improvement by exchanging some apples and pears.
When people with differing needs, property and capacities meet, there is an opportunity to generate gains for all of them. That is why people come together in markets, online exchanges or pirate ships. The mutual gains are the pie—which we call the surplus.
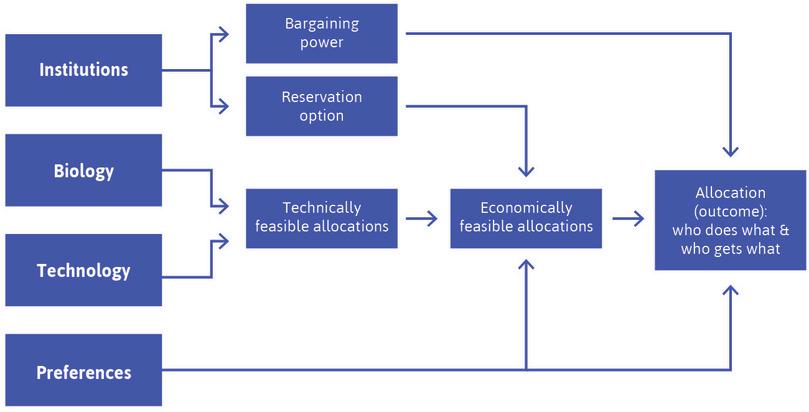
The allocations that we observe through history are largely the result of the institutions, including property rights and bargaining power, that were present in the economy. Figure 5.11 summarizes what we have learnt about the determination of economic outcomes from the succession of scenarios involving Angela and Bruno.
- Technology and biology determine whether or not they are able to mutually benefit, and the technically feasible set of allocations (Section 5.5). If Bruno’s land had been so unproductive that Angela’s labour could not produce enough to keep her alive, then there would have been no room for a deal.
- For allocations to be economically feasible, they must be Pareto improvements relative to the parties’ reservation options, which may depend on institutions (such as Angela’s survival rations from the government (Section 5.7) or legislation on working hours (Section 5.10)).
- The outcome of an interaction depends on people’s preferences (what they want), as well as the institutions that provide their bargaining power (ability to get it), and hence how the surplus is distributed (Section 5.10).
The story of Angela and Bruno provides three lessons about efficiency and fairness, illustrated by Figure 5.10, to which we will return in subsequent units.
- When one person or group has power to dictate the allocation, subject only to not making the other party worse off than in their reservation option, the powerful party will capture the entire surplus. If they have done this, then there cannot be any way to make either of them better off without making the other worse off (point D in the figure). So this must be Pareto efficient!
- Those who consider their treatment unfair often have some power to influence the outcome through legislation and other political means, and the result may be a fairer distribution in their eyes or ours, but may not necessarily be Pareto efficient (point F). Societies may face trade-offs between Pareto-efficient but unfair outcomes, and fair but Pareto-inefficient outcomes.
- If we have institutions under which people can jointly deliberate, agree on, and enforce alternative allocations, then it may be possible to avoid the trade-off and achieve both efficiency and fairness—as Angela and Bruno did through a combination of legislation and bargaining between themselves (point H).
5.12 Measuring economic inequality
In our analysis of the interaction between Angela and Bruno, we have assessed the allocations in terms of Pareto efficiency. We have seen that they (or at least one of them) can be better off if they can negotiate a move from a Pareto-inefficient allocation to one on the Pareto efficiency curve.
But the other important criterion for assessing an allocation is fairness. We know that Pareto-efficient allocations can be highly unequal. In the case of Angela and Bruno, inequality resulted directly from differences in bargaining power, but also from differences in their endowments: that is, what they each owned before the interaction (their initial wealth). Bruno owned land, while Angela had nothing except time and the capacity to work. Difference in endowments, as well as institutions, may in turn affect bargaining power.
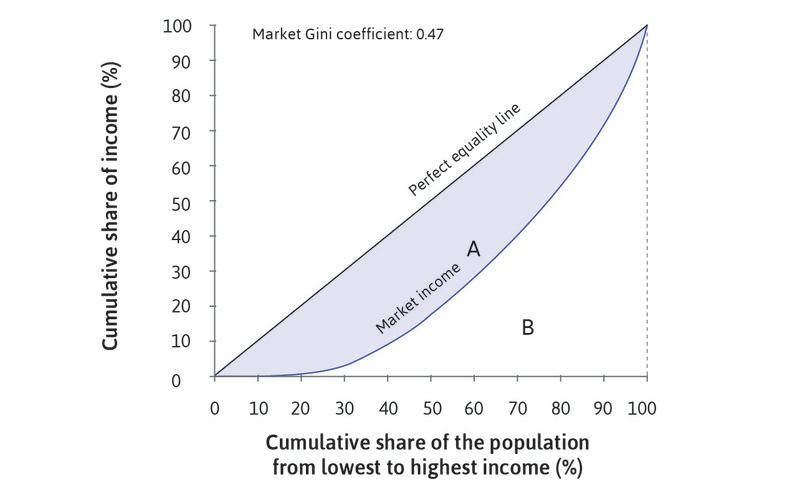
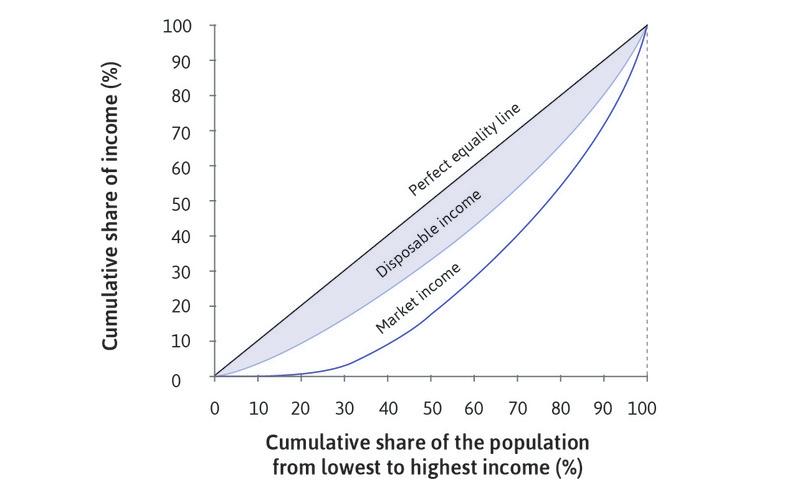
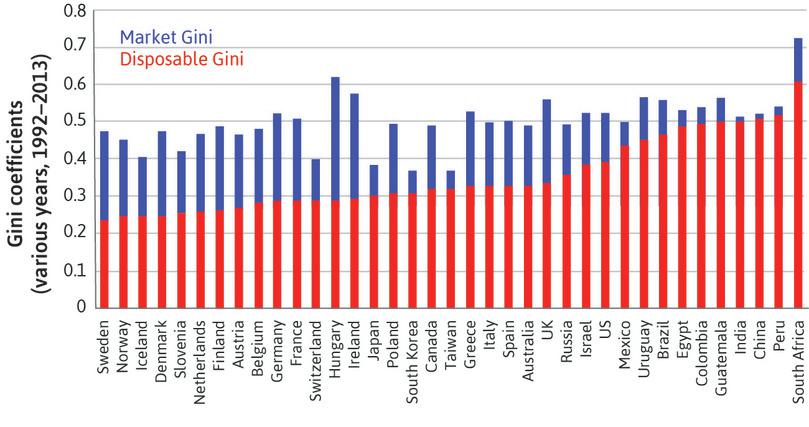
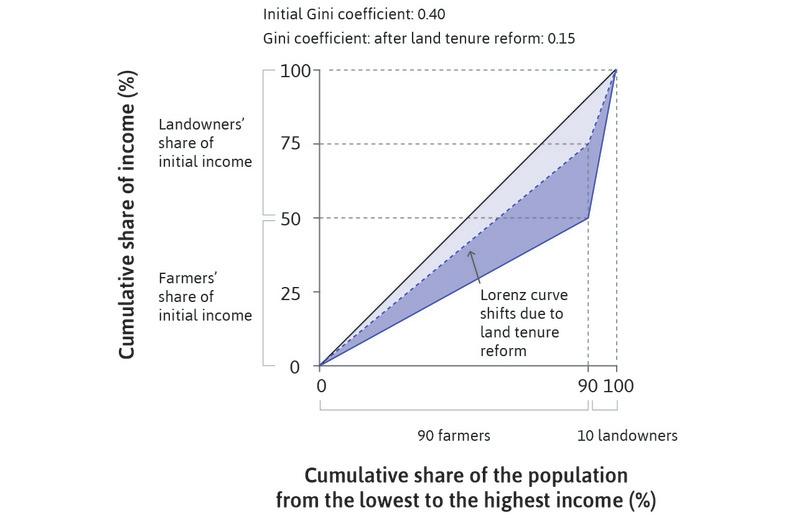
It is easy to assess the distribution between two people. But how can we assess inequalities in larger groups, or across a whole society? A useful tool for representing and comparing distributions of income or wealth, and showing the extent of inequality, is the Lorenz curve (invented in 1905 by Max Lorenz (1876–1959), an American economist, while he was still a student). It indicates how much disparity there is in income, or any other measure, across the population.4
- Lorenz curve
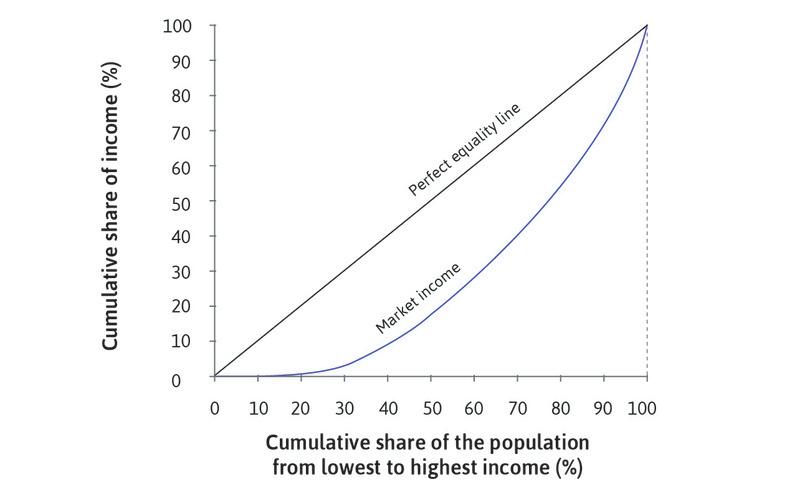
- A graphical representation of inequality of some quantity such as wealth or income. Individuals are arranged in ascending order by how much of this quantity they have, and the cumulative share of the total is then plotted against the cumulative share of the population. For complete equality of income, for example, it would be a straight line with a slope of one. The extent to which the curve falls below this perfect equality line is a measure of inequality. See also: Gini coefficient.
The Lorenz curve shows the entire population lined up along the horizontal axis from the poorest to the richest. The height of the curve at any point on the horizontal axis indicates the fraction of total income received by the fraction of the population given by that point on the horizontal axis.
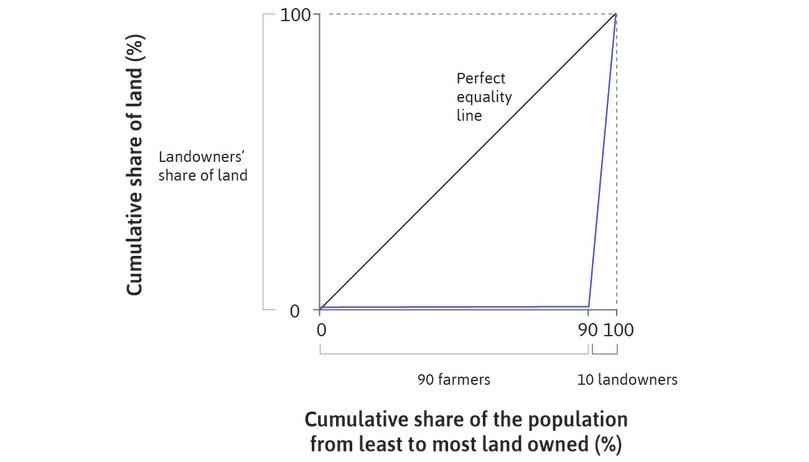
To see how this works, imagine a village in which there are 10 landowners, each owning 10 hectares, and 90 others who farm the land as sharecroppers, but who own no land (like Angela). The Lorenz curve is the blue line in Figure 5.12. Lining the population up in order of land ownership, the first 90% of the population own nothing, so the curve is flat. The remaining 10% own 10 hectares each, so the ‘curve’ rises in a straight line to reach the point where 100% of people own 100% of the land.
If instead each member of the population owned one hectare of land—perfect equality in land ownership—then the Lorenz curve would be a line at a 45-degree angle, indicating that the ‘poorest’ 10% of the population have 10% of the land, and so on (although in this case, everyone is equally poor, and equally rich).
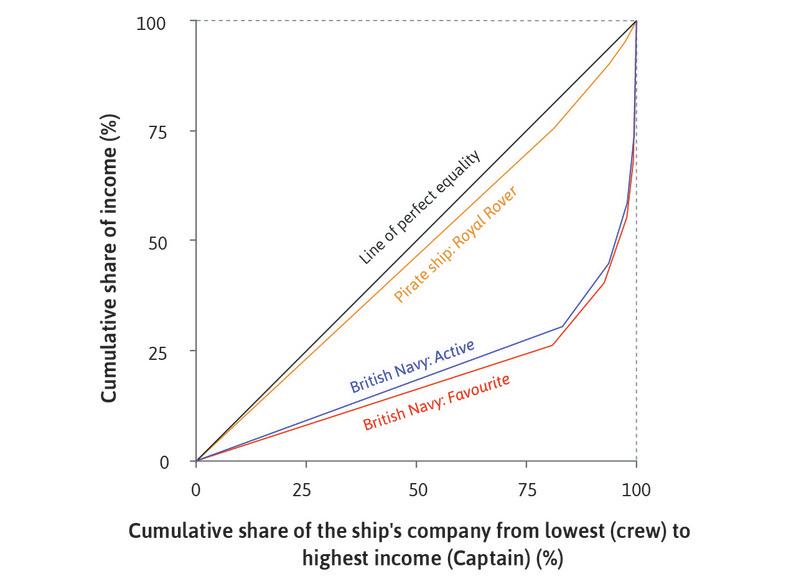
The Lorenz curve allows us to see how far a distribution departs from this line of perfect equality. Figure 5.13 shows the distribution of income that would have resulted from the prize-sharing system described in the articles of the pirate ship, the Royal Rover, discussed in the introduction to this unit. The Lorenz curve is very close to the 45-degree line, showing how the institutions of piracy allowed ordinary members of the crew to claim a large share of income.
In contrast, when the Royal Navy’s ships Favourite and Active captured the Spanish treasure ship La Hermione, the division of the spoils on the two British men-of-war ships was far less equal. The Lorenz curves show that ordinary crew members received about a quarter of the income, with the remainder going to a small number of officers and the captain. You can see that the Favourite was more unequal that the Active, with a lower share going to each crew member. By the standards of the day, pirates were unusually democratic and fair-minded in their dealings with each other.
The Gini coefficient
- Gini coefficient
- A measure of inequality of any quantity such as income or wealth, varying from a value of zero (if there is no inequality) to one (if a single individual receives all of it).
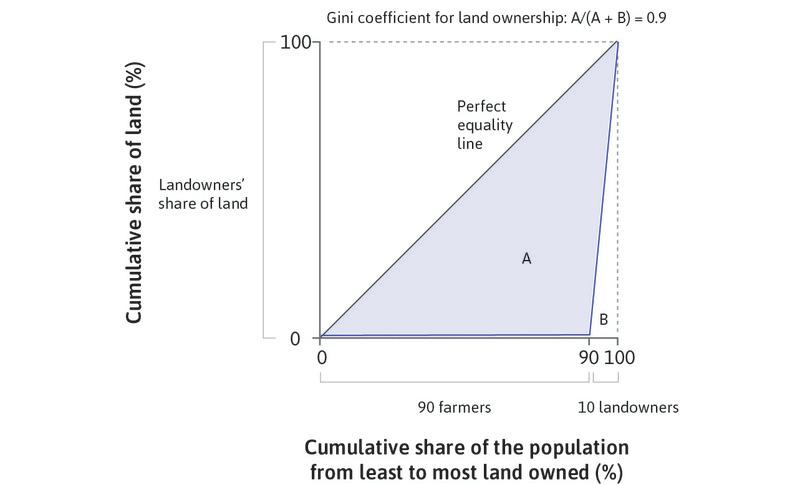
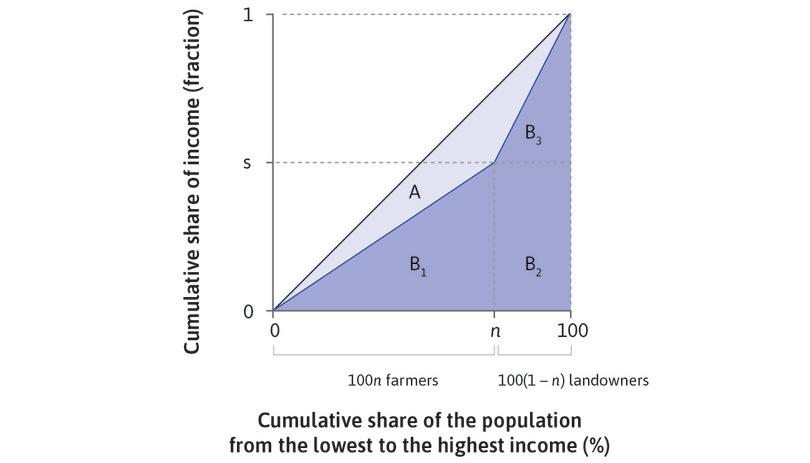
The Lorenz curve gives us a picture of the disparity of income across the whole population, but it can be useful to have a simple measure of the degree of inequality. You can see that more unequal distributions have a greater area between the Lorenz curve and the 45-degree line. The Gini coefficient (or Gini ratio) named after the Italian statistician Corrado Gini (1884–1965), is calculated as the ratio of this area to the area of the whole of the triangle under the 45-degree line.
If everyone has the same income, so that there is no income inequality, the Gini coefficient takes a value of 0. If a single individual receives all the income, the Gini coefficient takes its maximum value of 1. We can calculate the Gini for land ownership in Figure 5.14a as area A, between the Lorenz curve and the perfect equality line, as a proportion of area (A + B), the triangle under the 45-degree line:
Figure 5.14b shows the Gini coefficients for each of the Lorenz curves we have drawn so far.
| Distribution | Gini |
|---|---|
| Pirate ship Royal Rover | 0.06 |
| British Navy ship Active | 0.59 |
| British Navy ship Favourite | 0.6 |
| The village with sharecroppers and landowners | 0.9 |
Comparing Gini coefficients.
Figure 5.14b Comparing Gini coefficients.
Strictly speaking, this method of calculating the Gini gives only an approximation. The Gini is more precisely defined as a measure of the average difference in income between every pair of individuals in the population, as explained in the Einstein at the end of this section. The area method gives an accurate approximation only when the population is large.
Comparing income distributions and inequality across the world
- disposable income
- Income available after paying taxes and receiving transfers from the government.
To assess income inequality within a country, we can either look at total market income (all earnings from employment, self-employment, savings and investments), or disposable income, which better captures living standards. Disposable income is what a household can spend after paying tax and receiving transfers (such as unemployment benefit and pensions) from the government:
In Unit 1, we compared inequality in the income distributions of countries using the 90/10 ratio. Lorenz curves give us a fuller picture of how distributions differ. Figure 5.15 shows the distribution of market income in the Netherlands in 2010. The Gini coefficient is 0.47, so by this measure it has greater inequality than the Royal Rover, but less than the British Navy ships. The analysis in Figure 5.15 shows how redistributive government policies result in a more equal distribution of disposable income.
Notice that in the Netherlands, almost one-fifth of the households have a near-zero market income, but most nonetheless have enough disposable income to survive, or even live comfortably: the poorest one-fifth of the population receive about 10% of all disposable income.
There are many different ways to measure income inequality besides the Gini and the 90/10 ratio, but these two are widely used. Figure 5.16 compares the Gini coefficients for disposable and market income across a large sample of countries, ordered from left to right, from the least to the most unequal by the disposable income measure. The main reason for the substantial differences between nations in disposable income inequality is the extent to which governments can tax well off families and transfer the proceeds to the less well off.
Notice that:
- The differences between countries in disposable income inequality (the top of the lower bars) are much greater than the differences in inequality of market incomes (the top of the upper bars).
- The US and the UK are among the most unequal of the high-income economies.
- The few poor and middle-income countries for which data are available are even more unequal in disposable income than the US but …
- … (with the exception of South Africa) this is mainly the result of the limited degree of redistribution from rich to poor, rather than unusually high inequality in market income.
We study redistribution of income by governments in more detail in Unit 19 (Inequality).
Income inequality in market and disposable income across the world.
Income inequality in market and disposable income across the world.
Figure 5.16 Income inequality in market and disposable income across the world.
LIS. Cross National Data Center. Stefan Thewissen (University of Oxford) did the calculations in April 2015.
Question 5.10 Choose the correct answer(s)
Figure 5.15 shows the Lorenz curve for market income in the Netherlands in 2010.
Which of the following is true?
- If area A increases, then inequality (as measured by the Gini coefficient) rises.
- This method can be used when the population is large, as it is for a whole country such as the Netherlands.
- Countries with lower Gini coefficients have lower inequality (by this measure) hence a more equal income distribution.
- The coefficient takes the value zero when all have the same income (the Lorenz curve is on the perfect equality line).
Exercise 5.9 Comparing distributions of wealth
The table shows three alternative distributions of land ownership in a village with 100 people and 100 hectares of land. Draw the Lorenz curves for each case. For cases I and III calculate the Gini. For case II, show on the Lorenz curve diagram how the Gini coefficient can be calculated.
I 80 people own nothing 20 people own 5 hectares each II 40 people own nothing 40 people own 1 hectare each 20 people own 3 hectares each III 100 people own 1 hectare each
Einstein Inequality as differences among people
The Gini coefficient is a measure of inequality, precisely defined as:
To calculate g, you should know the incomes of every member of a population:
- Find the difference in income between every possible pair in the population.
- Take the mean of these differences.
- Divide this number by the mean income of the population, to get the relative mean difference.
- g = relative mean difference divided by two.
Examples:
There are just two individuals in the population and one has all the income. Assume their incomes are 0 and 1.
- The difference between the incomes of the pair = 1.
- This is the mean difference because there is just one pair.
- Mean income = 0.5, so the relative mean difference = 1/0.5 = 2.
- g = 2/2 = 1 (perfect inequality, as we would expect).
Two people are dividing a pie: one has 20%, and the other 80%.
- The difference is 60% (0.60).
- This is the mean difference (there are only two incomes, as before).
- Mean income is 50% or 0.50. The relative mean difference is 0.6/0.5 = 1.20.
- g = 0.60.
The Gini coefficient is a measure of how unequal their slices are. As an exercise, confirm that if the size of the smaller slice of the pie is σ, g = 1 − 2σ.
There are three people, and one has all of the income, which we assume is 1 unit.
- The differences for the three possible pairs are 1, 1, and 0.
- Mean difference = 2/3.
- Relative mean difference = (2/3)(1/3) = 2.
- g = 2/2 = 1.
Approximating the Gini using the Lorenz curve
If the population is large, we obtain a good approximation to the Gini coefficient using the areas in the Lorenz diagram: g ≈ A/(A + B).
But with a small number of people, this approximation is not accurate.
You can see this if you think about the case of ‘perfect inequality’ when one individual gets 100% of the income, for which the true Gini is 1, whatever the size of the population (we calculated it for populations of 2 and 3 above). The Lorenz curve is horizontal at zero up to the last individual, and then shoots up to 100%. Try drawing the Lorenz curves when the size of the population, N, is 2, 3, 10, and 20.
- When N = 2 , A/(A + B) = 0.5, a very poor approximation to the true value, g = 1.
- When N is large, area A is not quite as big as area A + B, but the ratio is almost 1.
There is a formula that calculates the correct Gini coefficient from the Lorenz diagram:
(Check for yourself that this works for the perfect inequality case when N = 2.)
5.13 A policy to redistribute the surplus and raise efficiency
Angela and Bruno live in the hypothetical world of an economic model. But real farmers and landowners face similar problems.
In the Indian state of West Bengal, home to more people than Germany, many farmers work as sharecroppers (bargadars in the Bengali language), renting land from landowners in exchange for a share of the crop.
The traditional contractual arrangements throughout this vast state varied little from village to village, with virtually all bargadars giving half their crop to the landowner at harvest time. This had been the norm since at least the eighteenth century.
But, like Angela, in the second half of the twentieth century many thought this was unfair, because of the extreme levels of deprivation among the bargadars. In 1973, 73% of the rural population lived in poverty, one of the highest poverty rates in India. In 1978, the newly elected Left Front government of West Bengal adopted new laws, called Operation Barga.
The new laws stated that:
- Bargadars could keep up to three-quarters of their crop.
- Bargadars were protected from eviction by landowners, provided they paid them the 25% quota.
Both provisions of Operation Barga were advocated as a way of increasing output. There are certainly reasons to predict that the size of the pie would increase, as well as the incomes of the farmers:
- Bargadars had a greater incentive to work hard and well: Keeping a larger share meant that there was a greater reward if they grew more crops.
- Bargadars had an incentive to invest in improving the land: They were confident that they would farm the same plot of land in the future, so would be rewarded for their investment.
West Bengal enjoyed a subsequent dramatic increase in farm output per unit of land, as well as farming incomes. By comparing the output of farms before and after the implementation of Operation Barga, economists concluded that both improved work motivation and investment occurred. One study suggested that Operation Barga was responsible for around 28% of the subsequent growth in agricultural productivity in the region. The empowerment of the bargadars also had positive spillover effects as local governments became more responsive to the needs of poor farmers.5
Efficiency and fairness
Operation Barga was later cited by the World Bank as an example of good policy for economic development.6
Figure 5.17 summarizes the concepts developed in this unit that we can use to judge the impact of an economic policy. Having gathered evidence to describe the resulting allocation, we ask: is it Pareto efficient, and fair? Is it better than the original allocation by these criteria?
The evidence that Operation Barga increased incomes indicates that the pie got larger, and the poorest people got a larger slice.
In principle, the increase in the size of the pie means there could be mutual gains from the reforms, with both farmers and landowners made better off.
However, the actual change in the allocation was not a Pareto improvement. The incomes of some landowners fell following the reduction in their share of the crop. Nevertheless, in increasing the income of the poorest people in West Bengal, we might judge that Operation Barga was fair. We can assume that many people in West Bengal thought so, because they continued to vote for the Left Front alliance. It stayed in power from 1977 until 2011.
We do not have detailed information for Operation Barga, but we can illustrate the effect of the land reform on the distribution of income in the hypothetical village of the previous section, with 90 sharecroppers and 10 landowners. Figure 5.18 shows the Lorenz curves. Initially, the farmers pay a rent of 50% of their crop to the landowners. Operation Barga raises the farmer’s crop share to 75%, moving the Lorenz curve towards the 45-degree line. As a result, the Gini coefficient of income is reduced from 0.4 (similar to the US) to 0.15 (well below that of the most equal of the rich economies, such as Denmark). The Einstein at the end of this section shows you how the Gini coefficient depends on the proportion of farmers and their crop share.
Einstein The Lorenz curve and the Gini coefficient in a class-divided economy with a large population
Think about a population of 100 people in which a fraction n produce the output, and the others are employers (or landlords, or other claimants on income who are not producers).
Take, as an example, the farmers and landlords in the text (in West Bengal). Each of the n × 100 farmers produces q and he or she receives a fraction, s, of this; so each of the farmers has income sq. The (1 − n) × 100 employers each receive an income of (1 − s)q.
The figure below presents the Lorenz curve and the perfect equality line similar to Figure 5.18 in the text.
The slope of the line separating area A from B1 is s/n (the fraction of total output that each farmer gets), and the slope of the line separating area A from B3 is (1 − s)/(1 − n), the fraction of total output that each landlord gets. We can approximate the Gini coefficient by the expression A/(A + B), where in the figure B = B1 + B2 + B3.
So we can express the Gini coefficient in terms of the triangles and rectangle in the figure. To see how, note that the area of the entire square is 1 while the area (A+B) under the perfect equality line is 1/2. The area A is (1/2) − B. Then we can write the Gini coefficient as
We can see from the figure that
so,
This means that the Gini coefficient in this simple case is just the fraction of the total population producing the output (the farmers) minus the fraction of the output that they receive in income.
Inequality will increase in this model economy if:
- The fraction of producers in the economy increases but the total share of output they receive remains unchanged. This would be the case if some of the landlords became farmer tenants, each receiving a fraction s of the crop they produced.
- The fraction of the crop received by the producers falls.
5.14 Conclusion
Economic interactions are governed by institutions, which specify the rules of the game. To understand the possible outcomes, we first consider what allocations are technically feasible, given the limits imposed by biology and technology. Then, if participation is voluntary, we look for economically feasible allocations: those which could provide mutual gains (a surplus), and therefore are Pareto-improving relative to the reservation positions of the parties involved.
Which feasible allocation will arise depends on the bargaining power of each party, which determines how a surplus will be shared and in turn depends on the institutions governing the interaction. We can evaluate and compare allocations using two important criteria for judging economic interactions: fairness and Pareto efficiency.
Concepts introduced in Unit 5
Before you move on, review these definitions:
5.15 References
- Banerjee, Abhijit V., Paul J. Gertler, and Maitreesh Ghatak. 2002. ‘Empowerment and Efficiency: Tenancy Reform in West Bengal’. Journal of Political Economy 110 (2): pp. 239–280.
- Clark, Andrew E., and Andrew J. Oswald. 2002. ‘A Simple Statistical Method for Measuring How Life Events Affect Happiness’. International Journal of Epidemiology 31 (6): pp. 1139–1144.
- Leeson, Peter T. 2007. ‘An–arrgh–chy: The Law and Economics of Pirate Organization’. Journal of Political Economy 115 (6): pp. 1049–94.
- Lorenz, Max O. 1905. ‘Methods of Measuring the Concentration of Wealth’. Publications of the American Statistical Association 9 (70).
- Pareto, Vilfredo. 2014. Manual of political economy: a variorum translation and critical edition. Oxford, New York, NY: Oxford University Press.
- Raychaudhuri, Ajitava. 2004. Lessons from the Land Reform Movement in West Bengal, India. Washington, DC: World Bank.
-
Peter T. Leeson. 2007. ‘An–arrgh–chy: The Law and Economics of Pirate Organization’. Journal of Political Economy 115 (6): pp. 1049–94. ↩
-
Vilfredo Pareto. (1906) 2014. Manual of Political Economy: A Variorum Translation and Critical Edition. Oxford, New York, NY: Oxford University Press. ↩
-
Andrew Clark and Andrew Oswald. 2002. ‘A Simple Statistical Method for Measuring How Life Events Affect Happiness’. International Journal of Epidemiology 31 (6): pp. 1139–1144. ↩
-
Max O. Lorenz. 1905. ‘Methods of Measuring the Concentration of Wealth’. Publications of the American Statistical Association 9 (70). ↩
-
Abhijit V. Banerjee, Paul J. Gertler, and Maitreesh Ghatak. 2002. ‘Empowerment and Efficiency: Tenancy Reform in West Bengal’. Journal of Political Economy 110 (2): pp. 239–80. ↩
-
Ajitava Raychaudhuri. 2004. Lessons from the Land Reform Movement in West Bengal, India. Washington, DC: World Bank. ↩